Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Grid self-adaptive finite element method for simulating martensite phase transformation

A martensitic phase transformation and finite element technology, applied in CAD numerical modeling, special data processing applications, design optimization/simulation, etc., can solve the rare problems of solving dynamic evolution problems, achieve fast calculation methods, reduce calculation resource effect

Active Publication Date: 2020-12-25
SHANGHAI UNIV
View PDF5 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

The current mesh adaptive finite element method is mostly used to solve static problems, and it is relatively rare to solve dynamic evolution problems

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Grid self-adaptive finite element method for simulating martensite phase transformation
  • Grid self-adaptive finite element method for simulating martensite phase transformation
  • Grid self-adaptive finite element method for simulating martensite phase transformation

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

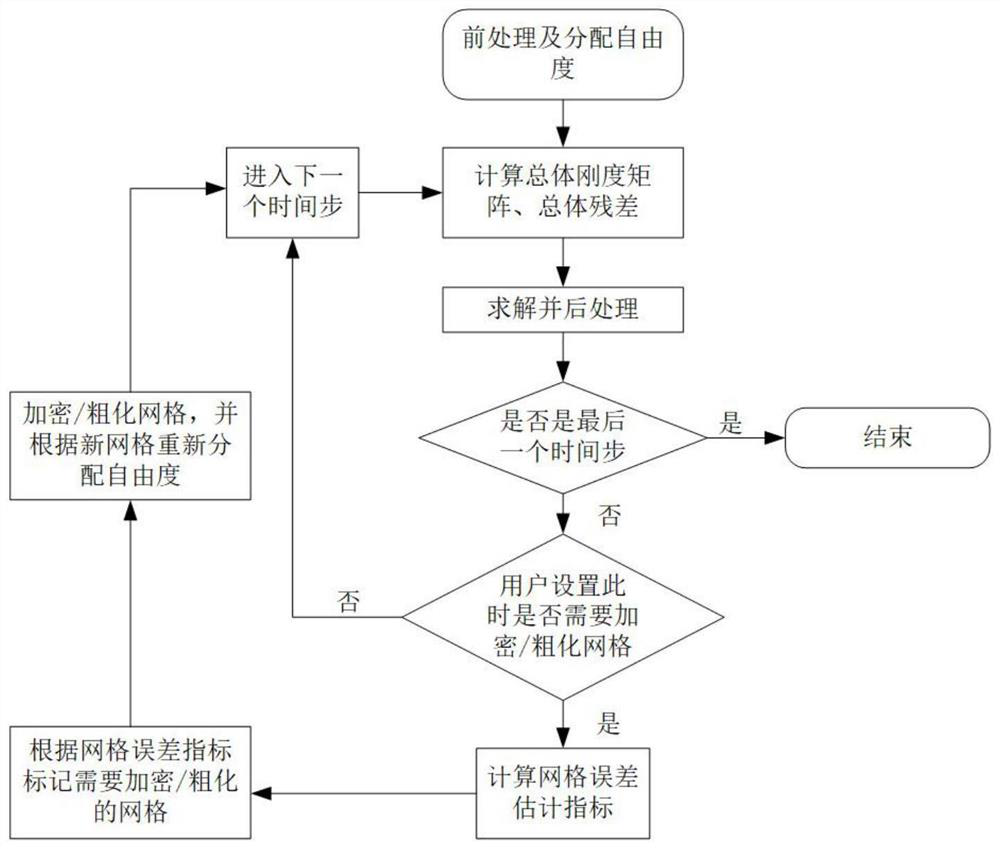
[0047] In this embodiment, a mesh adaptive finite element method for simulating martensitic transformation includes the following steps:

[0048] (1) Establish the phase field model of martensitic phase transformation, and establish the corresponding finite element model at the same time;

[0049] (2) Perform finite element pre-processing;

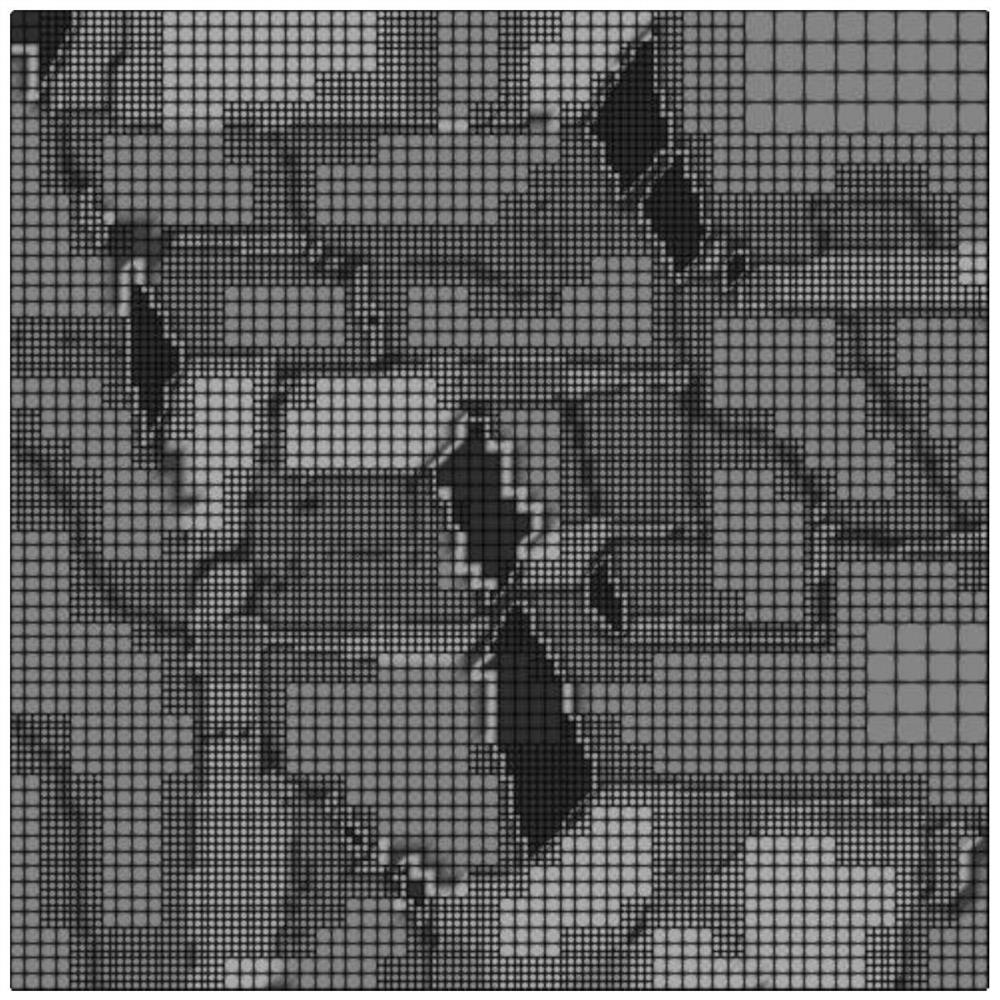
[0050] (3) Write the corresponding grid adaptive finite element program code, call the corresponding finite element program code and the open source finite element program deal.II, select the error estimation criterion to calculate the error estimate of each grid unit, and use the error estimate Determine whether the grid needs to be refined / coarsened by size, realize dynamic grid division in simulation calculations, track the interface of martensitic variants and refine the grid at the interface, coarsen the grid in the non-interface area, and reduce the overall grid Quantity, thereby reducing the total number of degrees of freedom of th...

Embodiment 2

[0054] This embodiment is basically the same as Embodiment 1, especially in that:

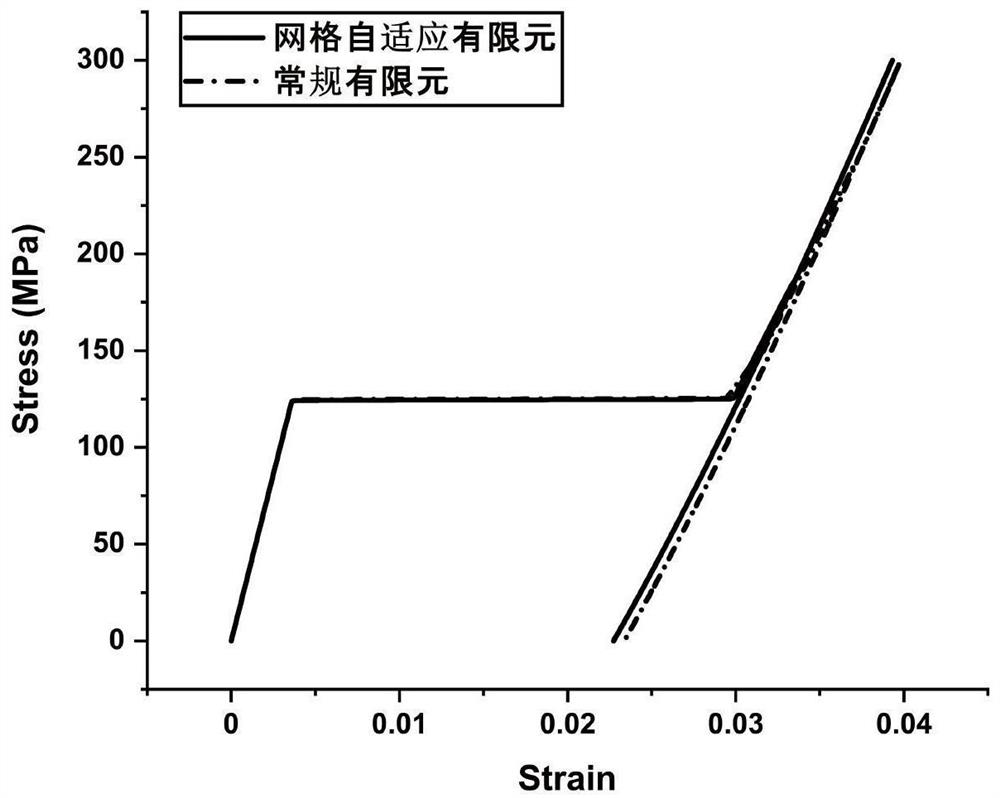
[0055] In this embodiment, taking the Ti2448 alloy as an example, the temperature-induced martensitic transformation at a temperature of 100K is calculated and simulated. In the step (1), the time-dependent Ginzburg Landau equation (Time Dependent Ginzburg Landau equation, TDGL equation) is used in the phase field model to describe the evolution process of the martensitic phase transformation, and the mechanical balance equation also needs to be satisfied :

[0056]

[0057] σ ij，j +f=0,

[0058] where η p The field variable of the phase field model also becomes an order parameter, representing the pth martensite variant; L is the kinetic coefficient; F is the total free energy; δF / δη p is the variational derivative; f is the body force; σ ij is the stress tensor, obtained through the displacement field and the constitutive relation:

[0059] σ=C(η p ): ε el (u)=C(η p ): (ε tot (u)...

Embodiment 3

[0079] This embodiment is basically the same as the previous embodiment, and the special features are:

[0080] In this embodiment, taking Ti2448 alloy as an example, the stress-induced martensitic transformation at a temperature of 200K is calculated and simulated. see figure 1 , a grid-adaptive finite element method for simulating martensitic phase transformation, including the following steps:

[0081] (1) Establish the phase field model and finite element model of martensitic transformation:

[0082] The phase field model uses the time-dependent Ginzburg Landau equation (Time dependent Ginzburg Landauequation, TDGL) to describe the evolution process of the martensitic phase transformation, and also needs to satisfy the mechanical balance equation:

[0083]

[0084] σ ij，j +f=0,

[0085] where η p The field variable of the phase field model also becomes an order parameter, representing the pth martensite variant; L is the kinetic coefficient; F is the total free ene...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a grid self-adaptive finite element method for simulating martensite phase transformation, and is applied to the field of industrial production simulation design and scientificresearch of shape memory alloys. A martensite phase transformation phase field finite element model is established, proper grid unit posteriori error estimation is set, grid units needing to be encrypted / coarsened are marked, and the encryption / coarsening step is executed, so that the effects of effectively tracking a martensite variant interface, encrypting grids at the interface and coarseningthe grids at a non-interface are achieved; under the condition of keeping the calculation precision, the total number of degrees of freedom of a solution required by phase field simulation calculationis reduced, the calculation efficiency is effectively improved, and the simulation research process is promoted. The method provided by the invention can effectively and dynamically track the dynamically changed martensite variant interface, requires fewer computing resources and computing time, and can effectively improve the computing efficiency and promote the simulation research process.

Description

technical field [0001] The invention relates to a finite element method numerical calculation and simulation of a phase field model of martensitic phase transformation, which is applied to the technical field of numerical simulation of shape memory alloys. Background technique [0002] In the industrial production and scientific research of shape memory alloy materials, the martensitic transformation behavior of shape memory alloys needs to be studied in detail. The phase field method is a numerical simulation method for simulating the microstructure evolution of materials at the mesoscopic scale. In the past few decades, the phase field method has become a powerful tool for simulating the microstructure evolution of materials at the mesoscopic scale, and has been successfully applied to simulate the microstructure evolution of materials during the martensitic phase transformation process. The governing equations of the martensitic phase transformation phase field model are...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G06F30/23G06F111/10
CPCG06F30/23G06F2111/10
Inventor 麦嘉伟张统一徐涛孙升朱玉泉
Owner SHANGHAI UNIV
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com