Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

An Optimal Order Reduction Method for Higher-Order Systems Based on Nonlinear Programming

A nonlinear programming and linear system technology, applied in general control systems, control/regulation systems, instruments, etc., can solve problems such as limiting the scope of application, and achieve the effect of reducing design difficulty and reducing order effects

Inactive Publication Date: 2017-09-12
BEIHANG UNIV
View PDF4 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

Among them, the first two methods are based on the transient response or steady-state response of the system, which greatly limits its application range.
The balance method of singular value decomposition is truncated and degraded according to the singular value of the original system, which can ensure the stability of the system after order reduction, but it needs to solve two high-order Lyapunov equations during the implementation process

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • An Optimal Order Reduction Method for Higher-Order Systems Based on Nonlinear Programming
  • An Optimal Order Reduction Method for Higher-Order Systems Based on Nonlinear Programming
  • An Optimal Order Reduction Method for Higher-Order Systems Based on Nonlinear Programming

Examples

Experimental program
Comparison scheme
Effect test

Embodiment Construction

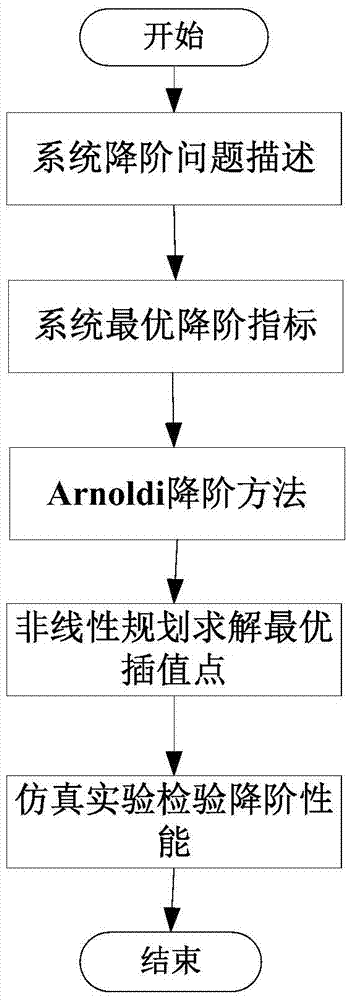
[0031] The present invention will be further described in detail below in conjunction with the technical scheme and accompanying drawings. See figure 1 , the present invention is an optimal reduction method for high-order systems based on nonlinear programming. The specific steps of the method are as follows:

[0032] Step 1: System Reduction Problem Description

[0033] Consider the following one-input-single-output linear time-invariant system:

[0034]

[0035] in for control input. The transfer function corresponding to system (1) is

[0036] G(s)=C(sI n -A) -1 B (2)

[0037] Among them, I n is an n-order identity matrix. Suppose the system model after order reduction is Σ m ,

[0038]

[0039] The corresponding transfer function is:

[0040] G m (s)=C m (sI m -A m ) -1 B m(4)

[0041] Here I m is the identity matrix of order m. For system Σ m ,m≤n, control input The purpose of order reduction is to make Σ and Σ m Under the same impulsive ...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention provides a nonlinear programming based optimal reduction method of a high-order system. The method comprises six major steps of 1, describing the reduction problem; 2, determining the optimal reduction indexes of the system; 3, graining the Arnoldi reduction method; 4, solving an optimal interpolation point by nonlinear programming; 5, inspecting the reduction performance by simulation experiment; 6, finishing the design; the first step is to determine the high-order linear system reduction purchase and perform mathematical description; the second step is to determine error norm index of the system reduction so as to prepare for the proposing of the optimal reduction method; the third step is to gain the Arnoldi reduction method based on the krylov subspace; the fourth step is to propose the optimal interpolation point solution method based on the nonlinear programming to obtain the optimal reduction model; the fifth step is to perform simulation experiment for the designed system optimal reduction method; after the steps above are done, the design is finished. The method is used for processing the high-order linear system, and the control rule design is simplified.

Description

technical field [0001] The present invention relates to an optimal order reduction method for a high-order system based on nonlinear programming, which is an optimal order reduction method for a linear time-invariant system. The model reduction method with the smallest frequency domain response error of the front and rear systems is used to deal with high-order linear systems and simplify the design of control laws, and belongs to the field of automatic control technology. Background technique [0002] With the rapid development of modern engineering technology, complex high-level systems such as flight control systems, power systems, and VLSIs have emerged. The number of differential equations describing these linear systems is large, which brings great challenges to the numerical simulation and control design of the system. Effective model reduction for these high-order systems can reduce the difficulty of system analysis, reduce computational load, and facilitate simulat...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Patents(China)
IPC IPC(8): G05B13/04
Inventor 刘金琨杨柳
Owner BEIHANG UNIV
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com