Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

All-pass time reversal ultra-wideband wireless communication method and system

A time-reversal and wireless channel technology, which is applied in baseband system components, transmission systems, transmitter/receiver shaping networks, etc., can solve the complex channel estimation algorithm waveform generator optimization parameter design time synchronization, calculation Increased quantity, inconvenient project realization and other issues

Active Publication Date: 2011-04-27
四川电子科技大学教育发展基金会
View PDF0 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

The theoretical results of amplitude equalization for the entire channel show that this technology is superior to PTR and pure forced zero equalization technology in suppressing multipath, but this system requires complex channel estimation algorithms and complex optimization of waveform generators Parameter design and strict time synchronization are not easy to implement
[0007] Equalization technology is an effective technology to suppress coherent multipath and incoherent multipath, but the above-mentioned minimum root mean square error and forced return to zero equalization technology are difficult in structure and engineering implementation, the equalization algorithm is complex, and the amount of calculation also varies with the propagation path. It is difficult to be widely used in high-speed wireless communication systems due to the sharp increase in the number of

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • All-pass time reversal ultra-wideband wireless communication method and system
  • All-pass time reversal ultra-wideband wireless communication method and system
  • All-pass time reversal ultra-wideband wireless communication method and system

Examples

Experimental program
Comparison scheme
Effect test

specific Embodiment approach 1

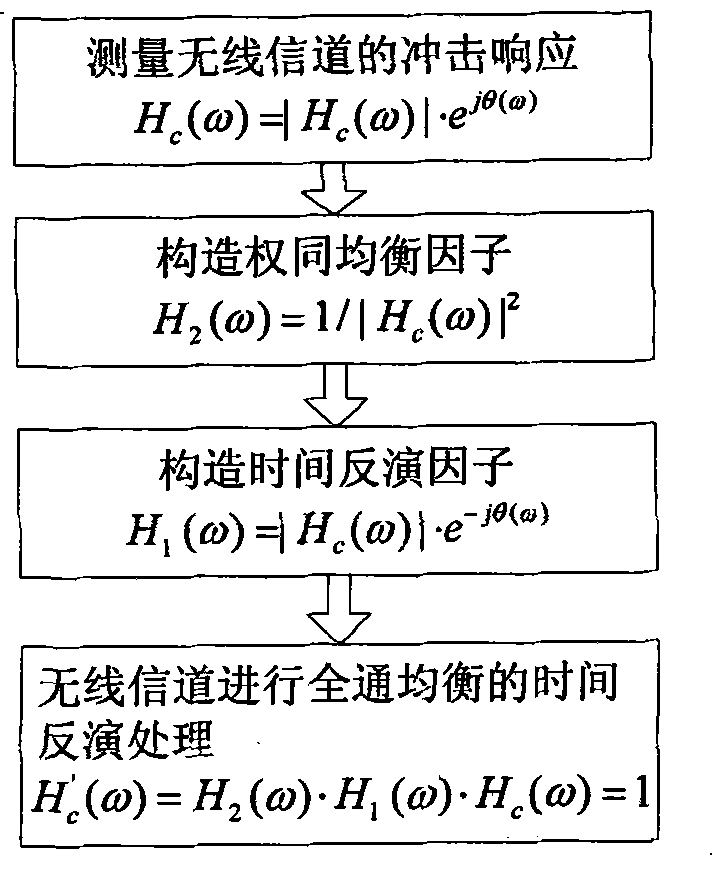
[0045] In step 4, use the all-pass equalization factor H of the wireless channel obtained in step 2 2 (ω)=1 / |H c (ω)| 2 and the time reversal factor H of the wireless channel obtained in step 3 1 (ω)=|H c (ω)|·e -jθ(ω) , the time-reversal processing of all-pass equalization for the wireless channel is performed at the transmitter, and the specific method is as follows:

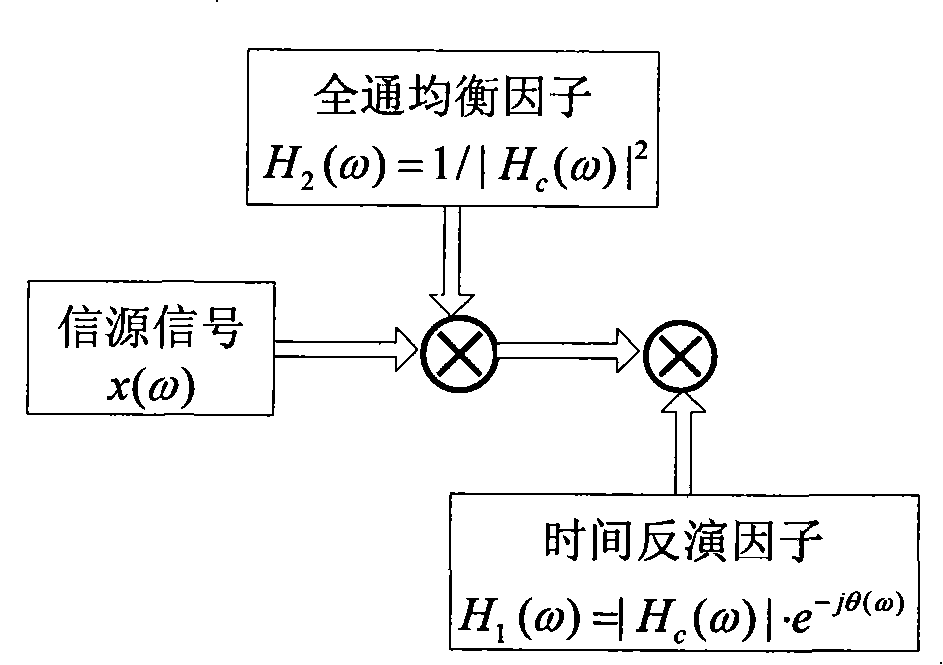
[0046] Such as figure 2 As shown, assuming that the source signal is x(ω), the time inversion process of all-pass equalization is performed on the source signal x(ω) in turn, that is, the source signal x(ω) and the all-pass equalization factor H 2 (ω)=1 / |H c (ω)| 2 with time reversal factor H 1 (ω)=|H c (ω)|·e -jθ(ω) Convolution operations are performed sequentially, and the obtained signal x(ω)·1 / |H c (ω)| 2 ·|H c (ω)|·e -jθ(ω) Signal x(ω)·1 / |H is obtained after wireless channel transmission c (ω)| 2 ·|H c (ω)|·e -jθ(ω) ·|H c (ω)|·e jθ(ω) =x(ω).

[0047] In the above scheme, the time inv...

specific Embodiment approach 2

[0048] In step 4, use the all-pass equalization factor H of the wireless channel obtained in step 2 2 (ω)=1 / |H c (ω)| 2 and the time reversal factor H of the wireless channel obtained in step 3 1 (ω)=|H c (ω)|·e -jθ(ω) , the time-reversal processing of all-pass equalization for the wireless channel is carried out at the receiving end, and the specific method is as follows:

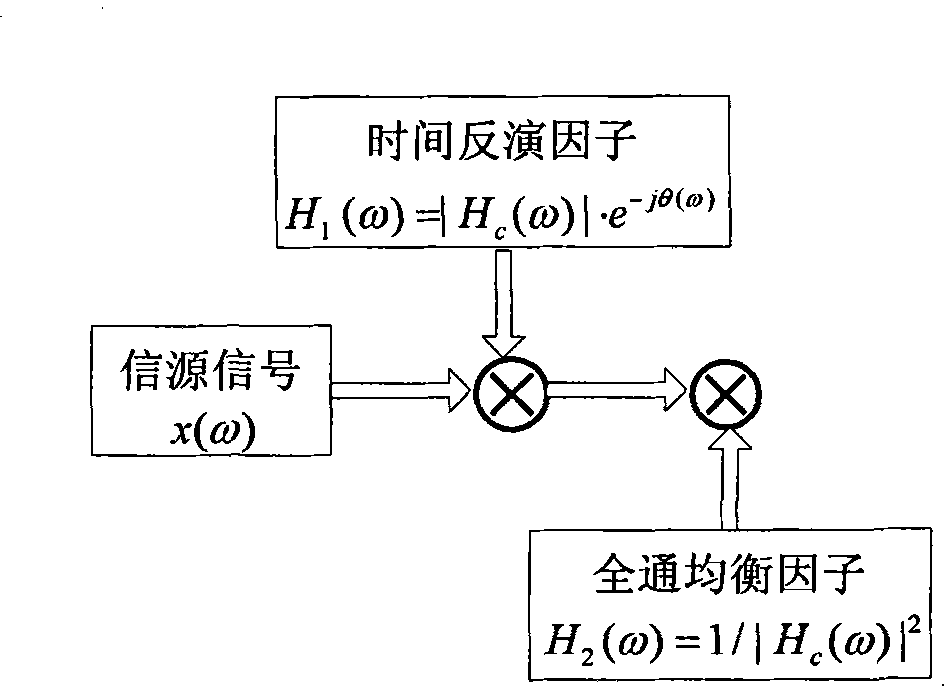
[0049] Such as Figure 4 As shown, let the source signal be x(ω), and the signal received by the antenna at the receiving end is y(ω)=x(ω)|H c (ω)|·e jθ(ω) , the signal y(ω) and the all-pass equalization factor H 2 (ω)=1 / |H c (ω)| 2 with time reversal factor H 1 (ω)=|H c (ω)|·e -jθ(ω) Convolution operations are performed sequentially to obtain the signal y(ω)·1 / |H c (ω)| 2 ·|H c (ω)|·e -jθ(ω) =x(ω).

[0050] In the above scheme, the time inversion process of all-pass equalization is performed on the signal received by the receiving antenna as y(ω), or the signal received by the receiving a...

specific Embodiment approach 3

[0051] In step 4, use the all-pass equalization factor H of the wireless channel obtained in step 2 2 (ω)=1 / |H c (ω)| 2 and the time reversal factor H of the wireless channel obtained in step 3 1 (ω)=|H c (ω)|·e -jθ(ω) , the time-reversal processing of all-pass equalization for the wireless channel is carried out at the transmitting end and the receiving end respectively, and the specific method is as follows:

[0052] Such as Figure 6 As shown, assuming that the source signal is x(ω), the all-pass equalization process is performed on the source signal x(ω), that is, the source signal x(ω) and the all-pass equalization factor H 2 (ω)=1 / |H c (ω)| 2 Carry out convolution operation, the obtained signal x(ω)·1 / |H c (ω)| 2 Signal x(ω)·1 / |H is obtained after wireless channel transmission c (ω)| 2 ·|H c (ω)|·e jθ(ω) ; Then the signal x(ω)·1 / |H c (ω)| 2 ·|H c (ω)|·e jθ(ω) and time reversal factor H 1 (ω)=|H c (ω)|·e -jθ(ω) Carry out convolution operation to get th...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention provides an all-pass balanced time-reversal ultra-wide band (UWB) wireless communication method and system used for restraining the coherent multi-path effect in the UWB wireless communication system and improving the transmission performance of the communication system which belongs to the wireless communication technical field. The shock response Hc(omega)=|Hc(omega)| *e<jtheta(omega)> of a wireless channel is measured firstly; and then a all-pass balanced factor H2(omega)=1 / |Hc(omega)|<2> and a time-reversal factor H1(omega)=|Hc(omega)|*e<-jtheta(omega)> of the wireless channel are constructed respectively; finally, the all-pass balanced factor H2 (omega) and the time-reversal factor H1 (omega) are used for the all-pass balanced time-reversal processing to the wireless channel, so that a transmission function of the wireless channel after the all-pass balanced time-reversal processing is H'c(omega)=H2(omega)*H1(omega)*Hc(omega)=1. The invention adopts the all-pass balanced factor H2(omega)=1 / |Hc(omega)|<2> to process all-pass balancing to the time-reversal wireless channel, enables the wireless channel processed by the all-pass balanced time-reversal processing to be equivalent to an all-pass channel and eliminates the coherent multi-path effect (namely frequency selective fading) in the ultra-wide band wireless communication channel. The all-pass balanced time-reversal ultra-wide band wireless communication method has the advantages of simple operation, lower computation burden and being applicable to the wireless communication systems with different communication schemes.

Description

technical field [0001] The invention belongs to the technical field of wireless communication, and relates to a time reversal (TR, Time Reversal) wireless communication technology. Background technique [0002] TR (TR, Time Reversal) is a new type of multipath fading suppression technology developed in recent years. Different from traditional multipath fading technology, TR has unique time-space focusing function and natural environmental adaptability. TR can automatically adapt to various complex wireless transmission environments, and does not require complex multipath signal combination processing and adaptive algorithms, which greatly simplifies receiver design; more importantly, TR can fully suppress multipath fading Using multipath energy to improve signal-to-noise ratio, compress pulse extension, reduce inter-symbol interference, and improve system communication capacity. A large number of theoretical and experimental studies have shown that in an environment rich i...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Patents(China)
IPC IPC(8): H04L25/03H04B1/719
Inventor 赵德双王秉中张益
Owner 四川电子科技大学教育发展基金会
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com