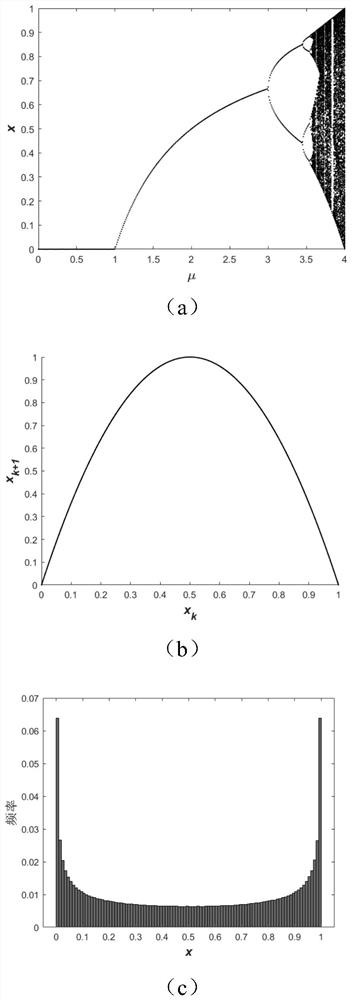
Coupling chaotic system and application thereof
A technology of chaotic system and system state, applied in the field of information security, can solve the problems of small parameter range, hidden safety hazards, simple structure, etc.
- Summary
- Abstract
- Description
- Claims
- Application Information
AI Technical Summary
Problems solved by technology
Method used
Image
Examples
Embodiment 1
[0068] A coupled chaotic system, the system state variable X in the k+1th iteration of the coupled chaotic system k+1 for:
[0069] x k+1 =Q k ×(f(X k )+Δ k ) mod 1;
[0070] Among them, f() is a function column composed of one or more mapping functions of discrete chaotic systems; Q k =diag(V k ); X and V are n-dimensional vectors, which respectively represent the system state variables and system parameters of the coupled chaotic system, and the subscript represents the number of iterations; Δ k is the n-dimensional coupling item constructed based on the system state variable in the kth iteration; "mod 1" means taking a decimal operation;
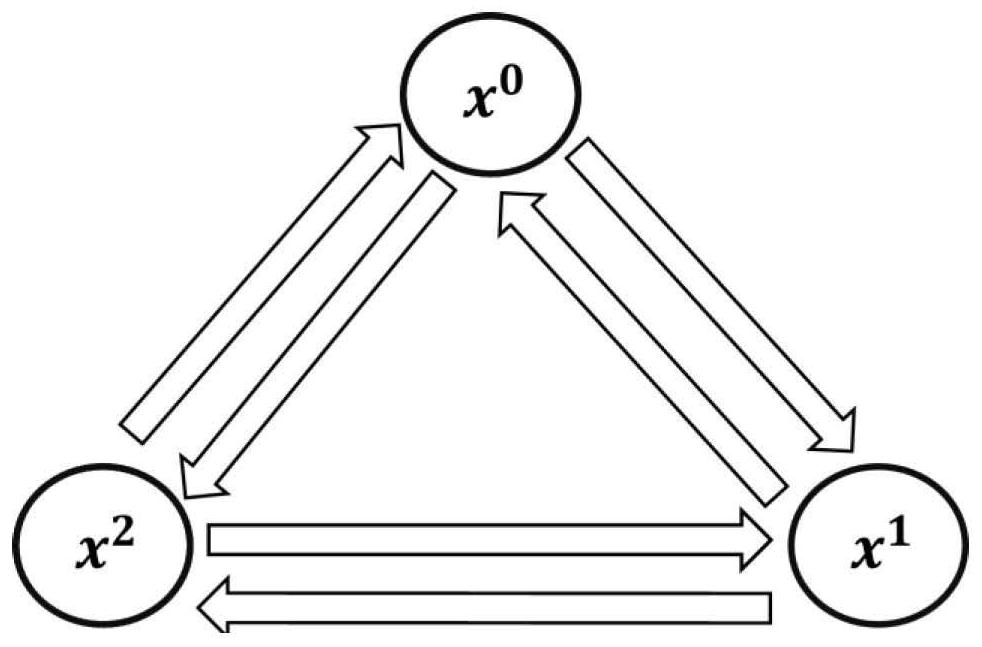
[0071] In this embodiment, n=3, correspondingly, the system state variable can be expressed as X=(x 0 ,x 1 ,x 2 ), in different iterations, it is distinguished by subscript; f() represents logistic chaotic system;
[0072] Optionally, in this embodiment, the n-dimensional coupling term Δ constructed based on the system state va...
Embodiment 2
[0083] A coupled chaotic system, the system state variable X in the k+1th iteration of the coupled chaotic system k+1 for:
[0084] x k+1 =Q k ×(f(X k )+Δ k ) mod 1;
[0085] Among them, f() is a function column composed of one or more mapping functions of discrete chaotic systems; Q k =diag(V k ); X and V are n-dimensional vectors, which respectively represent the system state variables and system parameters of the coupled chaotic system, and the subscript represents the number of iterations; Δ k is the n-dimensional coupling item constructed based on the system state variable in the kth iteration; "mod 1" means taking a decimal operation;
[0086] In this embodiment, n=3, correspondingly, the system state variable can be expressed as X=(x 0 , x 1 , x 2 ), in different iterations, it is distinguished by subscript; f() represents logistic chaotic system;
[0087] In the coupled chaotic system provided by this embodiment, during the iterative process, the coupling te...
Embodiment 3
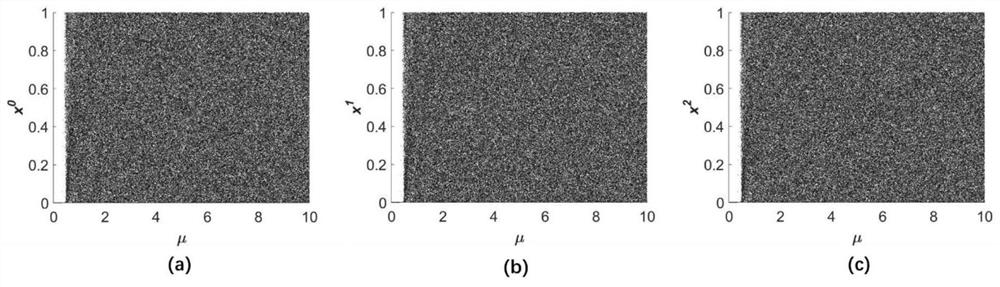
[0105] A coupled chaotic system, this embodiment is similar to the above-mentioned embodiment 2, the difference is that in this embodiment, and the operator σ 3 The way of disturbing the arrangement order of elements in the 2n-dimensional vector is correspondingly changed to ensure that the coupling structure of the coupled chaotic system is irregular.
PUM
 Login to View More
Login to View More Abstract
Description
Claims
Application Information
 Login to View More
Login to View More - R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com