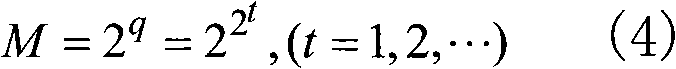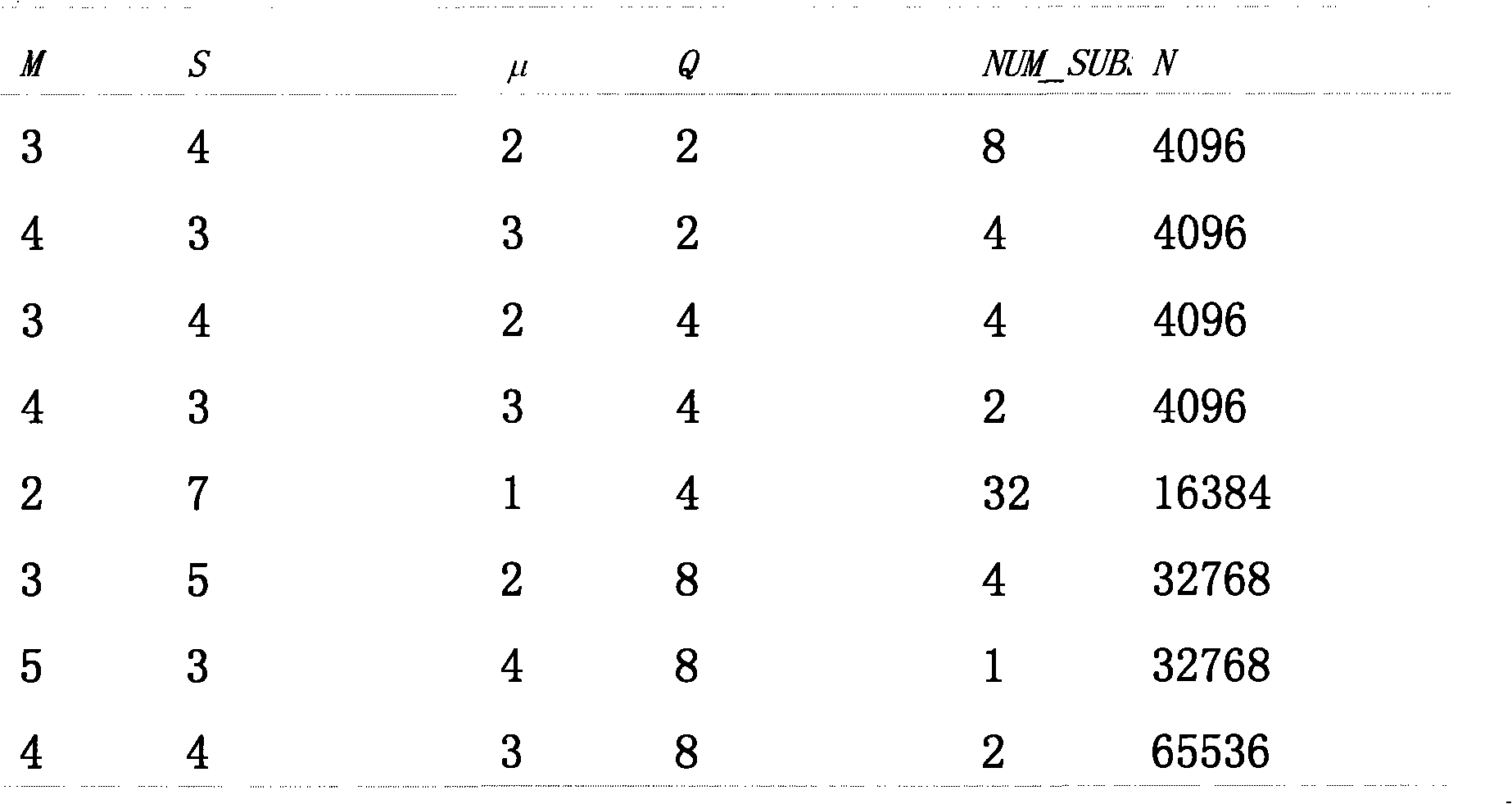Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Decomposable LDPC code coding method based on euclidean geometry

A technology of LDPC codes and coding methods, which is applied in the direction of preventing/detecting errors through diversity reception, digital transmission systems, electrical components, etc., can solve problems such as the construction of LDPC codes that cannot be solved, and achieve the effect of reducing complexity

Inactive Publication Date: 2008-09-03
ZHEJIANG UNIV
View PDF0 Cites 8 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0020] The method proposed by Shu Lin only solves the construction method of the LDPC code under the binary system, but cannot solve the construction of the LDPC code under the multi-ary modulation

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Decomposable LDPC code coding method based on euclidean geometry
  • Decomposable LDPC code coding method based on euclidean geometry
  • Decomposable LDPC code coding method based on euclidean geometry

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

[0031] 1) Select a suitable Euclidean space:

[0032] It is assumed that the code word transmission adopts the M-ary modulation mode, so there are M constellation points in the signal constellation diagram. Each constellation point is represented by q bits, and the multi-level coding is composed of q component codes. Select the appropriate Euclidean space EG(m, 2 according to the code length n of the LDPC code to be constructed. s ), making the number of all points in this space 2 ms =n.

[0033] 2) Select the appropriate q value according to the modulation factor:

[0034] Consider EG(m,2 s ) space in each μ-dimensional subspace, as we can see from the front, each subspace contains 2 μs points, and there will be 2 (m-μ)s -1 μ-dimensional subspace and it form a set of μ-dimensional parallel subspaces. Put the 2 in the whole space ms We can divide the points into q groups according to the modulation method, and the grouping set uses C q to represent, so C q The number...

Embodiment 2

[0058] The previous implementations were based on GF(2 s ) of the extended domain, so there will be certain restrictions on the selection of the length of the codeword and the selection of the modulation coefficient, then it can be constructed based on the definition in GF(p s ) on the Euclidean space EG(m,p s ), so that the number of points in the space is p ms , according to the modulation parameter q, choose p appropriately so that the number of points in each group is p ms / q, generally p and q satisfy the following relationship:

[0059] p t =q (t=1,2...) (7)

[0060] Based on the definition in GF(p s ) on the Euclidean space EG(m,p s ) method of constructing LDPC codes is based on the definition in GF(2 s EG(m,2 ) on s )it's the same. In this way, this method constructs a large class of decomposable LDPC codes. Table 2 lists the selection of p and the selection of other parameters under different modulation modes.

[0061] Table 2: Parameter selection under di...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention relates to a code method of a channel correcting LDPC code. The existing method only solves the construction method of the LDPC code below the binary system, but not solves the DDPC construction under the multi-system modulation. The method divides the initial LDPC code into q LDPC subcode to realize the decoding result of the LDPC short code by the q-th binary iteration decoding algorithm by means of multiple decoding. The final decoding result of the initial LDPC code is obtained by a simple combination based on the modulation and interweaving mode. The obtained LDPC code doesn't appear four rings in the Tanner graph. The coding mode is multi-coding, thus the decoding is multi-decoding. The decoding in each stage adopts the decoding algorithm below the binary system so as to greatly reduce the complexity of which directly decodes the multi-system code word.

Description

technical field [0001] The invention belongs to the communication field, and relates to an encoding method of a channel error correction LDPC (low density parity code, low density parity code) code, in particular to constructing a class of decomposable LDPC codes in a Euclidean geometric space with an algebraic method, A method for performing multi-level encoding on the decomposed sub-codes, so as to facilitate transmission and decoding of each sub-code as a component code of the multi-level encoding. Background technique [0002] The LDPC code was proposed by Gallager in 1962, so it is also called the Gallager code, which is another codeword near the Shannon limit (or channel capacity) besides the Turbo code. Although Gallager proved that LDPC codes are asymptotically good codes, due to the limited computing power at that time, LDPC codes were once considered to be an unrealizable channel coding method, and they did not receive people's attention for a long time. [0003] ...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): H04L1/00H04L1/06
Inventor 谢磊刘亚汪莹陈惠芳高明
Owner ZHEJIANG UNIV
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com