Buck-type converter cascade system stability analysis method based on gyrator model
A technology of cascade system and analysis method, applied in control/regulation system, high-efficiency power electronic conversion, output power conversion device, etc. It solves problems such as difficulty in calculating impedance of the connected system, and achieves the effects of intuitive and effective stability improvement, simplified calculation process, and intuitive and effective thinking.
- Summary
- Abstract
- Description
- Claims
- Application Information
AI Technical Summary
Problems solved by technology
Method used
Image
Examples
Embodiment 1
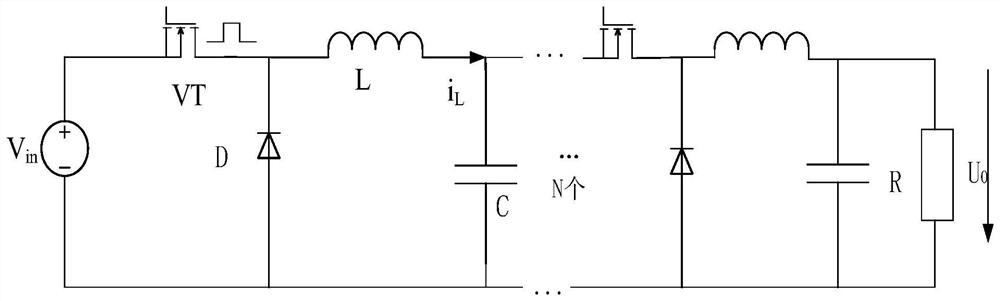
[0043] Taking the two-stage cascade system as an example, the Buck converter topology structure is given first, such as figure 1 shown, and derived its mathematical model.
[0044] figure 1 It is the main circuit topology diagram of Buck converter. where V in is the input DC voltage, VT is the controllable power device, V o is the output voltage, D is the freewheeling diode, L is the filter inductance, C is the filter capacitor, R is the load resistance, i L is the inductor current. By analyzing the turn-on and turn-off conditions of the power devices in the Buck converter, and selecting the inductor current i L and the output voltage V o As the state variable of the system, it can be deduced that the differential equations of the Buck converter when the power device is turned on and off are:
[0045]
[0046]
[0047] where V in is the input DC voltage, L is the filter inductor, C is the filter capacitor, and R is the load resistance.
[0048] It can be deduced...
PUM
 Login to View More
Login to View More Abstract
Description
Claims
Application Information
 Login to View More
Login to View More - R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com