Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Method for constructing quasi-cyclic low-density check code based on Euclidean geometry (EG)

A technology of a low-density check code and a construction method, which is applied in the field of construction of a quasi-cyclic low-density check code, can solve many problems, such as affecting decoding performance, and difficulty in further reducing the complexity of hardware implementation.

Inactive Publication Date: 2012-04-11
桂林市思奇通信设备有限公司
View PDF3 Cites 7 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0009] However, although these structured LDPC construction methods avoid the influence of 4 rings on the performance of iterative decoding, the error correction performance is better, but because there are still a lot of 6 rings in it, the check matrix density is high, which affects the decoding performance. , the complexity of its hardware implementation is difficult to further reduce

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Method for constructing quasi-cyclic low-density check code based on Euclidean geometry (EG)
  • Method for constructing quasi-cyclic low-density check code based on Euclidean geometry (EG)
  • Method for constructing quasi-cyclic low-density check code based on Euclidean geometry (EG)

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

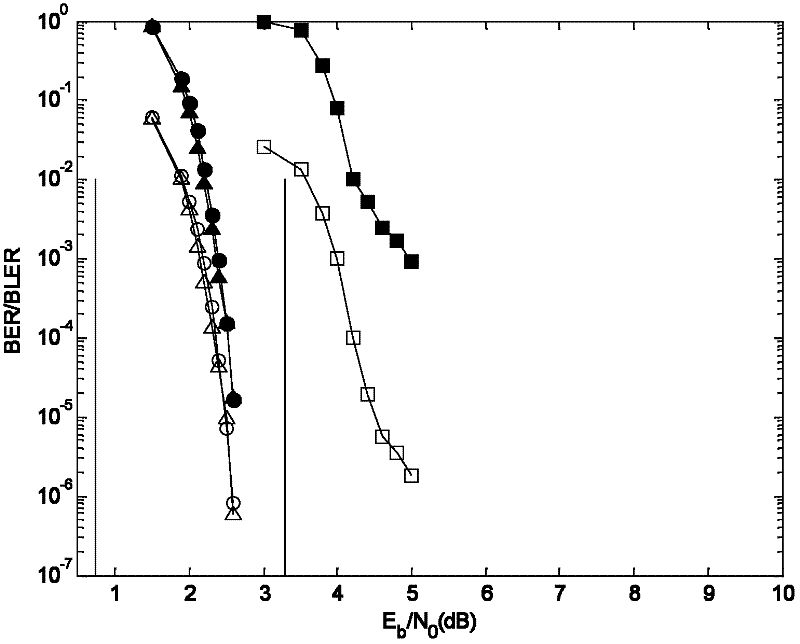
[0037] In this example, the steps of the quasi-cyclic LDPC encoding method based on Euclidean geometry are as follows:

[0038] I, select Euclidean geometry EG (4,2 2 ), construct 21 sparse matrices, denoted as H 1 , H 2 ,…H 21 ,

[0039] EG(4,2 2 ) in all straight lines are divided into K=(2 3×2 -1) / (2 2 -1) = 21 cyclic classes. For 1≤k≤21, construct a sparse matrix H k , matrix H k Each column of is the associated vector of the line in the kth cycle class, H k is a 4×1 matrix array composed of n×n cyclic permutation matrices, randomly select a straight line in the kth cyclic class, and use the associated vector of the straight line as the matrix H k The first column of H k The rest of the columns are obtained by the piecewise cyclic shift of the previous column. Based on the correlation vector of straight lines in each cyclic class of Euclidean geometry, a sparse matrix H consisting of cyclic permutation matrices is constructed k , and 21 sparse matrices are obt...

Embodiment 2

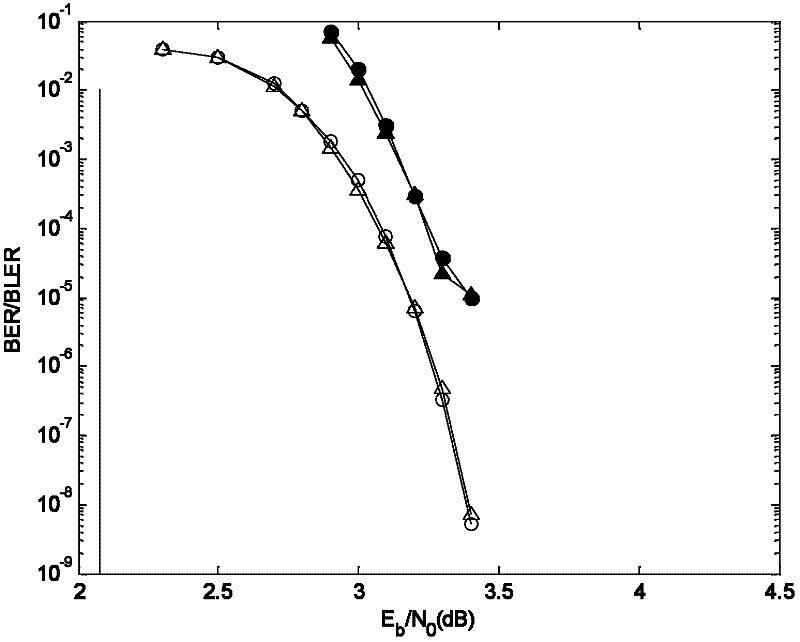
[0049] I, select Euclidean geometry EG (4,2 2 ), construct 21 sparse matrices, denoted as H 1 , H 2 ,…H 21 ,

[0050] EG(4,2 2 ) in all straight lines are divided into K=21 cycle classes. For 1≤k≤21, construct a sparse matrix H k , matrix H k Each column of is the associated vector of the line in the kth cycle class, H k is a 4×1 matrix array composed of n×n cyclic permutation matrices, randomly select a straight line in the kth cyclic class, and use the associated vector of the straight line as the matrix H k The first column of H k The rest of the columns are obtained by the piecewise cyclic shift of the previous column. Based on the correlation vector of straight lines in each cyclic class of Euclidean geometry, a sparse matrix H consisting of cyclic permutation matrices is constructed k , and 21 sparse matrices are obtained.

[0051] II, 21 sparse matrices obtained in step I are used as sub-matrixes to construct the following matrix:

[0052] H ...

Embodiment 3

[0060] I, choose Euclidean geometry EG (5,2 2 ), construct 85 sparse matrices, denoted as H 1 , H 2 ,…H 85 ,

[0061] Will EG(5,2 2 ) in all straight lines are divided into K=(2 4×2 -1) / (2 2 -1) = 85 cyclic classes. For 1≤k≤85, construct a sparse matrix H k , matrix H k Each column of is the associated vector of the line in the kth cycle class, H k is a 4×1 matrix array composed of n×n cyclic permutation matrices, randomly select a straight line in the kth cyclic class, and use the associated vector of the straight line as the matrix H k The first column of H k The rest of the columns are obtained by the piecewise cyclic shift of the previous column. Based on the correlation vector of straight lines in each cyclic class of Euclidean geometry, a sparse matrix H consisting of cyclic permutation matrices is constructed k , got 85 sparse matrices.

[0062] II, 85 sparse matrices that step I gained are used as sub-matrixes to construct the following matrix:

[0063] ...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a method for constructing quasi-cyclic low-density check codes based on Euclidean geometry (EG). The method comprises the following steps of: I, selecting Euclidean geometry EG (m, ps) to construct K sparse matrixes; II, constructing a matrix H by taking K matrixes as sub-matrixes; III, constructing a sub-array H (gamma, rho) of an array H for given code parameters: rho is more than or equal to row weight 1 and less than or equal to K, and gamma is more than or equal to line weight 1 and less than or equal to ps; IV, performing random arrangement to obtain a sparse matrix, wherein T is less than an optional threshold (gamma !) (pho-1) and more than or equal to 104, performing random arrangement for T times to obtain T sparse matrixes, and calculating the quantity of loops 6 in a corresponding Tenna figure; and V, selecting a matrix of which the quantity of loops 6 is smallest for serving as a check matrix of LDPC (Low Density Parity Check) codes to finish the construction of codes. The obtained LDPC codes are (2550, 1553), (5100, 4103), (15345, 11286). In the method, QC-LDPC (Quasi-Cyclic-Low Density Parity Check) codes not containing loops 4 are constructed by using the structural characteristics of EG, and QC-LDPC codes with least loops 6, superior loop distribution and excellent error correcting performance can be selected; and the method is suitable for China digital sound broadcasting.

Description

(1) Technical field [0001] The invention relates to the technical field of channel coding in the communication industry, in particular to a construction method of a Quasi Cyclic-Low Density Parity Check (QC-LDPC) based on Euclidean geometry. (2) Background technology [0002] Communication systems are designed to efficiently and reliably transfer information from sources to destinations. Noise on a disruptive communication channel can interfere with transmitted information, possibly reducing the reliability of the communication. Therefore, a key issue in communication system design is how to effectively and reliably transmit information in the case of random noise interference. The core is to provide immunity for the information bits to be sent by adding redundancy to resist The impact of noise on information, channel coding technology is to ensure communication reliability. [0003] In 1948, C.E.Shannon of Bell Laboratories in the United States proposed the famous channel...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): H03M13/11
Inventor 刘原华王新梅
Owner 桂林市思奇通信设备有限公司
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com