Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

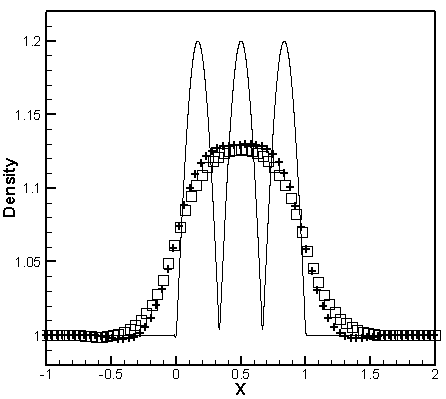
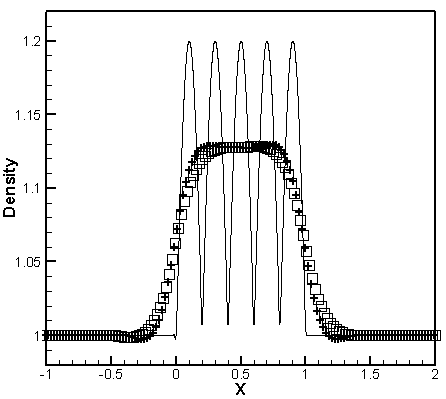
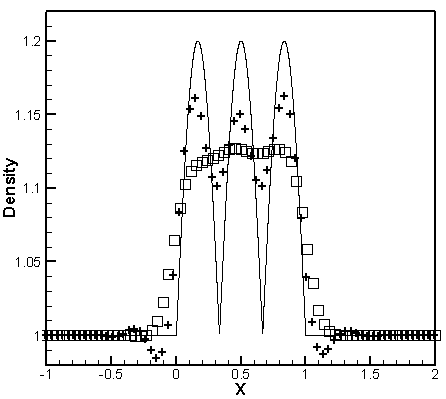
Method for simulating compressible flow field problem in multi-resolution TWENO format

An analog method and format pair technology, applied in special data processing applications, instruments, electrical digital data processing, etc., can solve problems such as insufficiency, and achieve the effect of strong robustness and easy promotion.

Inactive Publication Date: 2019-07-30
NANJING UNIV OF AERONAUTICS & ASTRONAUTICS
View PDF2 Cites 5 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

However, the methods mentioned above cannot be directly used in the ENO interpolation format, because these methods do not satisfy the rule of adding one interpolation point to the template at a time

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Method for simulating compressible flow field problem in multi-resolution TWENO format
  • Method for simulating compressible flow field problem in multi-resolution TWENO format
  • Method for simulating compressible flow field problem in multi-resolution TWENO format

Examples

Experimental program
Comparison scheme
Effect test

example 1

[0150] Example 1: Solving the one-dimensional nonlinear Burgers' equation:

[0151]

[0152] Its initial condition is u(x, 0)=0.5+sin(πx), which meets the periodic boundary condition. Numerically calculate the solution at t = 0.5 / π. The error and numerical accuracy obtained by numerical simulation with the new finite-difference multi-resolution TWENO format are shown in Table 1. For the convenience of comparison, the errors and numerical accuracy obtained by numerical simulation using the classic WENO-JS format are also listed in Table 1.

[0153] Table 1

[0154]

[0155]

example 2

[0156] Example 2: Solving the two-dimensional nonlinear Burgers' equation:

[0157]

[0158] Its initial condition is u(x, y, 0)=0.5+sin(π(x+y) / 2), which satisfies the periodic boundary condition. Numerically calculate the solution at t = 0.5 / π. Table 2 shows the errors and numerical accuracy obtained by numerical simulation using the new finite difference multi-resolution TWENO format. For the convenience of comparison, the errors and numerical accuracy obtained by numerical simulation using the classic WENO-JS format are also listed in Table 2.

[0159] Table 2

[0160]

[0161]

example 3

[0162] Example 3: Solving the one-dimensional Euler equation:

[0163]

[0164] where ρ is the density, u is the velocity in the x direction, E is the total energy, and p is the pressure. The initial conditions are ρ(x,0)=1+0.2 sin(x), u(x,0)=1, p(x,0)=1, γ=1.4. The calculation area of ​​x is [0, 2π], which satisfies the periodic boundary conditions. The exact solution of the density is ρ(x, t)=1+0.2sin(x-t), and the solution at t=2 is numerically calculated. The error and numerical accuracy obtained by numerical simulation with the new finite difference multi-resolution TWENO format are shown in Table 3. For the convenience of comparison, the errors and numerical accuracy obtained by numerical simulation using the classic WENO-JS format are also listed in Table 3.

[0165] table 3

[0166]

[0167]

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention relates to a method for simulating a compressible flow field problem in a multi-resolution TWENO format, and provides a brand new finite difference multi-resolution trigonometric function weighted essentially non-oscillatory format, and is used for numerical simulation of a compressible flow field problem. Innovative points of the method are as follows: only information on a spatialcenter nesting hierarchical structure is used, and any equivalent multi-resolution is not introduced; the adopted linear weight does not need to be calculated through complicated numerical values to obtain a theoretically optimal solution, and the solution can be artificially set to be any positive number meeting the sum of 1. Compared with a classical WENO format, the method is simpler and more convenient, higher in robustness and easier to popularize to a high-dimensional space. Finally, the novel finite difference multi-resolution TWENO format effectively simulates a plurality of classicalhyperbolic conservation law equation problems in a numerical mode, and the effectiveness and reliability of the method are fully verified.

Description

technical field [0001] The invention belongs to the technical field of computational fluid dynamics engineering, and specifically relates to a multi-resolution (multi-resolution) TWENO format simulation method for compressible flow field problems, which is a new type of finite-difference multi-resolution trigonometric function polynomial frame weighted and basically non-oscillating Format calculation method. Background technique [0002] In engineering applications, it is very important to construct a robust, efficient and high-precision numerical simulation method for solving aerodynamic problems. In 1959, Godunov proposed a first-order precision numerical simulation scheme for solving flow field problems. The numerical simulation method with first-order accuracy will not cause non-physical numerical oscillations when capturing shock waves, but it will over-smooth strong discontinuities, which are of great significance to the follow-up research of the problem. Therefore, i...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G06F17/50
CPCG06F30/23G06F2119/06
Inventor 王延萌朱君
Owner NANJING UNIV OF AERONAUTICS & ASTRONAUTICS
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com