Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

A software reliability growth model for introducing faults based on Weibull distribution

A growth model and reliability technology, applied in the field of software reliability growth model

Inactive Publication Date: 2019-05-28
SHANXI UNIV
View PDF5 Cites 11 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

In fact, not only may fault introduction be linear, exponentially distributed, or proportional to the number of faults removed, but fault introduction may also be a more complex variation
It may be affected by the environment, tools, and debugger's ability and technology during the debugging process.

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • A software reliability growth model for introducing faults based on Weibull distribution
  • A software reliability growth model for introducing faults based on Weibull distribution
  • A software reliability growth model for introducing faults based on Weibull distribution

Examples

Experimental program
Comparison scheme
Effect test

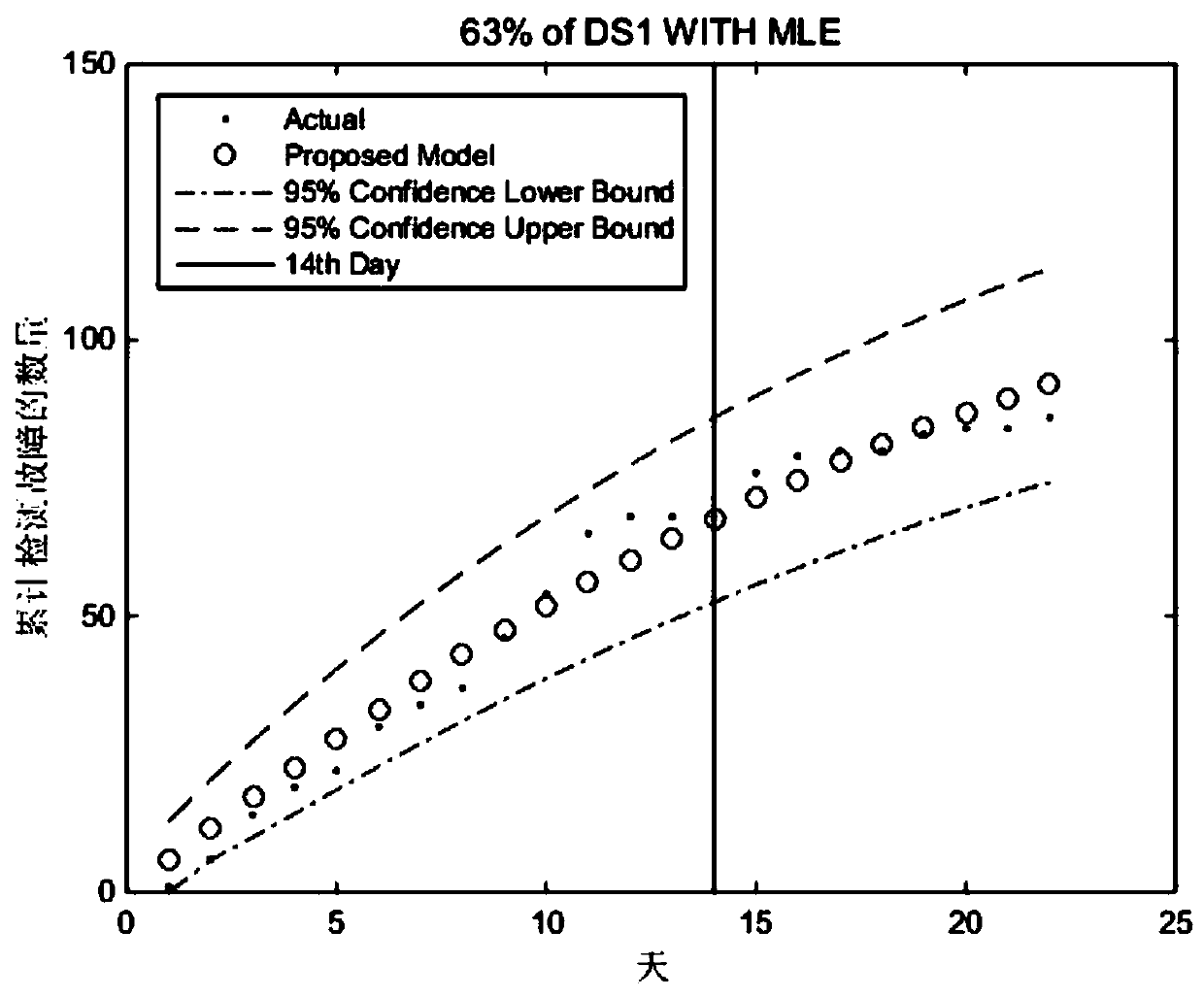
Embodiment 1

[0109] A software reliability growth model based on Weibull distribution introducing faults is characterized by comprising the following steps:

[0110] (1) The function of the total number of faults is expressed by the following formula:

[0111] a(t)=aF(t)+C (3)

[0112] Among them, a(t) represents the function of the total number of faults, a represents the total number of faults that are expected to be introduced in the end, F(t) is the distribution function of faults introduced, and C is the number of faults in the initially expected software;

[0113] (3) The fault introduction intensity function is expressed by the following formula:

[0114] η(t)=aF'(t) (4)

[0115] where η(t) means F'(t) means

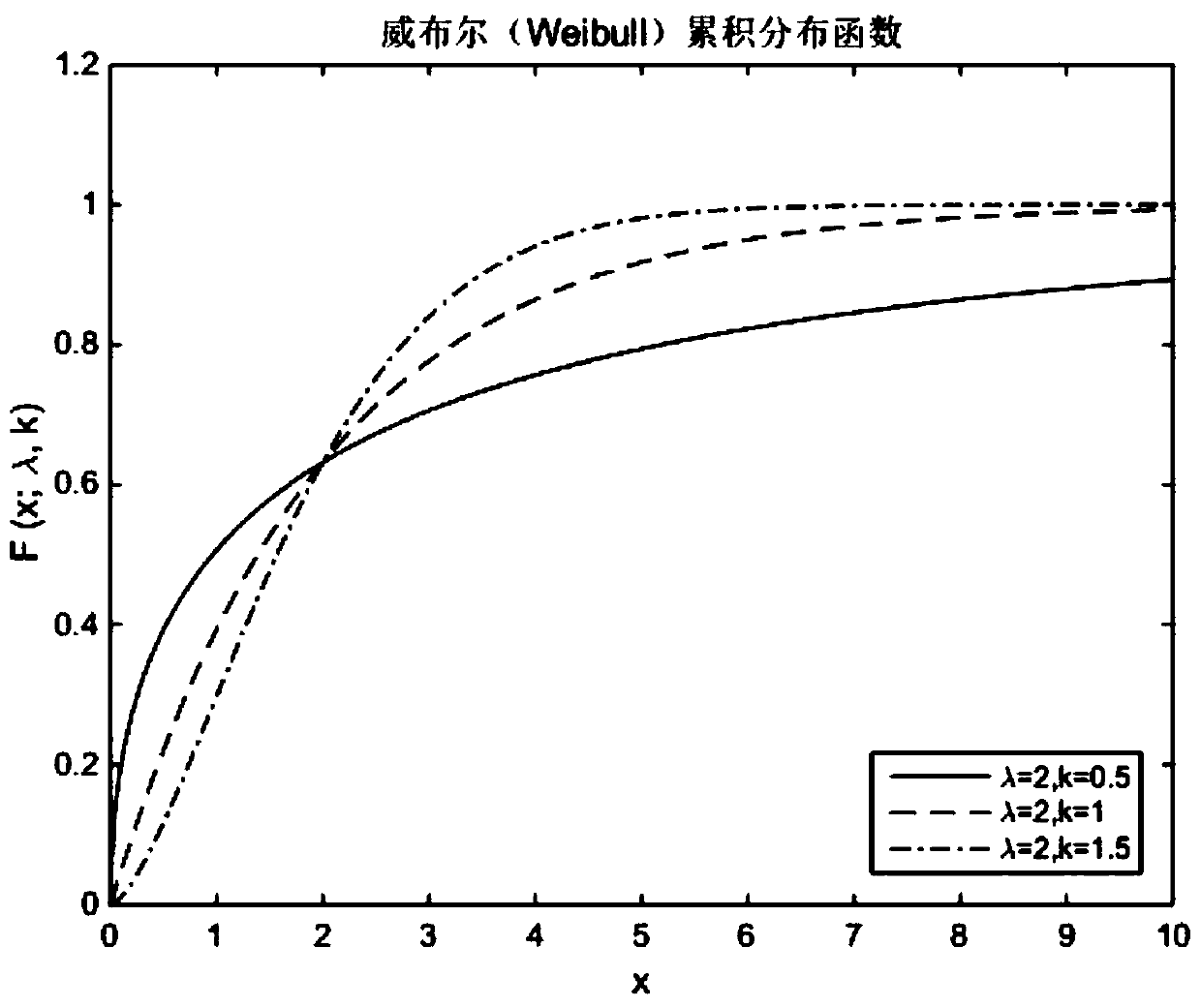
[0116] (3) Assuming that the total number of faults function (3) obeys the Weibull distribution, that is, the total number of faults function a(t) and the fault introduction intensity function η(t) can be expressed as:

[0117] a(t)=aF(t)+C=a[1-exp(-αt d )]+C (5)

[0118...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention belongs to the technical field of software reliability growth models, and particularly relates to a software reliability growth model for introducing faults based on Weibull distribution. In order to solve the above problems, the invention adopts the technical scheme that the software reliability growth model for introducing faults based on Weibull distribution comprises the following steps of (1) constructing a fault total number function; (2) constructing a fault introduction intensity function; (3) assuming a fault total number function to conform to the Weibull distribution,and constructing a fault total number function a (t) and a fault introduction intensity function eta (t); and (4) investigating the change condition of the fault introduction rate along with the testtime according to the fault introduction process, so that the fault introduction intensity function can be rewritten in the following form.

Description

technical field [0001] The invention belongs to the technical field of software reliability growth models, in particular to a software reliability growth model based on Weibull distribution introduced faults. Background technique [0002] Software testing and debugging is a complex process. Among them, the ability and technology of testers and debuggers have an important impact on the detection and troubleshooting of software faults. When a fault is detected, debuggers need relevant knowledge to consider how to completely remove and repair the fault without introducing new faults. However, the debugging process will be affected by many factors, for example, the technique of the debugger, the tools used for debugging, the debugging environment, and the psychological changes of the debugger during the debugging process. These factors will affect whether the debugger will introduce new faults when removing faults. People generally call it imperfect debugging when a new fault...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): G06F11/36
Inventor 王金勇
Owner SHANXI UNIV
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com