Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

H<infinite> fault tolerance control method of network linear parameter change system with short time delay and data packet loss

A linear parameter change, fault-tolerant control technology, applied in the direction of adaptive control, general control system, control/regulation system, etc., can solve the problems of property loss, even casualties, difficult to repair, etc.

Active Publication Date: 2018-07-24
龙岩煜联信息技术有限公司
View PDF8 Cites 10 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

Many practical systems can be described by such models, such as aircraft systems, wind energy conversion systems, etc. Once such complex systems fail, it may cause major property losses and even casualties. Therefore, it is necessary to study how to reduce catastrophic accidents , environmental pollution and personal and property losses, improving the reliability and safety of modern complex control systems has very important social and economic significance
Especially due to the application of the network, there may be a certain distance between the controller and the actuator, once a failure occurs, it is difficult to repair it in time

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • H&lt;infinite&gt; fault tolerance control method of network linear parameter change system with short time delay and data packet loss
  • H&lt;infinite&gt; fault tolerance control method of network linear parameter change system with short time delay and data packet loss
  • H&lt;infinite&gt; fault tolerance control method of network linear parameter change system with short time delay and data packet loss

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

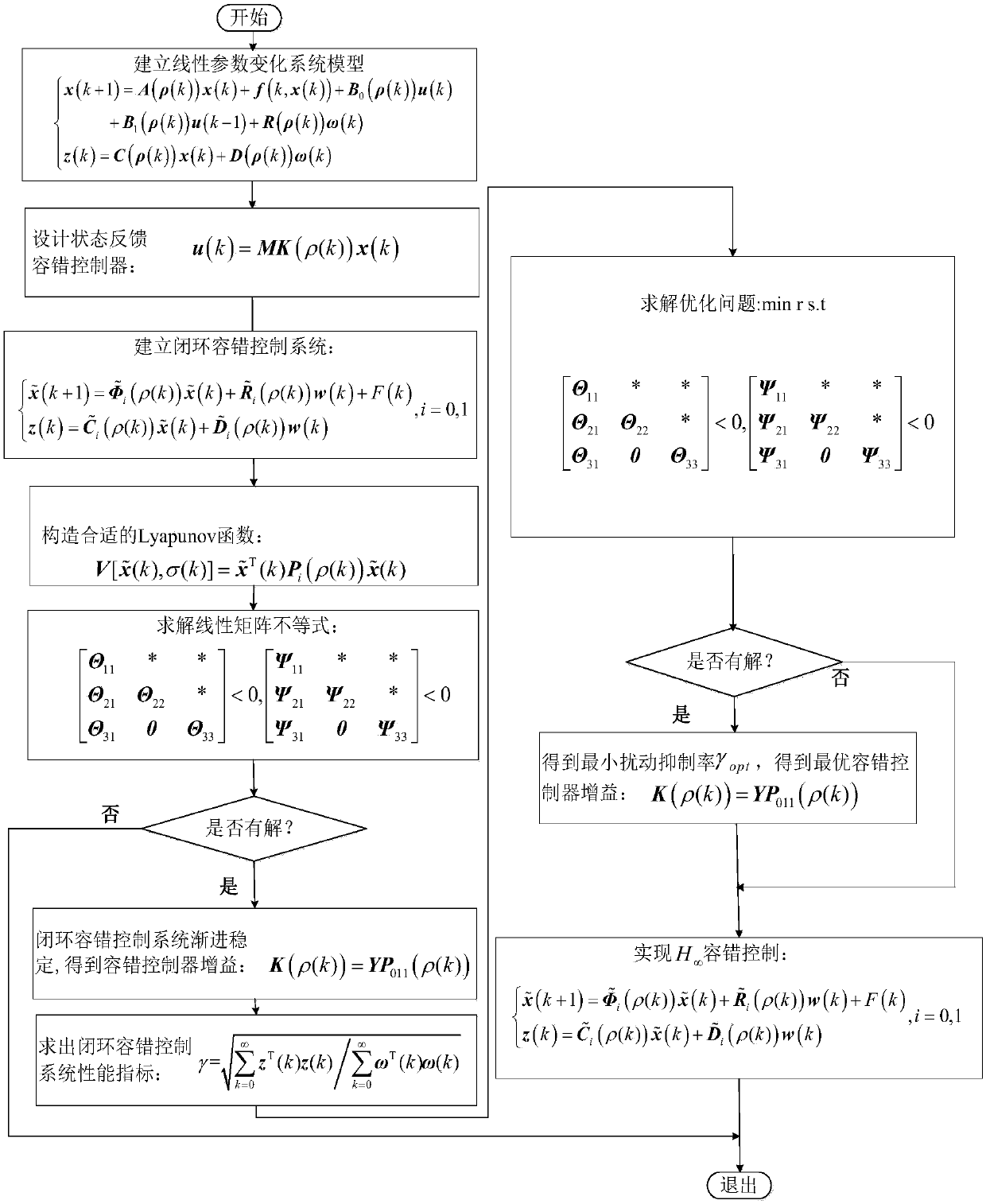
[0067] as attached figure 1 As shown, the H ∞ A fault-tolerant control method, comprising the following steps:

[0068] Step 1: Establish the linear parameter change system model as formula (1).

[0069] Step 2: Design the state feedback controller as formula (2).

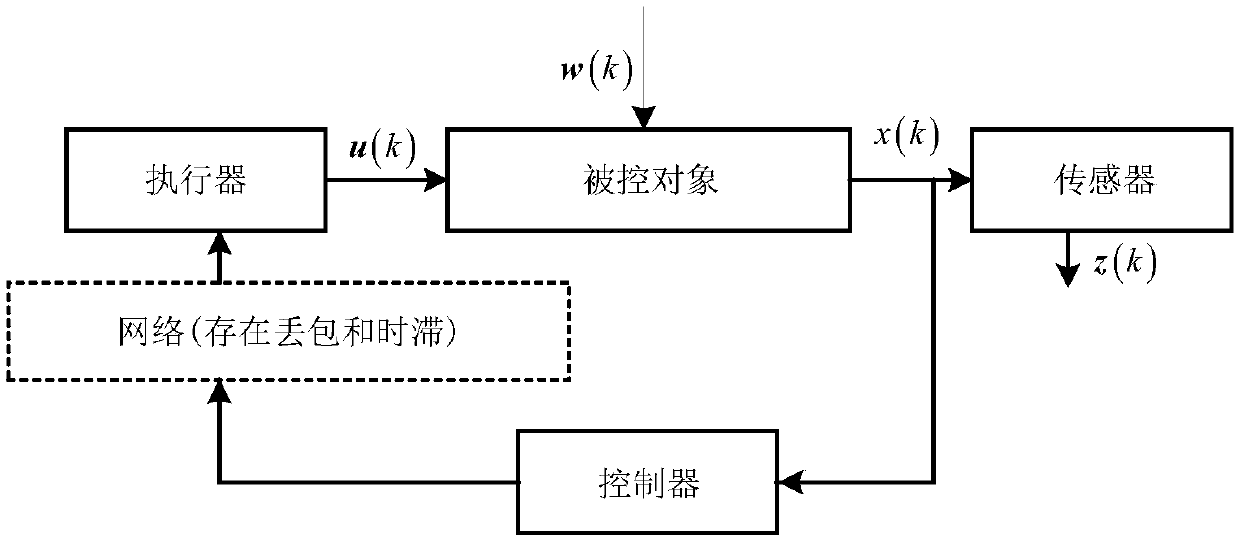
[0070] Step 3: Establish the closed-loop fault-tolerant control system of the networked linear parameter change system with short delay and data packet loss as formula (3).

[0071] Step 4: Construct a suitable Lyapunov function:

[0072]

[0073] where P i (ρ(k))=diag{P i11 (ρ(k)),P i22 (ρ(k))}, i=0,1.

[0074] when ω k = 0, by formula (3):

[0075]

[0076] in,

[0077]

[0078] Step 5: Based on the Lyapunov function constructed in step 2, using the Lyapunov stability theory and the linear matrix inequality analysis method, the closed-loop fault-tolerant control system of the networked linear parameter variation system with short delay and data packet loss is asymptotically stable and H ∞ A ...

Embodiment 2

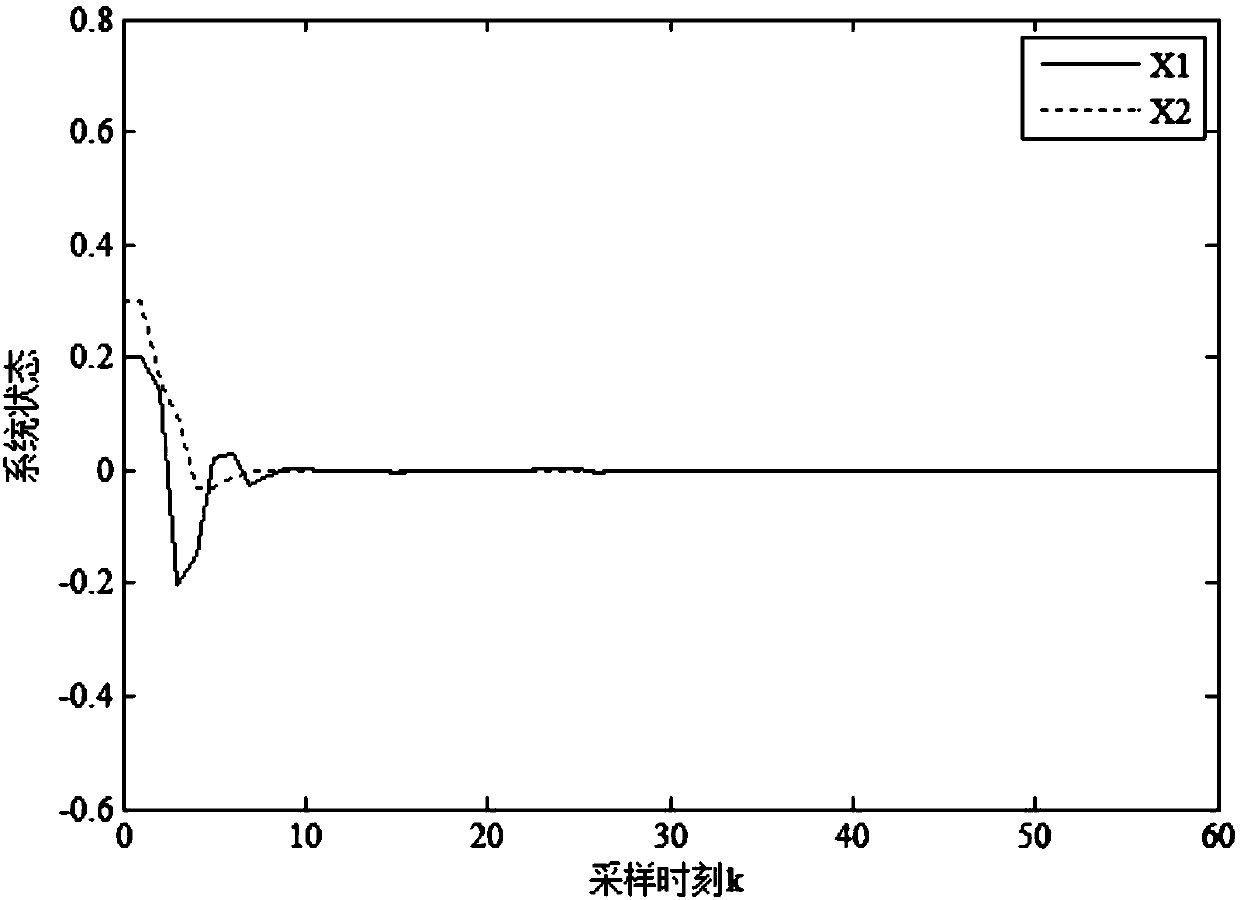
[0117] Adopt the H of a class of networked linear parameter change system with short delay and data packet loss proposed by the present invention ∞ The fault-tolerant control method, the specific implementation method is as follows:

[0118] A wind energy generator is transformed into a linear parameter change system, its mathematical model is formula (1), and its system parameters are given as:

[0119]

[0120]

[0121] Among them, ρ(k)=sin(k) 2 is a time-varying parameter, and the disturbance signal is selected as ω k =1 / k 2 , the Markov chain state transition probability matrix of random packet loss is:

[0122] Choose 3 random failure scenarios:

[0123] 1) The expectation of random failure is Variance is

[0124] 2) The expectation of random failure is Variance is

[0125] 3) The expectation of random failure is Variance is

[0126] Choose a basis function: f 1 (ρ(k))=1, f 2 (ρ(k))=sin(k) 2 , you can get:

[0127] P 011 (ρ(k))=P 0111 +sin...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention provides an H<infinite> fault tolerance control method of a network linear parameter change system with short time delay and data packet loss, and belongs to the control field of the network linear parameter change system. Executer faults, network short time delay and data packet loss of the linear parameter change system are taken into consideration, a proper Lyapunov function is constructed to obtain a sufficient condition for stability of a closed-loop fault tolerance control system, and a sufficient condition for existence of an H<infinite> fault tolerance controller is obtained by utilizing a Lyapunov stability theorem and a linear matrix inequality analysis method. An approximation base function and gridding technology are used to approximate a solution problem of an infinite-dimension linear matrix inequality set into a solution problem of a finite-dimension linear matrix inequality set, a Matlab LMI toolset is used for solution, and a gain matrix K(rho(k))=YP011(rho(k)). The method of the invention is suitable for H<infinite> fault tolerance control of the common network linear parameter change system, the H<infinite> fault tolerance controller is less conservative, and a higher performance index gamma is obtained.

Description

technical field [0001] The invention belongs to the control field of networked linear parameter change systems, and relates to a class of networked linear parameter change systems with short time delay and data packet loss. ∞ fault-tolerant control method. Background technique [0002] Linear Parameter-Varying (LPV) system is a kind of system whose parameters are constantly changing. The elements of the state matrix of this type of system are definite functions with time-varying parameters, and the range of time-varying parameters related to the function is able to measure. Many practical systems can be described by such models, such as aircraft systems, wind energy conversion systems, etc. Once such complex systems fail, it may cause major property losses and even casualties. Therefore, it is necessary to study how to reduce catastrophic accidents , environmental pollution and personal and property losses, improving the reliability and safety of modern complex control sys...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): G05B13/04
CPCG05B13/042
Inventor 潘丰陈冬杰邹金鹏
Owner 龙岩煜联信息技术有限公司
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com