Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

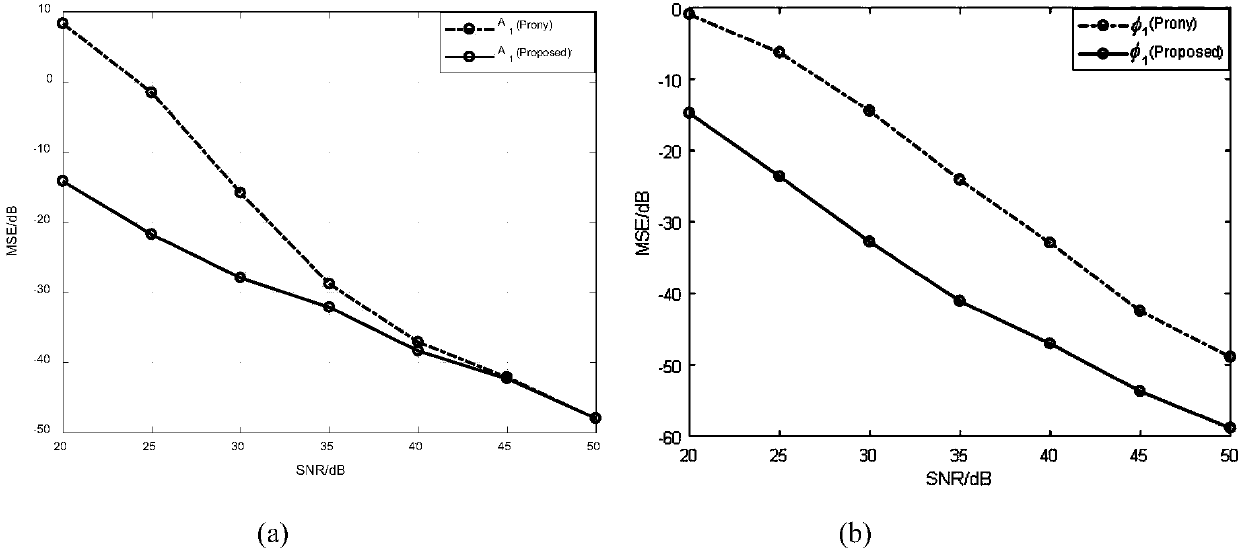
High-precision harmonic parameter estimation method based on sliding window DFT

A parameter estimation and high-precision technology, which is applied in the field of harmonic analysis of power system signals, can solve problems that affect the accuracy of harmonic analysis, fail to achieve the estimation effect, and huge calculation burden, so as to achieve enhanced robustness and robust anti-noise The effect of performance advantage and computational complexity advantage

Active Publication Date: 2018-05-15
南京福致通电气自动化有限公司 +1
View PDF4 Cites 15 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

However, it has inherent shortcomings such as frequency aliasing, spectrum leakage, and fence effects, which affect the accuracy of harmonic analysis.
Improvements such as windowing and interpolation can reduce the estimation error caused by spectrum leakage and fence effects to a certain extent, but when the frequency intervals of the harmonic components are relatively close, such methods cannot work effectively
In order to improve the estimation accuracy, the iterative DFT algorithm came into being, but the introduction of iteration will generate additional calculation amount, resulting in a huge calculation burden
In addition to DFT, there are some harmonic analysis methods based on the time domain, such as the Kalman filter method. However, the implementation of these methods needs to be based on the known characteristics of the signal data to be processed, and the state matrix also needs to be accurate in advance. definition, otherwise the pre-estimated effect will not be achieved

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • High-precision harmonic parameter estimation method based on sliding window DFT
  • High-precision harmonic parameter estimation method based on sliding window DFT
  • High-precision harmonic parameter estimation method based on sliding window DFT

Examples

Experimental program
Comparison scheme
Effect test

Embodiment Construction

[0030] The present invention will be further explained below in conjunction with the accompanying drawings.

[0031] A high-precision harmonic parameter estimation method based on sliding window Fourier transform (SWDFT). The multi-frequency signal of the power system under the noise background is:

[0032]

[0033] In the formula, s(t) represents the pure harmonic signal part containing the fundamental frequency component at time t, and q(t) represents the mean value is 0 and the variance is Additive white noise of , M is the number of harmonics, f m 、a m and φ m Respectively represent the frequency, amplitude and phase corresponding to the mth harmonic.

[0034] with period T s The signal is discretely sampled to obtain a discrete sequence x(n) of L points:

[0035]

[0036] where ω m = 2πf m T s , s(n) is the pure multi-frequency harmonic signal in the model at time n, and q(n) is the additive white Gaussian noise part in the model. Since each sinusoidal com...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a high-precision harmonic parameter estimation method based on sliding window DFT (Discrete Fourier Transform), which is suitable for estimating the harmonic components of a power system. After SWDFT is carried out on a power grid signal containing harmonic components in the power system, the transformation result has the same linear relationship as the original time domainsignal, namely, the transformed sequence still retains the mathematical structure of a multi-frequency signal, so that parameters can be estimated by using the existing harmonic analysis model. In addition, SWDFT enhances the signal-to-noise ratio of each component, and suppresses the interference of noise on the estimation performance. In the method, SWDFT is carried out on a harmonic signal inthe time domain first, and then, a Prony algorithm is adopted for the transformation result to obtain the estimated values of the frequency, amplitude and phase of each harmonic component of the signal. In order to enhance the estimation performance and robustness of the algorithm, a complex least squares (PLS) criterion is introduced to expand the original Prony algorithm. Therefore, the precision of parameter estimation is improved.

Description

technical field [0001] The present invention relates to a method for estimating harmonic parameters of stationary periodic signals, specifically a method for estimating harmonic parameters with high precision based on sliding window Fourier transform (SWDFT) and Prony algorithm, which is used for estimating harmonic frequency , amplitude and phase parameters, belonging to the technical field of power system signal harmonic analysis. Background technique [0002] With the development of power electronics technology and the expansion of industrial production, electronic components and nonlinear loads in power systems are increasing. While nonlinear loads bring huge economic benefits, they also inject a large number of high-order harmonics into the power grid. Many hazards, such as motor overheating, power system relay protection abnormality, transmission line loss increase, etc. At present, the treatment of harmonics mostly adopts the measures of injecting the corresponding ...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G01R23/16
CPCG01R23/16
Inventor 王开薛峰张琳谢庆明
Owner 南京福致通电气自动化有限公司
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com