Approximation method of label set probability density function
A technique of probability density function and approximation method, applied in the field of statistical signal processing, which can solve the problems of explosive growth of combination number, increase of function dimension, and unfavorable engineering application of set probability density function.
- Summary
- Abstract
- Description
- Claims
- Application Information
AI Technical Summary
Problems solved by technology
Method used
Image
Examples
Embodiment Construction
[0039] In order to describe the content described in the present invention for convenience, at first do following term definition:
[0040] Random set: refers to a given state space Depend on All finite subsets of form a hyperspace then defined in A random variable on is called a random set.
[0041] Set probability density function: refers to the probability density function of a random set.
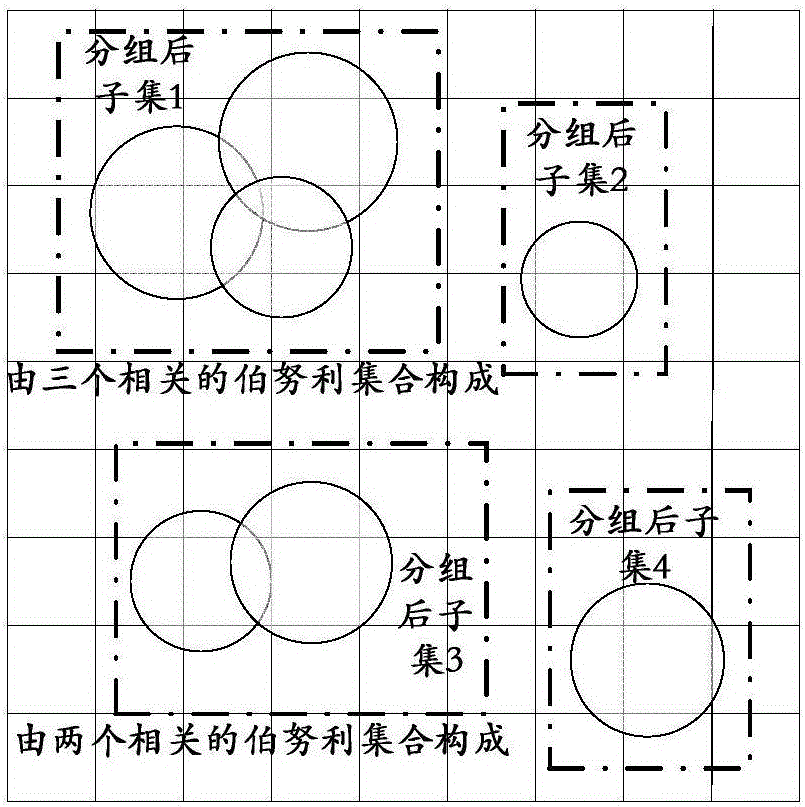
[0042] Bernoulli set: refers to any random set X, if its probability density function is
[0043]
[0044] Then the random set X is called a Bernoulli set, where r represents the probability of the existence of the target, and p(x) is the probability density function under the condition of the existence of the target.
[0045] Set edge probability density function: refers to any random set X, the probability density function of any subset thereof is called the set edge probability density function of the subset.
[0046] Set cardinality: refers to the number of elements in ...
PUM
 Login to View More
Login to View More Abstract
Description
Claims
Application Information
 Login to View More
Login to View More - R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com