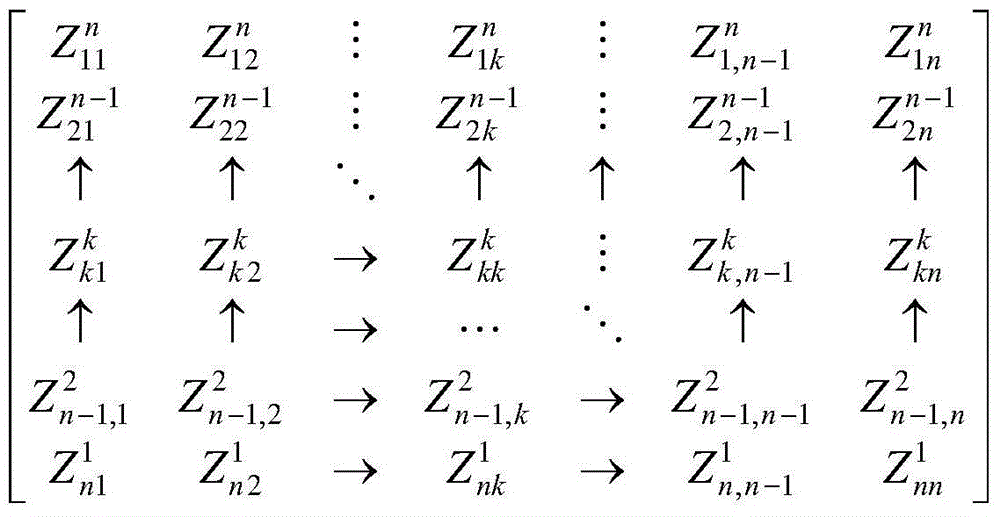Method for solving nodal impedance matrix of electric system on basis of triangular decomposition method of A=LDU
A technology of node impedance matrix and triangular decomposition, applied in complex mathematical operations and other directions, can solve problems such as large amount of calculation, long calculation time, and no consideration of the symmetry of Z-array elements, and achieve the effect of simplifying the calculation.
- Summary
- Abstract
- Description
- Claims
- Application Information
AI Technical Summary
Problems solved by technology
Method used
Image
Examples
Embodiment Construction
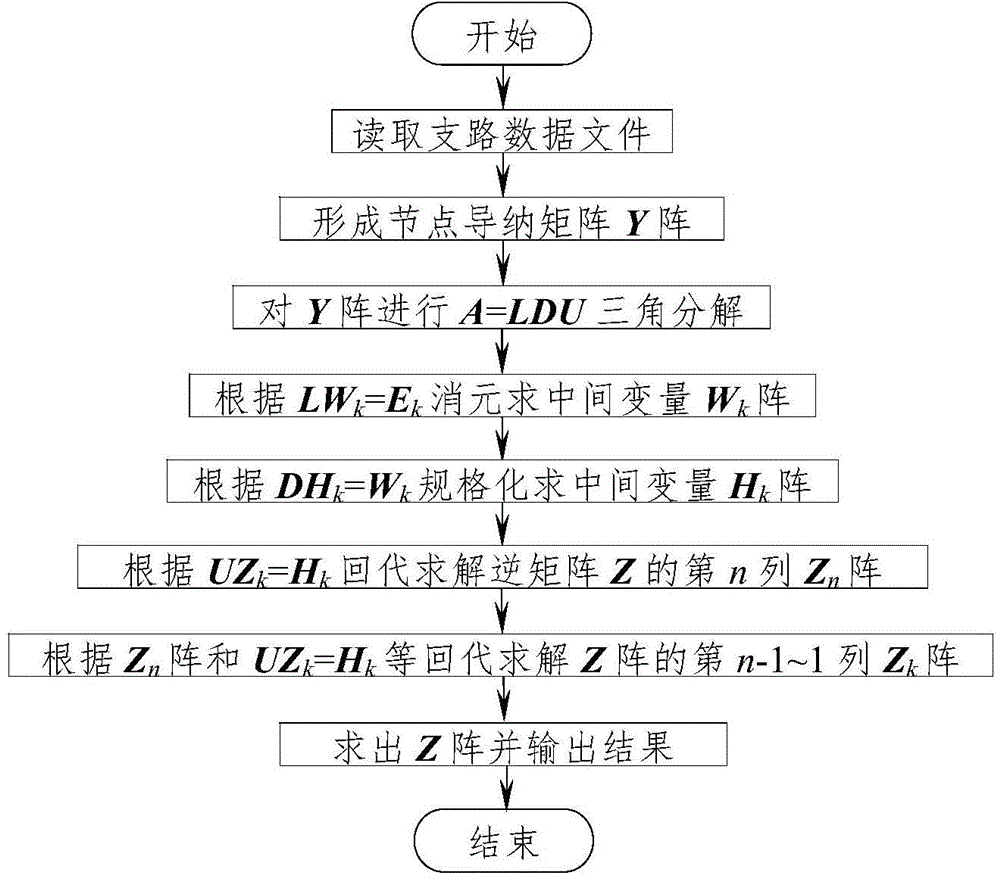
[0038] The present invention will be further illustrated by the following examples.
[0039]Example 1 Taking the n×n order node system as an example, compare the process of solving Z matrix elements with the traditional LDU triangular decomposition method and the method of the present invention. The comparison results are shown in Table 1.
[0040] Table 1 Comparison of the traditional LDU triangular decomposition method and the method of the present invention for solving the Z matrix element process
[0041]
[0042] It can be seen from Table 1 that:
[0043] (1) The traditional LDU triangular decomposition method to solve the process of Z matrix elements: a column of Z k All array elements are solved. The whole process can be expressed as: According to YZ k =E k , let Y=LDU, get LDUZ k =E k . LDUZ k =E k Can be further decomposed into: LW k =E k , DH k =W k , UZ k =H k Three equations. Each of these three equations has n equations, and each equation needs...
PUM
 Login to View More
Login to View More Abstract
Description
Claims
Application Information
 Login to View More
Login to View More - R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com