Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Magnetic resonance parallel imaging method of multi-constraint sliding window

An imaging method and sliding window technology, which are applied in magnetic resonance measurement, measurement using nuclear magnetic resonance imaging system, measurement of magnetic variables, etc., can solve problems such as noise amplification, consistent interpolation coefficients, and inability to meet imaging real-time requirements.

Active Publication Date: 2013-09-04
SOUTHERN MEDICAL UNIVERSITY
View PDF5 Cites 17 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

However, if the existing methods want to utilize other correlation constraints, it is often necessary to iteratively calculate the values ​​of all K-spaces to update the interpolation coefficients, which greatly increases the computational burden
Due to the long calculation time, it cannot meet the real-time requirements of clinical imaging
In addition, the interpolation coefficients in the existing methods can only be obtained in the calibration area, and it is assumed that the correlation of the data is consistent throughout the K space. However, due to the influence of noise and other factors in practice, the interpolation coefficients are difficult The entire K space is consistent, so that the existing GRAPPA algorithm has serious noise amplification and aliasing artifacts when the acceleration factor is large

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Magnetic resonance parallel imaging method of multi-constraint sliding window
  • Magnetic resonance parallel imaging method of multi-constraint sliding window
  • Magnetic resonance parallel imaging method of multi-constraint sliding window

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

[0045] A multi-constrained sliding window magnetic resonance parallel imaging method includes the following steps in sequence.

[0046] (1) Use multi-channel coils to fully sample the middle area of ​​K-space, and respectively fit to obtain forward reconstruction constraint weights, backward reconstruction constraint weights and self-reconstruction constraint weights.

[0047] The three reconstruction constraint coefficients are calculated in the following ways.

[0048] It is assumed that the uncollected points in the window can be obtained by the linear combination of the collected points, which is called the forward reconstruction constraint. The forward constraint weight F is calculated according to the forward reconstruction constraint relationship Iy=Fx, where I is the identity matrix, x is all collected points in the window, and y is all uncollected points in the window.

[0049] Assume that the points collected in the window can be obtained by the linear combination o...

Embodiment 2

[0068] A magnetic resonance parallel imaging method with multi-constraint sliding windows comprises the following steps in sequence.

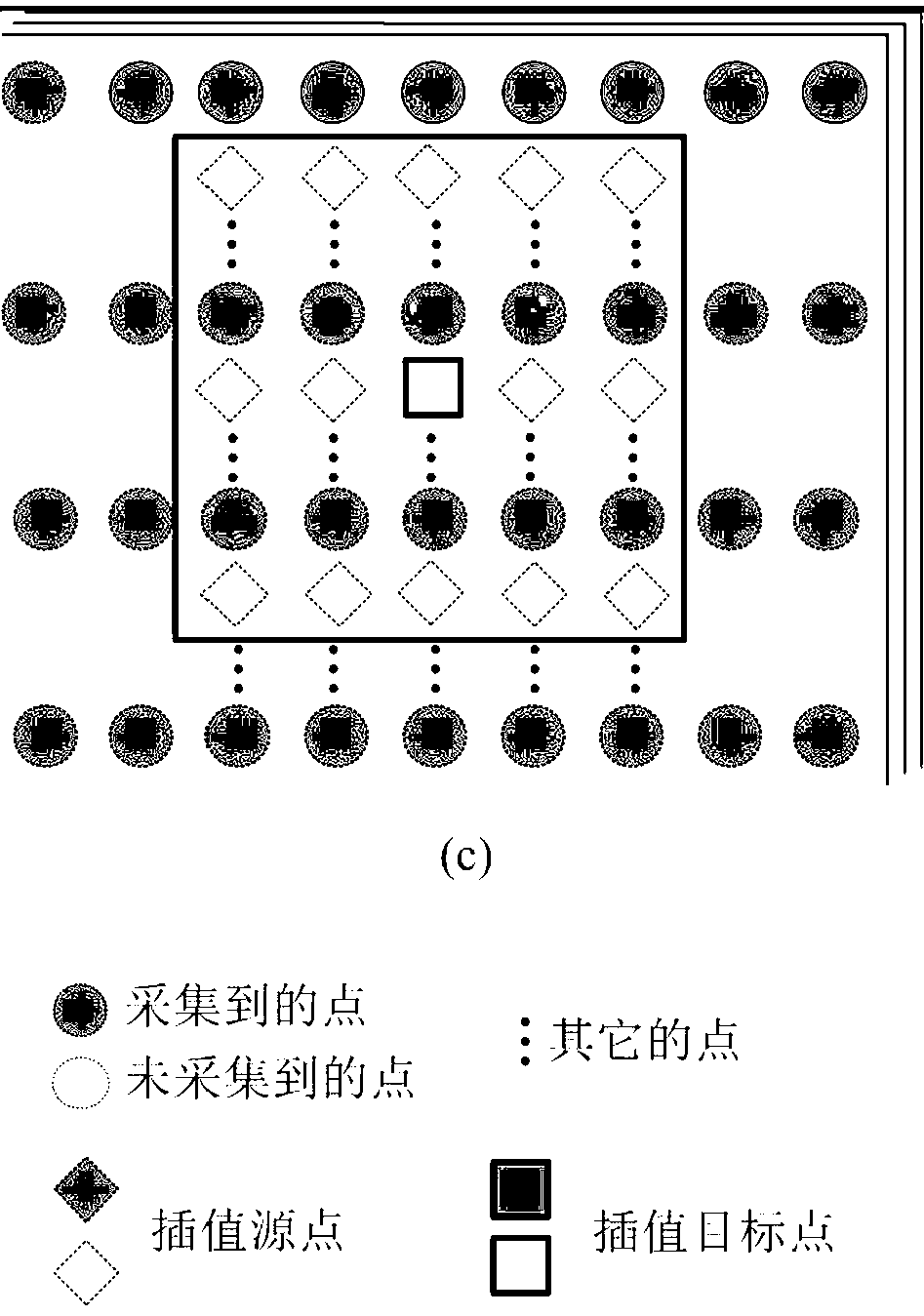
[0069] (1) Use multi-channel coils to fully sample the middle area of ​​K-space, and respectively fit to obtain forward reconstruction constraint weights, backward reconstruction constraint weights and self-reconstruction constraint weights. exist figure 1 , figure 2 and image 3 In , the black points are the collected K-space points, and the white points are the uncollected points. The interpolation source point in the constraint is represented by a diamond, while the interpolation target point is represented by a square. Interpolation is to combine the points corresponding to the K-space data collected by all coils, and only one coil is drawn in the figure for simplicity.

[0070] In the forward reconstruction constraint, the points collected in 2 rows and 5 columns are used to fit an uncollected point, such as figure 1 As shown, in the...

Embodiment 3

[0088] Through the method of the present invention, a reconstruction experiment is carried out on the K-space data acquired through acceleration, and this embodiment selects some of the experimental results for analysis and comparison.

[0089] Figure 6 Experimental results of reconstruction of head data scanned for SE sequences. Wherein (a) is the reconstruction result graph that adopts GRAPPA algorithm to obtain when external sampling acceleration factor is 3,12 calibration lines, (b) adopts the reconstruction result that the method of the present invention obtains when external sampling acceleration factor is 3,12 calibration lines Figure, (c) is the reconstruction result figure that adopts GRAPPA algorithm to obtain when external sampling acceleration factor 5, 20 calibration lines, (d) is the reconstruction result that adopts the method of the present invention to obtain when external sampling acceleration factor 5, 20 calibration lines picture.

[0090] Comparing thes...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a magnetic resonance parallel imaging method of a multi-constrain sliding window. The method sequentially comprises the following steps; (1) a multi-channel coil is utilized for conducting full sampling on a middle region of K space, and forward-direction, backward-direction and self reconstruction constraint weights are respectively obtained in a fitting mode; (2) the sliding window is selected from an accelerating sampling region, the sliding window moves respectively in the direction of frequency coding and the direction of phase coding, the collected data are utilized by the sliding window at each position for reconstructing data which are not collected, and initial estimation values, corresponding to each position, in the sliding window are obtained; (3) a linear weighted average method is adopted to gain K space output values which are not collected according to the initial estimation values, corresponding to each position, in the sliding window; (4), two dimensional Fourier transform is utilized for converting K space data which are not collected into images, and all coil images are united to obtain the final output image. Image artifacts and noise of the reconstructed image are obviously reduced.

Description

technical field [0001] The invention belongs to the technical field of magnetic resonance imaging, and in particular relates to a multi-constraint sliding window magnetic resonance parallel imaging method reconstructed in K space. Background technique [0002] Magnetic resonance imaging (MRI) has been widely used in clinical medical imaging due to its advantages of no ionizing radiation, rich tissue contrast information and non-invasive detection. However, limited by the Fourier encoding method and the Nyquist sampling theorem, MRI requires a long scan time, which not only brings some discomfort to the patient, but also easily produces motion artifacts in the reconstructed image. At the same time, the long scanning time limits the application of MRI to the imaging of moving objects, such as blood flow and heart. After decades of development, the method of accelerating acquisition by improving hardware performance, such as gradient switching rate and magnetic field strength,...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G01R33/48G01R33/561
Inventor 许林冯衍秋冯前进陈武凡
Owner SOUTHERN MEDICAL UNIVERSITY
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com