Dynamic measurement method for radial stiffness and axial stiffness of bearing
A technology of dynamic measurement and radial stiffness, which is applied in the direction of mechanical bearing testing, etc., which can solve the problems of high equipment requirements, complex structure of the test device, and difficulty in measuring the bearing stiffness.
- Summary
- Abstract
- Description
- Claims
- Application Information
AI Technical Summary
Problems solved by technology
Method used
Image
Examples
Embodiment 1
[0063] Embodiment 1: Verification of radial stiffness calculation method:
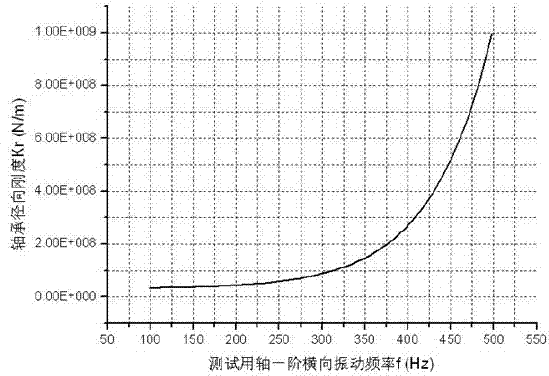
[0064] Model material density 7850 Kg / m 3 , elastic modulus 2E+11Pa, Poisson's ratio 0.3, the model structure please refer to the main parameters of the model structure in Figure 4 Example 1, the unit is mm. The finite element model of the structure was established to solve the natural frequency of the first-order lateral vibration of the test shaft under different spring stiffnesses. Some calculation results are shown in Table 1:
[0065] Table 1:
[0066] Rigidity Kr(N / m) 1E+07 2E+07 3E+07 4E+07 5E+07 6E+07 7E+07 8E+07 9E+07 1E+08 Frequency f(Hz) 98.55 137.56 166.32 189.64 209.41 226.62 241.87 255.56 267.96 279.93
[0067] Use Table 1 to fit the relationship between stiffness and frequency between 98.55 Hz and 279.93 Hz. Here, a power function is used for fitting, and the fitting function is: K r =209.06206×f 2.31959 ;
[0068] Now assume that the spring sti...
Embodiment 2
[0069] Example 2: Verification of the axial stiffness calculation method:
[0070] Model material density 7850 Kg / m 3 , elastic modulus 2E+11Pa, Poisson's ratio 0.3, the model structure please refer to Figure 8 for the main parameters of the model structure of Example 2, the unit is mm. The mass M of the structure is 16.757Kg. The finite element model of the structure is established, and the spring stiffness is set to 1E+6N / m. The natural frequency of the first-order axial vibration of the test shaft is calculated to be 38.897 Hz. Now assume that the stiffness of the spring is unknown, and use the formula to calculate the spring stiffness value, then K a =4π 2 f 2 M=4π 2 ×38.897 2 ×16.757=1000894.31N / m, compared with 1E+6N / m, the error is about 0.09%.
PUM
| Property | Measurement | Unit |
|---|---|---|
| Density | aaaaa | aaaaa |
| Elastic modulus | aaaaa | aaaaa |
Abstract
Description
Claims
Application Information
 Login to View More
Login to View More - R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com