Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Computing method for equivalent elastic modulus of two-dimensional porous materials

A technology of equivalent elastic modulus and porous materials, applied in computing, special data processing applications, instruments, etc., can solve problems such as difficult application, complex application of periodic loads, unsuitable equivalent elastic modulus of two-dimensional porous materials, etc. , to achieve the effect of small amount of calculation

Inactive Publication Date: 2012-05-02
XIAN UNIV OF TECH
View PDF4 Cites 9 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

This method is a finite element method based on characteristic elements. Although it overcomes the shortcomings of the experimental method, such as uneconomical and small sample size, the application of periodic loads is complicated, and sometimes it is difficult to apply, and it is not suitable for calculating two-dimensional irregular shapes. Equivalent Elastic Modulus of Porous Materials

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Computing method for equivalent elastic modulus of two-dimensional porous materials
  • Computing method for equivalent elastic modulus of two-dimensional porous materials
  • Computing method for equivalent elastic modulus of two-dimensional porous materials

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

[0026] Hexagonal 2D porous material along x1 、x 2 and x 3 The equivalent modulus of elasticity in the direction.

[0027] Such as figure 2 In the structure of the hexagonal two-dimensional porous material shown, each characteristic unit has 4 oblique pore walls with length d and thickness t and 2 vertical pore walls with length h and thickness t, and the pore depths are all b. x 1 The axis is perpendicular to the vertical hole wall, x 2 Axis parallel to the vertical hole wall, and x 3 The axis is along the direction of the hole depth. The wall surface of the hypotenuse hole and x 1 -x 3 The included angle of the faces is θ, which is defined as the spread angle. In this embodiment, the base material is aluminum alloy, and its physical parameters related to linear elastic deformation are: Young's modulus E s = 68.97GPa, Poisson's ratio v s =0.35, density ρ s =2700Kg / m 3 .

[0028] Step 1. Use ANSYS / Multiphysics software to establish a hexagonal two-dimensional poro...

Embodiment 2

[0045] Calculation of Coplanar Equivalent Elastic Modulus of Triangular Two-Dimensional Porous Materials

[0046] In this embodiment, the base material is aluminum alloy, and its physical parameters related to the linear elastic stage are: Young's modulus is E s =68.97GPa, Poisson's ratio is v s =0.35, the density is ρ s =2700Kg / m 3 .

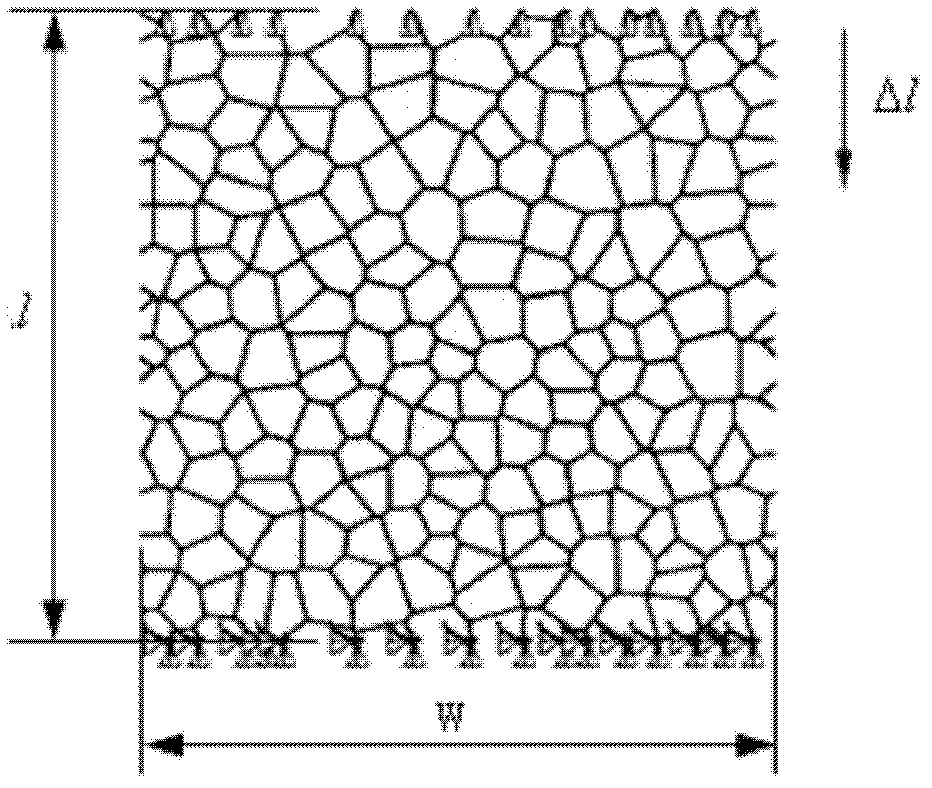
[0047] ANSYS / Multiphysics software was used to establish the finite element calculation model of the two-dimensional porous material, and the model was obtained as follows: Figure 7 shown, along the l 1 The direction is called coplanar, and the coplanar equivalent elastic modulus is defined as E 1 * . The side length of the triangle in the periodic feature unit is 5mm, the base angle is 60°, and the wall thickness is 0.15mm. According to the ASTM tensile test standard, the number of complete elements in the length and width directions of the two-dimensional porous material finite element calculation model should be m 1 = 51 and n 1 =...

Embodiment 3

[0052] Calculation of Coplanar Equivalent Elastic Modulus of Square Two-Dimensional Porous Materials

[0053] In this embodiment, the base material is aluminum alloy, and its physical parameters related to the linear elastic stage are: Young's modulus is E s =68.97GPa, Poisson's ratio is v s =0.35, the density is ρ s =2700Kg / m 3 .

[0054] ANSYS / Multiphysics software was used to establish the finite element calculation model of the two-dimensional porous material, and the model was obtained as follows: Figure 8 shown, along the l 1 The direction is called coplanar, and the coplanar equivalent elastic modulus is defined as E 1 * . The side length of the square in the periodic feature unit is 5mm, and the wall thickness is 0.15mm. According to the ASTM tensile test standard, the number of complete elements in the length and width directions of the two-dimensional porous material finite element calculation model should be m 1 = 44 and n 1 =26, then the length l of the ...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a computing method for an equivalent elastic modulus of two-dimensional porous materials. The computing method comprises the following steps of: firstly building a finite element computation model of the two-dimensional porous materials, then dividing a grid, exerting a displacement load, obtaining a resultant force of all nodes at fixed ends of loads along a direction of the equivalent elastic modulus, and finally obtaining the equivalent elastic modulus of the finite element computation model of the two-dimensional porous materials according to formula computing. Themethod disclosed by the invention is applicable to solving of the equivalent modulus of the two-dimensional porous materials with regular and irregular conformation in a covariance direction, moreover, size limit of a product is avoided, and the computing method has the advantages of simplicity and rapidness.

Description

technical field [0001] The invention belongs to the technical field of calculation of static performance parameters of two-dimensional porous materials, and relates to a calculation method of equivalent elastic modulus of two-dimensional porous materials. Background technique [0002] Two-dimensional porous materials can be divided into regular and irregular configurations. Among them, the common structural forms of regular configuration two-dimensional porous materials are: triangle, alternating triangle, rectangle, alternating rectangle, convex hexagon, concave hexagon, Sparse X-shape, dense X-shape, circular, elliptical, corrugated and sinusoidal, etc. Regardless of the configuration of the two-dimensional porous material, when a low-speed static compression load is applied along the co-planar or non-planar direction, the stress-strain curve will include deformation processes such as linear elasticity, plastic yielding, plateau region and densification. The slope of the ...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): G06F17/50
Inventor 孙德强卫延斌刘淼
Owner XIAN UNIV OF TECH
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com