Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Multi-channel bipolar sparse spectrum inversion method based on Hessian matrix constraint

A sparse spectrum and spectrum inversion technology, applied in the field of oil and gas and geophysical exploration, can solve the problems of inability to reflect the distribution of formation reflection coefficients, lack of spatial coherence of reflection coefficients, and dislocation, etc., to achieve rich geological and reservoir information, high Resolution, the effect of increasing the resolution

Pending Publication Date: 2022-07-29
CHINA PETROLEUM & CHEM CORP +1
View PDF2 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0007] However, due to the lack of spatial coherence of the reflection coefficients of each channel, phenomena such as "displacement" and "bifurcation" appear on the reflection coefficient profile, which cannot reflect the real distribution of the formation reflection coefficient, and there is still no effective solution.

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Multi-channel bipolar sparse spectrum inversion method based on Hessian matrix constraint
  • Multi-channel bipolar sparse spectrum inversion method based on Hessian matrix constraint
  • Multi-channel bipolar sparse spectrum inversion method based on Hessian matrix constraint

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

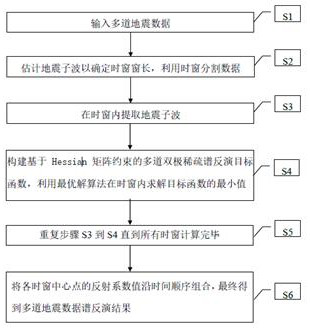
[0062] like figure 1 As shown, the multi-channel bipolar sparse spectrum inversion method based on Hessian matrix constraints includes the following steps:

[0063] S1. Input multi-channel seismic data.

[0064] S2. Estimate the seismic wavelet to determine the length of the time window, and use the time window to divide the data:

[0065] First, estimate the dominant frequency f of the wavelet of the multi-channel seismic data s(x,t) m :

[0066]

[0067] Among them, S(x,f) represents the amplitude spectrum of the multi-channel signal s(x,t), and M is the number of seismic traces.

[0068] Take the main frequency as f w The length of the zero-phase rake wavelet is used as the window length of the time window, so that the center sampling point of the time window corresponds to the position of the main peak of the zero-phase rake wavelet.

[0069] After that, set the moving window step as a time sampling interval of the multi-channel seismic data, and use the time windo...

Embodiment 2

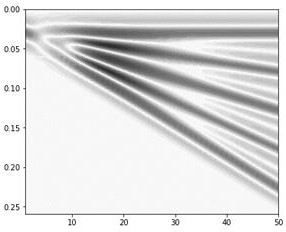
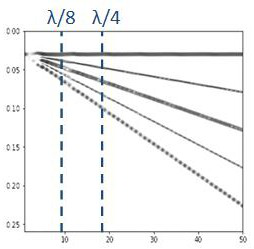
[0098] The sand-mud interbed wedge-shaped model seismic forward modeling section was inverted using the multi-channel bipolar sparse spectrum inversion method based on Hessian matrix constraints described in Example 1. The sand-mud interbed wedge-shaped model seismic forward modeling section was as follows: figure 2 As shown, the calculated reflection coefficient profile is as follows image 3 shown.

Embodiment 3

[0100] The Marmousi model seismic forward profile is inverted using the multi-channel bipolar sparse spectrum inversion method based on Hessian matrix constraints described in Example 1. The Marmousi model seismic forward profile is as follows: Figure 5 As shown, the calculated reflection coefficient profile is as follows Image 6 shown.

[0101] Will image 3 , Image 6 The inversion results shown are the same as the conventional spectral inversion results ( Figure 4 , Figure 7 ), it can be seen that: the resolution capability of conventional spectral inversion for thin layers is relatively poor, when the thickness of thin layers is less than 1 / 8 wavelength, it cannot be resolved, and the position of reflection coefficients is displaced and lacks lateral continuity; based on Hessian matrix Constrained multi-channel bipolar sparse spectral inversion can resolve thin layers with a thickness less than 1 / 8 wavelength, and the reflection coefficient has good lateral continu...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention belongs to the field of oil gas and geophysical exploration, and particularly relates to a multichannel bipolar sparse spectrum inversion method based on Hessian matrix constraint. The method comprises the following steps: estimating the wavelength of seismic wavelets, determining the length of a time window, and segmenting multiple channels of seismic data; seismic wavelets in the time window are extracted; constructing a multi-channel bipolar sparse spectrum inversion objective function based on Hessian matrix constraint in the time window, and calculating a reflection coefficient of multi-channel seismic data in the time window; and combining the center reflection coefficient results of the time windows along a time sequence to obtain a spectrum inversion result of the multi-channel seismic data. According to the method, the real contact relation of the stratum can be reflected, the real pinching position of the stratum can be depicted, and high-resolution and richer geological information can be provided. While the seismic data resolution is improved, the method can ensure the transverse continuity of stratums in multi-channel data, and can provide more accurate and rich geological and reservoir information.

Description

technical field [0001] The invention belongs to the field of oil and gas and geophysical exploration, and particularly relates to a multi-channel bipolar sparse spectrum inversion method based on Hessian matrix constraints. Background technique [0002] Due to the influence of the tuning effect, the application of conventional seismic data and traditional processing methods cannot identify thin layers and small-scale geological structures with a thickness smaller than the tuning thickness, and it is difficult to provide high-precision interpretation results. The spectral inversion method does not rely on prior information such as logging data, and only needs to use the spectral information of the seismic data itself to invert the sparse reflection coefficient of the formation. This method has been extensively studied and discussed due to its ability to resolve seismic thin layers below the tuning thickness. [0003] The traditional spectral inversion method uses a single se...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G01V1/28G01V1/30
CPCG01V1/282G01V1/301
Inventor 罗红梅王长江陈攀峰杨培杰颜世翠张娟王庆华刘华夏张志敬郑文召
Owner CHINA PETROLEUM & CHEM CORP
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com