High-dimensional data completion method for wireless sensor networks based on Hankel transform
A wireless sensor network and high-dimensional data technology, applied in the field of data completion, can solve problems such as the inability to consider the impact of noise, high computational complexity, and complex operations, and achieve enhanced correlation, enriched data structure, and reduced operations. Effect
- Summary
- Abstract
- Description
- Claims
- Application Information
AI Technical Summary
Problems solved by technology
Method used
Image
Examples
Embodiment Construction
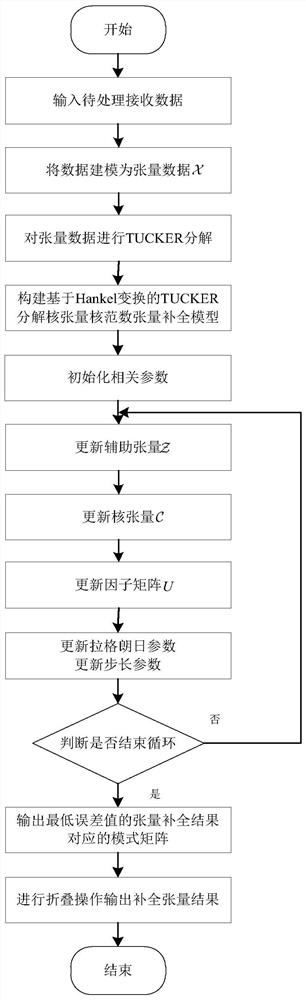
[0035] Embodiments of the present invention: a high-dimensional data completion method for wireless sensor networks based on Hankel transform, such as figure 1 shown, including the following steps:
[0036] S1, model the wireless sensor network data into tensor form according to the analysis requirements where i represents the corresponding physical dimension, such as frequency, time, power, geographic location, acceleration, speed, etc., N represents the number of physical dimensions, (I 1 ,…,I i ,…,I N ) represents the size of each physical dimension of the wireless network transmission data, represents a real number;
[0037] S2, statistical tensor data The effective element positions of , constituting the tensor data support set Ω, where Ω is the same as A logical tensor with the same dimensions and the same size, the elements only contain 0 and 1, representing a tensor Whether there is valid data at the corresponding position of ;
[0038] S3, perform TUCKER ...
PUM
 Login to View More
Login to View More Abstract
Description
Claims
Application Information
 Login to View More
Login to View More - R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com