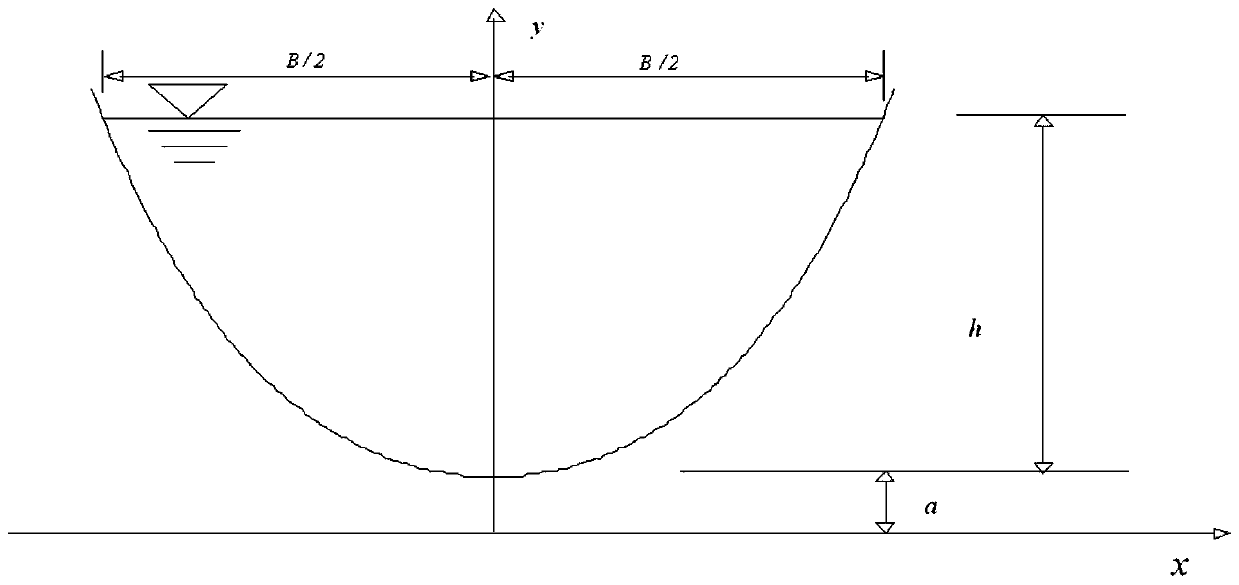
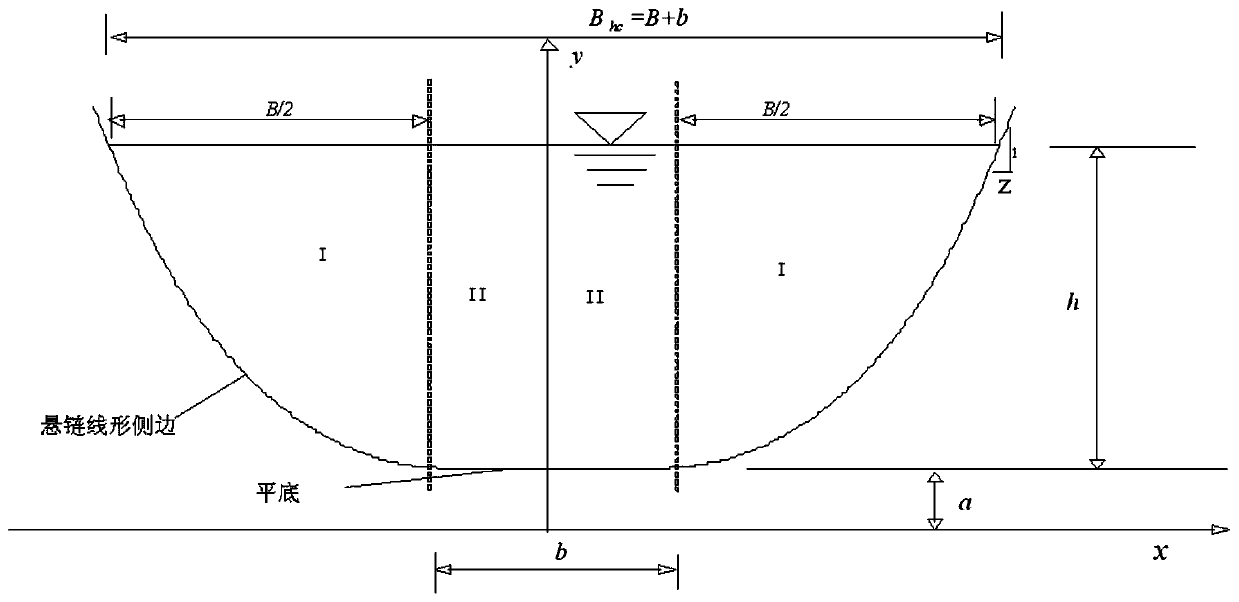
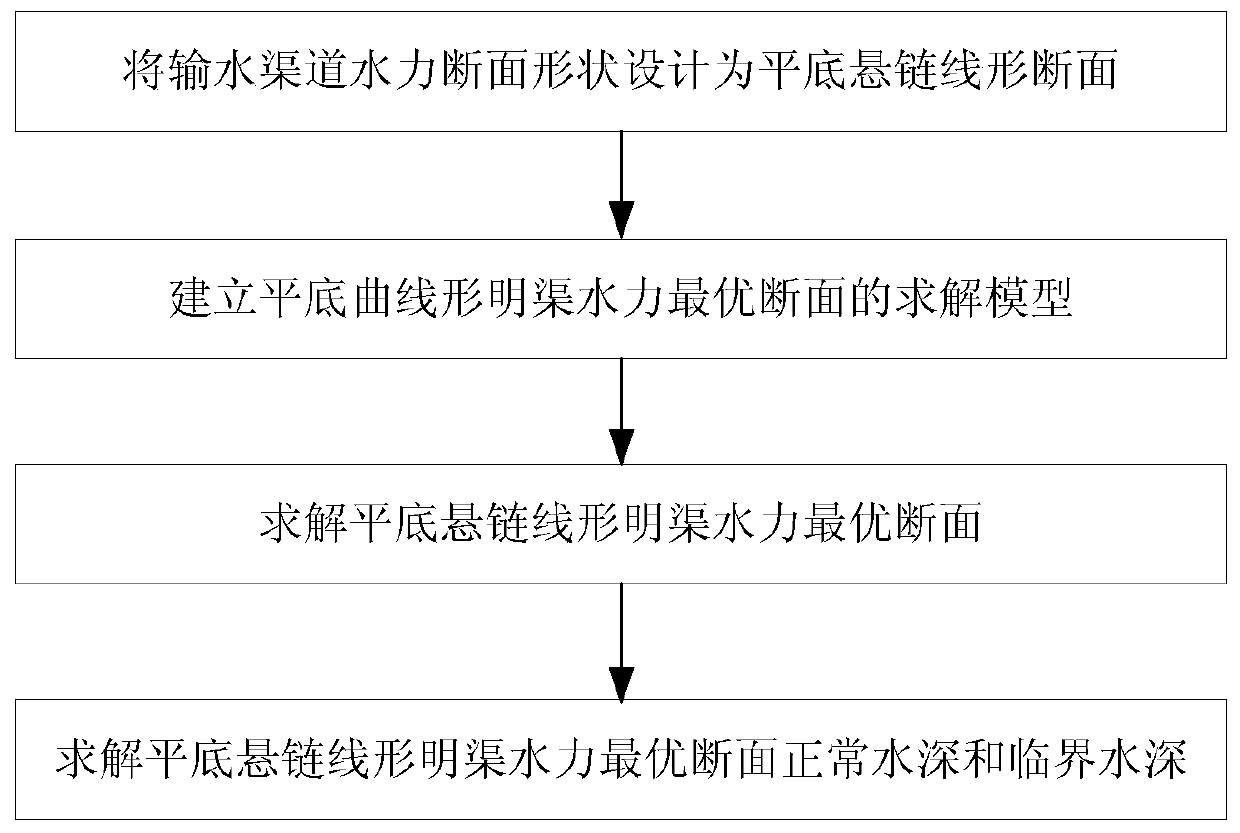
Novel flat-bottom catenary open channel and solving method of hydraulic optimal section thereof

Abstract

The invention discloses a novel flat-bottom catenary open channel and a solving method of a hydraulic optimal section thereof. An opening of the flat-bottom catenary open channel is upward, and the section of the flat-bottom catenary open channel comprises a flat bottom and two catenary side edges which are positioned on two sides of the flat bottom and are smoothly connected with the flat bottom.The solving method comprises the following steps: designing the shape of the hydraulic section of the water conveying channel into a flat-bottom catenary section; establishing a solution model of thehydraulic optimal section of the flat-bottom curved open channel; solving the hydraulic optimal section of the flat-bottom catenary-shaped open channel; and solving the normal water depth and critical water depth of the hydraulic optimal section of the flat-bottom catenary open channel. The method has the advantages that the catenary section has no stress concentration point, and the open channelis not prone to have cracks caused by stress concentration. The open channel is small in leakage, good in stability, excellent in hydraulic characteristic, high in anti-freezing capacity and the like, also has the advantages of being flexible in flat-bottom section design, low in construction difficulty, easy to manage and maintain, easy to compact at the bottom and the like, and can improve thewater delivery efficiency, reduce the water delivery loss and reduce the construction cost.

Description

technical field [0001] The invention relates to a novel flat-bottomed catenary-shaped open channel and a method for solving the optimal hydraulic section thereof, and belongs to the technical field of section planning and design of water delivery channels in irrigation areas. Background technique [0002] Open channel section design is very important for water delivery channels. A suitable channel section can not only increase flow capacity, improve water delivery efficiency, reduce water delivery loss, but also reduce construction costs. Scholars have conducted a lot of research on channel sections, and proposed many channel section optimization theories and new channel section types, so that designers can choose suitable sections according to different purposes, different hydraulic, hydrogeological conditions, and sediment content. Types of. In my country, farmland irrigation is still dominated by traditional surface irrigation, and the irrigation area of ​​open channel w...

AI Technical Summary

Problems solved by technology

But like other curved sections, it has the following disadvantages: (1) The known knowledge is that because the bottom of the curved section is a curve and the bottom width is 0, compaction equipment such as road rollers cannot compact the foundation, and the bottom is not suitable for compaction. The bottom of the section is prone to subsidence, voids, etc., which may easily cause damage to the channel, resulting in leakage and affecting the service life; (2) Due to the constraints of the curved section function, the bottom width cannot be adjusted, so the section width of the channel cannot be adjusted flexibly. On the one hand, it increases the difficulty of design, on the other hand, it makes it difficult for the curved section to be suitable for large-scale water delivery channels, nor can it be applied to wide and shallow channels (large and medium-sized channels are generally wide and shallow channels), so the design and construction are not flexible (3) the catenary-shaped section of the prior art can not often adopt different materials (in order to reduce construction cost in the project, the bottom often adopts cheap materials to reduce construction cost) ); (4) Since the construction machinery cannot enter the channel for construction, it increases the difficulty of construction and also increases the construction cost; (5) Since the bottom width of the purely curved section is 0, all construction machinery cannot enter the channel for maintenance after completion. There is no flat space for personnel to stand during maintenance, which increases the difficulty of maintenance

Research shows that the width-to-depth ratio of the trapezoidal section is (where, z is the slope coefficient), the ratio of the water surface width of the catenary section to the shape coefficient However, there has not been any study on the optimal cross-section of flat-bottomed catenary channels

Images