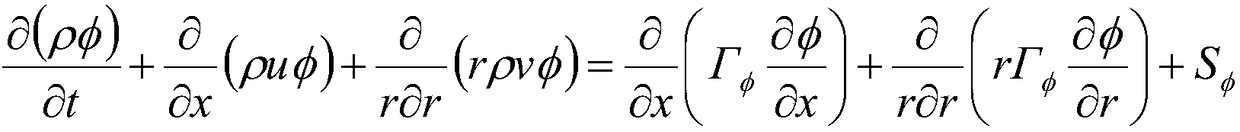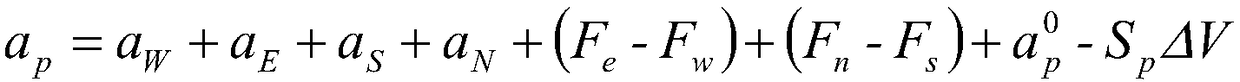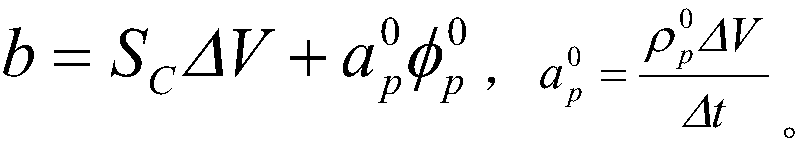Eureka AIR delivers breakthrough ideas for toughest innovation challenges, trusted by R&D personnel around the world.

Fluent-based dust explosion simulation method

A simulation method, dust explosion technology, applied in special data processing applications, instruments, electrical digital data processing, etc., can solve the problems of casualties and economic losses, and achieve the effect of simple calculation

Inactive Publication Date: 2018-08-24
HARBIN UNIV OF SCI & TECH
View PDF2 Cites 4 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

Since the first dust explosion accident in Durin Flour Mill in Italy in 1785, many serious dust explosion accidents have occurred in the world, thus causing a large number of casualties and huge economic losses.
Although scientific researchers from various countries have conducted a lot of discussions and researches on the prevention and protection of dust explosions for more than 100 years, so far, dust explosions are still an important source of danger that threatens the safe production of process industries such as modern processing, transportation, and storage. one

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Fluent-based dust explosion simulation method
  • Fluent-based dust explosion simulation method
  • Fluent-based dust explosion simulation method

Examples

Experimental program
Comparison scheme
Effect test

specific Embodiment approach 1

[0019] A fluent-based dust explosion simulation method in this embodiment,

[0020] Step 1, the gas phase conservation equation of the general form of the gas in the two-dimensional region: Perform two-dimensional expansion in the cylindrical coordinate system, and the form in the two-dimensional cylindrical coordinate system is:

[0021]

[0022] In the formula, φ is the generalized variable of velocity, temperature and component concentration; the two-dimensional area including the explosion vessel and the pipeline are all cylindrical;

[0023] Step 2: Using the finite volume method in the discretization method to calculate the fluid dynamics software FIUENT, in the integration process, make assumptions about the composition of the flux and its first-order derivative diffusion flux on the interface, and form different Discrete format, only the discrete equation derived by the finite volume method can guarantee conservation as long as the interpolation method on the inte...

specific Embodiment approach 2

[0028] Different from the specific embodiment 1, the fluent-based dust explosion simulation method of this embodiment adopts the finite volume method and requires the general conservation equation Carry out discretization to solve it, convert the integral of the partial differential equation into the discrete algebraic equation of each variable in the control volume, and solve the algebraic equation linearly. The discretization of the convection item in the equation adopts the first-order upwind method, and the discretization of the diffusion item adopts the middle self-difference format, the time discretization adopts the Guass-Seidel first-order implicit format, and the linearization equation of the two-dimensional discrete equation is:

[0029] a P φ P =a W φ W +a E φ E +a S φ S +a N φ N +b;

[0030] In the formula, the coefficient a W , a E , a S , a N Depending on the particular discretization scheme introduced in the convective term, the first-order upwind...

specific Embodiment approach 3

[0032] Different from the second specific embodiment, in this embodiment, a fluent-based dust explosion simulation method writes out that the central grid nodes of all control volumes have the formula other discrete equations of other discrete equations to form a linear algebraic equation system. The unknown quantity in the equation system is the φ value on each node. Solving this equation system can obtain the distribution of the unknown quantity φ in space; through the "guess-correction" In the process, the pressure field is calculated on the basis of the staggered grid, so as to achieve the purpose of solving the momentum equation. The calculation steps of the steady-state flow algorithm are described as follows:

[0033] First, assume a velocity distribution u 0 , v 0 ,w 0 In this way, the coefficients and constant terms in the momentum discrete equation are calculated;

[0034] 2. Assume a pressure field p * ;

[0035] 3. Solve the three momentum equations in turn t...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The present invention relates to a fluent-based dust explosion simulation method. Although numerical simulation has become an increasingly popular research tool, there is a lack of good models for describing the interaction between phases and between particles. The method provided by the present invention is characterized in that: for the gas-conservation equation of the general form of gas in thetwo-dimensional region, the finite volume method in the discretization method is used to calculate the fluid dynamics software FIUENT; in the integration process, constitutions of the convective fluxof the solved function itself on the interface and of the first derivative diffusion flux of the solved function are assumed to form different discrete formats; and only a discrete equation derived by using the finite volume method can guarantee conservation as long as the control volumes located on both sides of the interface in the interpolation method on the interface are the same. The methodprovided by the present invention is simple in calculation, and pseudo-diffusion cannot be generated when the particle has a more complex change experience and the motion value calculation of the particle can be better tracked.

Description

Technical field: [0001] The invention relates to a fluent-based dust explosion simulation method. Background technique: [0002] Industrial dust explosions involve various industries and departments. Dust can be seen everywhere in industries such as grain processing, coal mining, textile, metallurgy, and chemical industry. With the progress of the times, the maturity of technology and the expansion of production scale, industrial dust explosion accidents are easier occur. Since the first dust explosion accident in the Italian Durin flour mill in 1785, many serious dust explosion accidents have occurred in the world, which caused a large number of casualties and huge economic losses. Although scientific researchers from various countries have conducted a lot of discussions and researches on the prevention and protection of dust explosions for more than 100 years, so far, dust explosions are still an important source of danger that threatens the safe production of process ind...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): G06F17/50
CPCG06F30/20
Inventor 蒋永清迟长宇
Owner HARBIN UNIV OF SCI & TECH
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com