Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Water and sand dynamic simulation method based on bioflocculation

A technology of biological flocculation and simulation method, applied in the fields of water and sediment dynamics of biological flocculation and hydraulic engineering, which can solve the problems of complicated influencing factors and inability to accurately and comprehensively describe the actual situation.

Active Publication Date: 2015-12-23
CHINA INST OF WATER RESOURCES & HYDROPOWER RES
View PDF2 Cites 9 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

However, for the characteristics of water and sand under the influence of bioflocculation, due to the different mechanism of action, the influencing factors are more complicated because they focus on the micro-ecosystem with biological activity, and the pure physical and chemical theory can no longer describe the actual situation accurately and comprehensively.

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Water and sand dynamic simulation method based on bioflocculation
  • Water and sand dynamic simulation method based on bioflocculation
  • Water and sand dynamic simulation method based on bioflocculation

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

[0039] General idea of ​​bioflocculation-based water-sediment dynamics model system

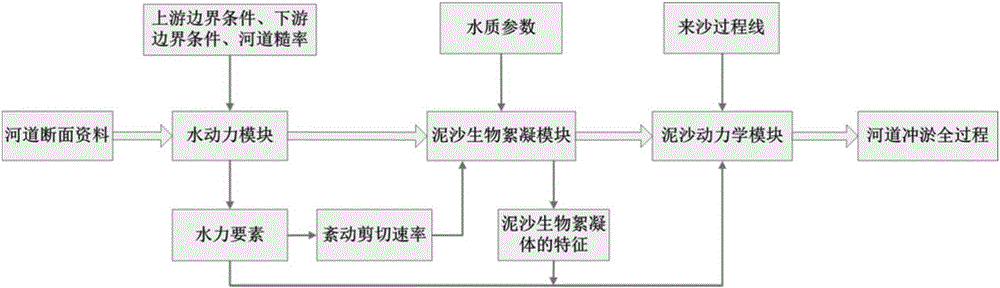
[0040] The general idea of ​​the water-sediment dynamics model system based on bioflocculation is as follows: figure 1 shown. It mainly includes the following parts:

[0041] (1) Given the initial channel cross-section data, using the upstream boundary conditions, downstream boundary conditions and channel roughness, the size and change of the hydraulic elements along the channel are calculated through the hydrodynamic module, and the water surface line (water level) distribution and water depth distribution are obtained. , Velocity distribution, Flow distribution.

[0042] Among them, the channel cross-section data include the position and elevation of each measuring point in the river channel cross-section. The upstream boundary conditions may include upstream hydrographs and upstream hydrographs, and in this embodiment only upstream hydrographs are used. The downstream boundary conditi...

Embodiment 2

[0049] Model equations and principles

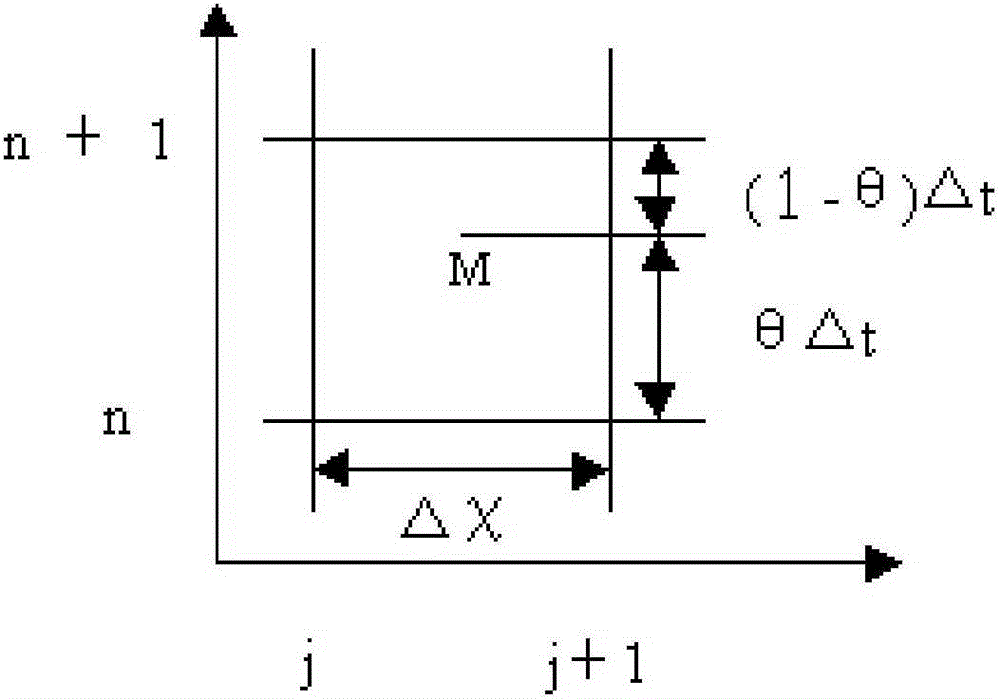
[0050] 1. Water flow equation

[0051] Open channel flow is often considered as one-dimensional flow, so one-dimensional flow continuity equation and equation of motion are the governing equations of open channel flow problems. The purpose of using one-dimensional unsteady flow mathematical model calculation is to correctly estimate the magnitude and change of hydraulic elements (such as water level, flow rate, flow velocity, etc.) when these hydraulic phenomena occur.

[0052] The governing equations for one-dimensional unsteady flow are Saint-Venant equations:

[0053] ∂ Q ∂ x + ∂ A ∂ t = q - - - ( 1 )

[0054] ...

Embodiment 3

[0066] Main parameters and corresponding biofilm impact corrections

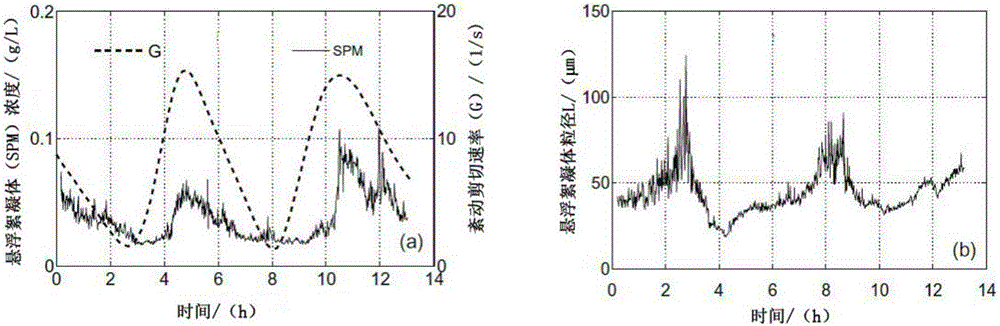
[0067] 1 Calculation of erosion and deposition of suspended sediment

[0068] The sand-carrying force of suspended sediment is calculated by introducing Zhang Ruijin’s formula of carrying sand-carrying force:

[0069] S * = K ( U 3 gRω ) m - - - ( 5 )

[0070] In the formula, S * U is the average velocity of the section; R is the hydraulic radius. For wide and shallow channels, R in formula (5) can be replaced by the average water depth H; K (=0.245) and m (=0.92) are respectively Sand force coefficient and index. ω in formulas (3)-(5) is applicable to pure sediment particles or sediment biological flocs, or their c...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention provides a water and sand dynamic simulation method based on bioflocculation. The method includes the following steps that A, initial river way section data are given, the water power factor of each section is calculated according to the upstream boundary condition, the downstream boundary condition and the river way roughness rate, and then water power factor distribution of a whole river way is acquired; B, the water power factors are used for calculating the water turbulent fluctuation shearing rate and then feature data of suspended sand bioflocculation bodies are calculated through the water turbulent fluctuation shearing rate and measured water quality parameters; C, an upstream sand inflow hydrograph, the water power factors of all the sections and the features of the suspended sand bioflocculation bodies are used for calculating scouring sedimentation and moving transportation of the sand bioflocculation bodies of the corresponding sections, and the river way section condition generated after silt is washed away is given; and D, the step A, the step B and the step C are repeated at corresponding time nodes till calculation of a whole period is finished, the whole river way silt washing-away process is simulated, and the evolvement rule of the river way is provided.

Description

technical field [0001] The invention relates to the technical field of water conservancy engineering, in particular to the field of water and sand dynamics of biological flocculation. Background technique [0002] my country's rivers have high sediment content and large amount of sediment transport. Sediment transport has an important impact on river bed evolution and water conservancy project construction. Therefore, sediment research has always been highly valued in my country, and a relatively mature research system has been formed. . However, traditional sediment research takes clean sediment as the research object, which is mainly produced by rock weathering, and its surface characteristics, particle characteristics and population characteristics are all based on rock particles, ignoring the influence of microorganisms. [0003] In recent years, with the rapid development of social economy, a large amount of production and domestic water discharge, point source and non-...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): E02B1/02
Inventor 赵慧明曹文洪汤立群王崇浩王玉海刘大滨郭传胜秦俊桃
Owner CHINA INST OF WATER RESOURCES & HYDROPOWER RES
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com