Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Space tangent vector-based power system static-state voltage stability domain boundary approximation method

A technology for static voltage stability and power systems, applied in electrical components, circuit devices, AC network circuits, etc., can solve the problem of difficult formation of hyperplane analytic formula, difficult hyperplane analytic formula to describe the boundary of voltage stability domain, complex topological characteristics of the boundary, etc. question

Active Publication Date: 2018-09-04
NORTHEAST DIANLI UNIVERSITY
View PDF3 Cites 2 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

Although this method improves the calculation accuracy, it is difficult to form a unified hyperplane analytical formula, which seriously affects the evaluation efficiency of the static voltage stability of the system.
[0006] 2) The hyperplane approximation method no longer constructs the SVSR by searching the saddle node bifurcation points point by point, but constructs the SVSR by approximating the local boundary of the static voltage stability domain, which significantly improves the construction of the approximate boundary of the SVSR compared with the fitting method efficiency, but this method has high conservatism
[0007] Due to the extremely complex topological properties of the boundary, it is difficult to use a unified hyperplane analytical formula to describe the boundary of the voltage stability domain
Therefore, the construction of SVSR still lacks a general method that is efficient and ensures good accuracy

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Space tangent vector-based power system static-state voltage stability domain boundary approximation method
  • Space tangent vector-based power system static-state voltage stability domain boundary approximation method
  • Space tangent vector-based power system static-state voltage stability domain boundary approximation method

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

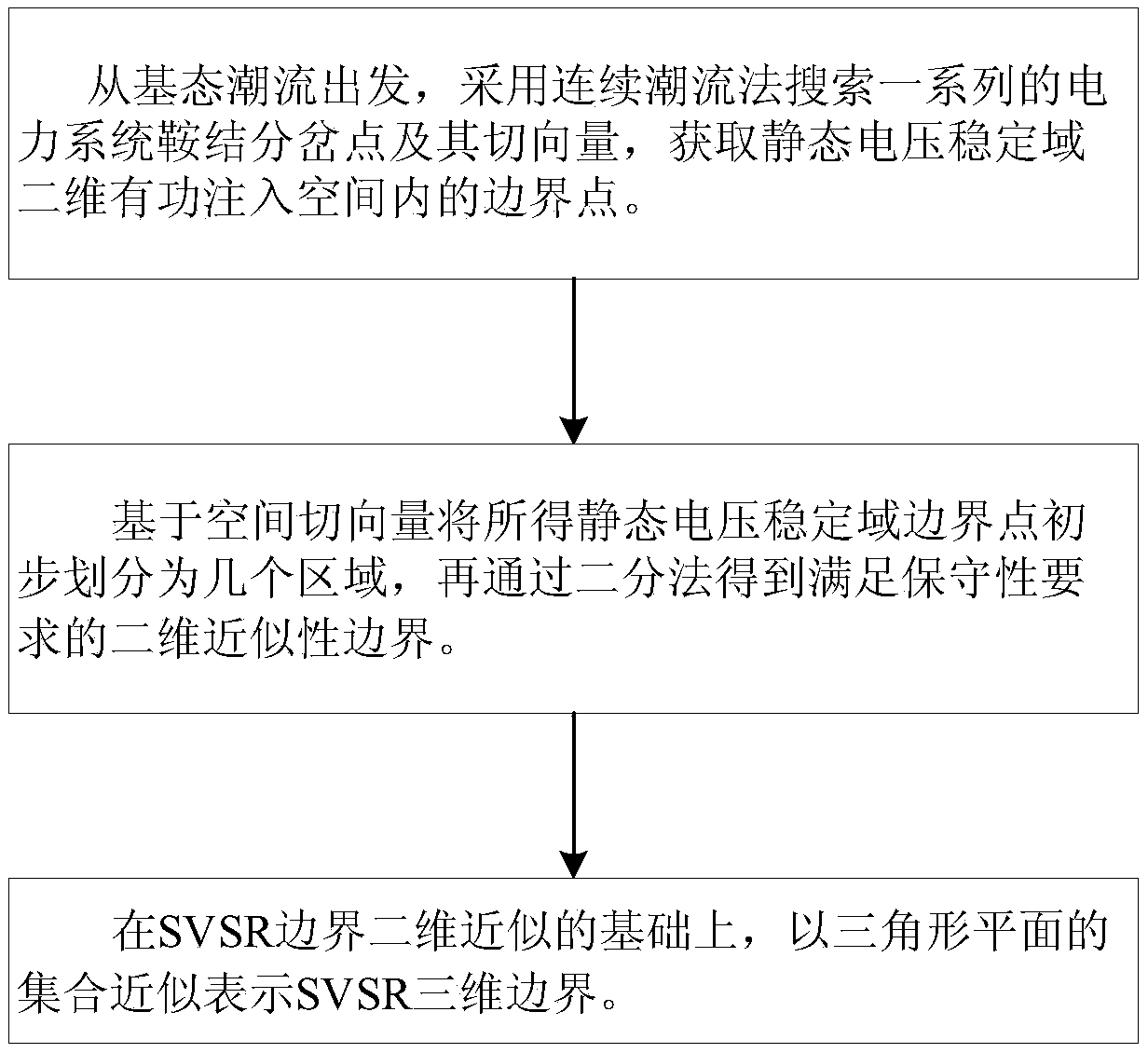
[0072] The embodiment of the present invention provides a method for approximating the boundary of the static voltage stability region of a power system based on a space tangent vector, such as figure 1 As shown, the method includes the following steps:

[0073] 101: Using the ground state power flow as the starting point, the continuous power flow method is used to search for a series of power system saddle junction bifurcation points and their tangent vectors to obtain the boundary point in the two-dimensional active power injection space of the static voltage stability domain, that is, the SNB point;

[0074] 102: Obtain a two-dimensional approximate boundary that meets the requirement of a certain distance error threshold;

[0075] Based on the space tangent vector, the static voltage stability domain formed by the obtained boundary points is preliminarily divided into several regions, such as figure 2 As shown, the static voltage stability domain can be determined by R 1 With R...

Embodiment 2

[0080] The following combined with the specific calculation formula, attached image 3 , Figure 4 , Figure 5 as well as Image 6 The solution in Example 1 is further introduced, as detailed in the following description:

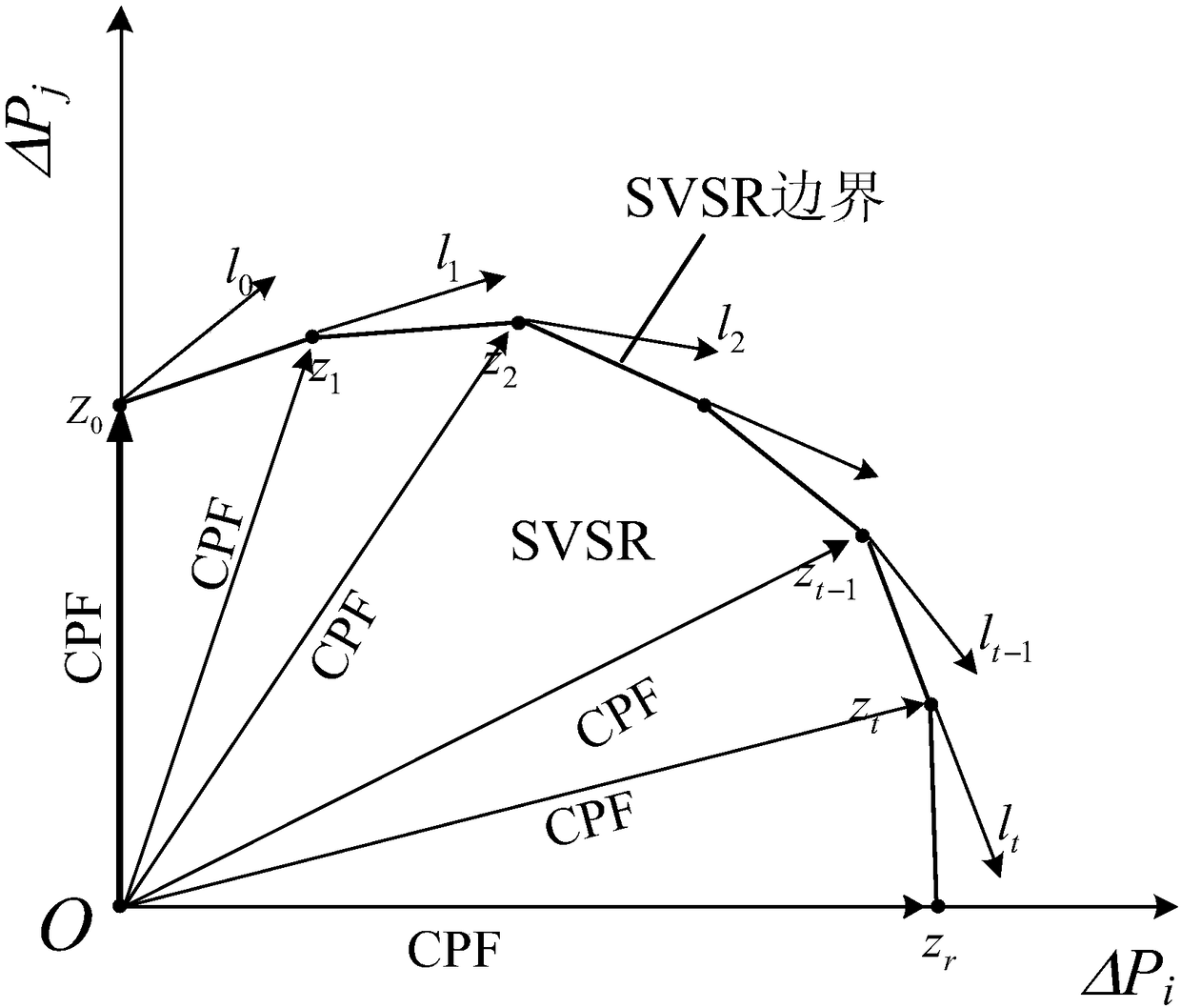
[0081] 201: Taking the base state power flow as the starting point, repeatedly calling the continuous power flow to obtain a series of SNB points under different power growth directions and the tangent vector and load margin λ corresponding to the SNB points;

[0082] Among them, the SNB point when only node j has active power injection is denoted as z 0 , Along the direction in which the active power injection of the i-node increases gradually, denote the obtained SNB points as z 1 ,z 2 …z r (r is divided by z 0 The number of SNB points obtained outside). The obtained SNB point and its tangent vector are mapped to the two-dimensional active power injection space with the active power injection of nodes i and j as the coordinate axis.

[0083] 202: Using the metho...

Embodiment 3

[0149] The following combines specific examples, Figure 7 , Picture 8 , Picture 9 And Table 1 verifies the feasibility of the schemes in Examples 1 and 2, as detailed in the following description:

[0150] This example is to search for the approximate boundary of the two-dimensional SVSR of the WECC 3-machine 9-node system as an example to verify the effectiveness of the method. The WECC 3-machine 9-node test system is as follows Figure 7 Shown.

[0151] With load nodes 5 and 9 as the key nodes for voltage stability, the active power injection of nodes 5 and 9 is selected as the coordinate axis, and the SVSR is constructed using this method in the two-dimensional active power injection space.

[0152] Set different power growth directions, repeatedly call the continuous power flow method to search to obtain a series of SNB points and their tangent vectors, such as Picture 8 Points 1, 2...13 are shown. The coordinates of the SNB point and its tangent vector in the two-dimensional...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a space tangent vector-based power system static-state voltage stability domain boundary approximation method. The method comprises the following steps of taking ground state power flow as the starting point, adopting a continuous power flow method to search a series of power system saddle-node bifurcation points and tangent vectors thereof, and obtaining boundary points inan active power injection space of a static-state voltage stable domain, namely the saddle-node bifurcation points; based on the space tangent vectors, primarily dividing the static-state voltage stable domain formed by the boundary points into several regions, and calculating the distances from the boundary points to corresponding line sections through a method of bisection, and obtaining a two-dimensional approximation boundary which satisfies certain distance error threshold value requirements; and on the basis of the two-dimensional approximation boundary, establishing a three-dimensionalapproximation boundary according to a triangular establishment principle, then obtaining a triangular approximate plane equation, calculating the maximum distance error from all SNB points in the approximate region corresponding to S triangular approximate planes to the plane, and when the error threshold value is satisfied, completing approximation to obtain a three-dimensional SVSR triangular approximate boundary.

Description

Technical field [0001] The present invention relates to the field of static voltage stability domain, and in particular to a method for approximating the boundary of the static voltage stability domain of a power system based on a space tangent vector. Background technique [0002] Since the 1970s, large-scale voltage collapse accidents have occurred in many countries in the world, causing huge economic losses. Since then, the issue of power grid voltage security and stability has attracted great attention from the power industry and academia, and a lot of research has been carried out. The static voltage stability region (SVSR) research has focused on the security and stability of power systems. Occupies a pivotal position in the field of sexual analysis. [0003] The static voltage stability domain is the area formed by and only all the system operating points that satisfy the static voltage stability. The key to obtaining SVSR is the construction of the SVSR boundary. Due to t...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): H02J3/00
CPCH02J3/00H02J2203/20
Inventor 姜涛李雪陈厚合李晓辉王长江李国庆张儒峰张嵩
Owner NORTHEAST DIANLI UNIVERSITY
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com