Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

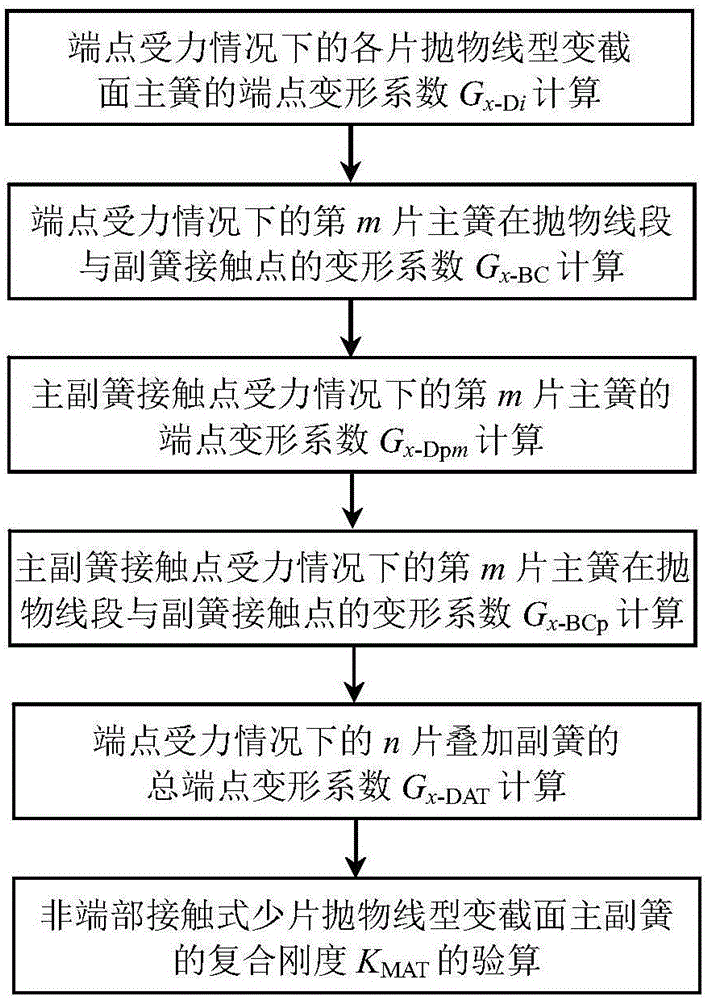
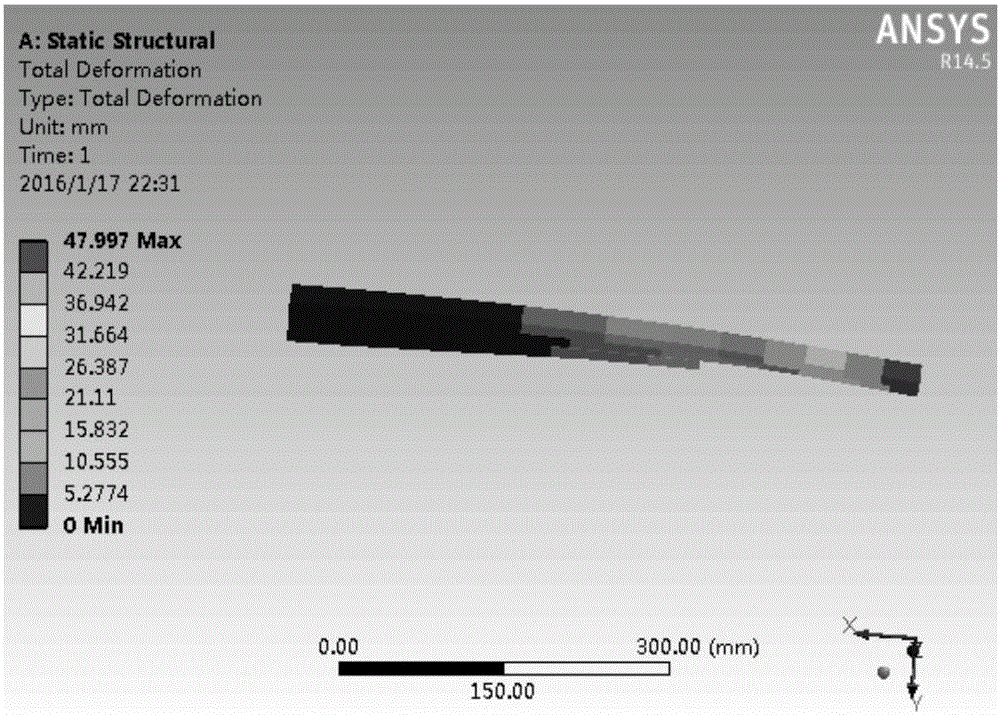
Checking computation method for composite stiffness of non-end contact type few-leaf parabolic main and auxiliary spring

A composite stiffness and parabolic technology, which is applied in the field of vehicle suspension leaf springs, can solve the problem that the non-end contact type few-piece parabolic variable cross-section main and auxiliary springs cannot meet the requirements of composite stiffness checking, the lengths of the main and auxiliary springs are not equal, and the main and auxiliary springs are not equal in length. Problems such as complex analysis and calculation of the internal force of the auxiliary spring

Inactive Publication Date: 2016-09-21
周长城
View PDF0 Cites 12 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

However, due to the non-isomorphic straight section of the end of the main spring, the unequal length of the main and auxiliary springs, the deformation and internal force analysis and calculation of the main and auxiliary springs are very complicated, therefore, for the non-end contact type few-piece parabolic variable-section main and auxiliary The composite stiffness of the spring has not been given an accurate and reliable checking method before.
Although some people have previously given the design and calculation methods of a few variable-section leaf springs, for example, Peng Mo and Gao Jun once proposed a variable-section leaf spring in "Automotive Engineering", 1992 (Volume 14) No. 3 The design and calculation method is mainly for the design and calculation of the small-piece parabolic variable-section leaf spring with the same structure at the end. Requirements for its composite stiffness checking calculation
At present, most engineering designers ignore the influence of unequal lengths of the main and auxiliary springs, and directly superimpose the stiffness of the main spring and the auxiliary spring for approximate checking calculations, which cannot satisfy the precise design of the non-end contact type few-piece parabolic variable-section main and auxiliary springs Requirements for accurate calculation of its composite stiffness

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Checking computation method for composite stiffness of non-end contact type few-leaf parabolic main and auxiliary spring
  • Checking computation method for composite stiffness of non-end contact type few-leaf parabolic main and auxiliary spring
  • Checking computation method for composite stiffness of non-end contact type few-leaf parabolic main and auxiliary spring

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

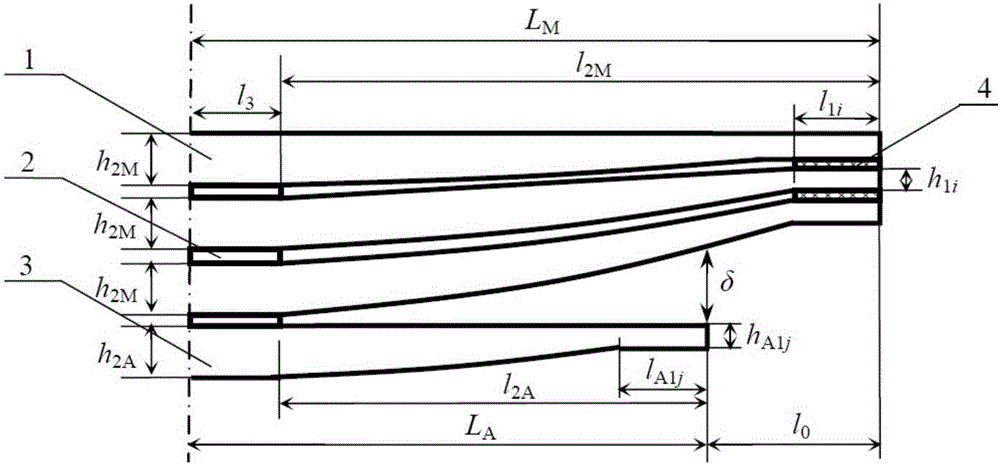
[0035] Example 1: The width of a non-end contact type few parabolic variable section primary and secondary springs is b = 60mm, the modulus of elasticity E = 200GPa, half of the installation distance l 3 =55mm; Among them, the number of main reeds m=2, half the length L of each main spring M =575mm, the distance l from the root of the parabolic segment of the main spring to the end point of the spring 2M =L M -l 3 =520mm, the root thickness h of each main spring 2M =11mm; the thickness h of the straight section at the end of the first main spring 11 =7mm, the thickness ratio of the parabolic segment of the first main spring to β 1 = h 11 / h 2M =0.64, the thickness h of the straight section at the end of the second main spring 12 = 6mm, the thickness ratio of the parabolic segment of the second main spring to β 2 = 0.55. Number of secondary reeds n=1, half length L of secondary reeds A =375mm, the horizontal distance l between the auxiliary spring contact and the main...

Embodiment 2

[0061] Embodiment 2: The width b=60mm of a non-end contact type few-piece parabolic variable-section main spring, half of the installation distance l 3 = 60mm, modulus of elasticity E = 200GPa; among them, the number of main reeds m = 2, half the length of the main spring L M =600mm, the distance l from the root of the parabolic segment of the main spring to the end point of the main spring 2M =L M -l 3 =540mm, the thickness h of the straight section at the root of each main spring 2M =12mm; the thickness h of the straight section at the end of the first main spring 11 = 8mm, the thickness ratio of the parabolic segment of the first main spring to β 1 = h 11 / h 2M =0.67; the thickness h of the straight section at the end of the second main spring 12 =7mm, the thickness ratio of the parabolic segment of the second main spring to β 2 = h 12 / h 2M = 0.58. The number of secondary reeds n=1, half the length L of the secondary reed A =410mm, the distance l from the root ...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

PropertyMeasurementUnit
Half lengthaaaaaaaaaa
Widthaaaaaaaaaa
Elastic modulusaaaaaaaaaa
Login to View More

Abstract

Belonging to the technical field of suspension steel plate springs, the invention relates to a checking computation method for the composite stiffness of non-end contact type few-leaf parabolic main and auxiliary spring. According to the structural parameter and elastic modulus of each main spring leaf and each auxiliary spring leaf in the non-end contact type few-leaf parabolic tapered main and auxiliary spring, precise checking computation of the composite stiffness of the non-end contact type few-leaf parabolic tapered main and auxiliary spring carried out. By means of examples and ANSYS simulation verification, the checking computation method for composite stiffness of the non-end contact type few-leaf parabolic tapered main and auxiliary spring provided by the invention is correct, thus providing a reliable checking computation method for composite stiffness checking computation. The method can acquire an accurate and reliable composite stiffness checking calculation value of the non-end contact type few-leaf parabolic tapered main and auxiliary spring, ensures that the product can meet a design required value of a suspension for composite stiffness, thus enhancing the product design level and performance and vehicle travel riding comfort. At the same time, the method also can reduce the design and experiment test cost, and speeds up product development rate.

Description

technical field [0001] The invention relates to a vehicle suspension leaf spring, in particular to a method for checking and calculating the composite stiffness of a non-end contact type few-piece parabolic primary and secondary spring. Background technique [0002] In order to meet the design requirements of variable stiffness of the vehicle suspension under different loads, a small number of variable cross-section main and auxiliary springs are used. There is a certain gap between the auxiliary spring contacts and the main spring to ensure that when it is greater than the auxiliary spring After the active load, the main and auxiliary springs work together to meet the design requirements of composite stiffness. The force of the first main spring of the few variable cross-section main and auxiliary springs is complex, not only bears vertical loads, but also bears torsional loads and longitudinal loads. Therefore, the end straight section of the first main spring is actually ...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G06F17/50
CPCG06F30/17G06F30/23
Inventor 于曰伟王炳超周长城汪晓邵明磊袁光明赵雷雷
Owner 周长城
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com