Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

A Method for Seismic Data Reconstruction

A technology of seismic data and coefficients, applied in the fields of seismology, seismic signal processing, geophysical measurement, etc., can solve the problems of low accuracy of reconstruction data and slow calculation speed of seismic data reconstruction, achieve fast calculation speed, high calculation accuracy, improve The effect of computational efficiency

Active Publication Date: 2021-05-11
CHINA NAT OFFSHORE OIL CORP +1
View PDF6 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0004] The purpose of the present invention is to provide a seismic data reconstruction method to solve the problems of slow calculation speed and low precision of reconstruction data in current seismic data reconstruction

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • A Method for Seismic Data Reconstruction
  • A Method for Seismic Data Reconstruction
  • A Method for Seismic Data Reconstruction

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

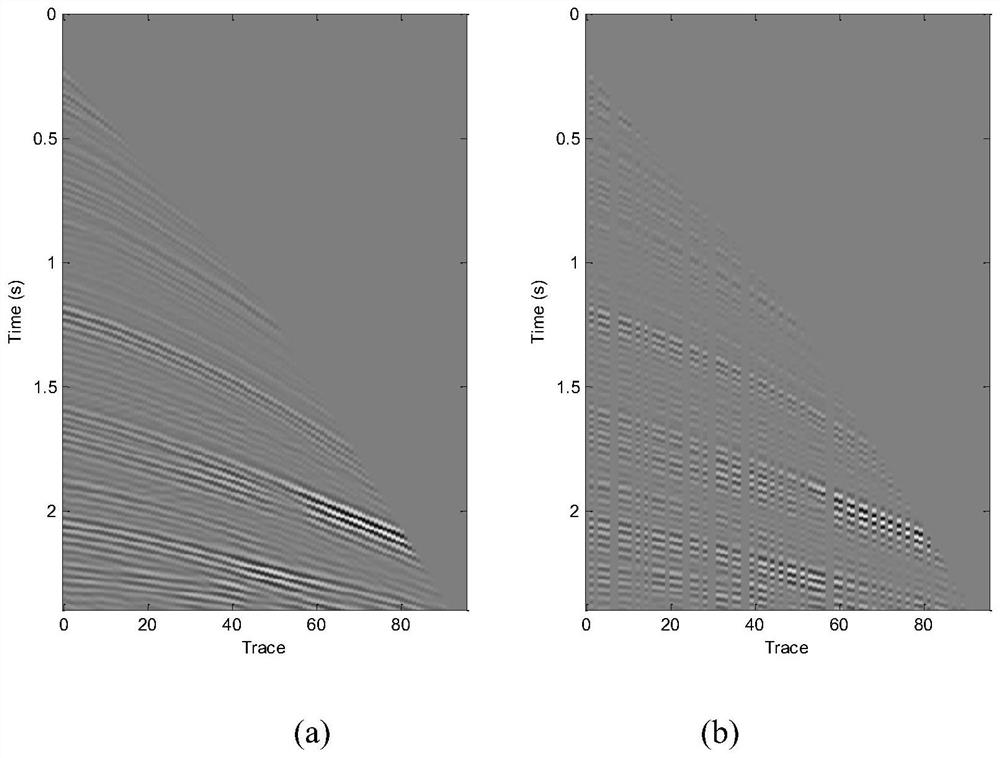
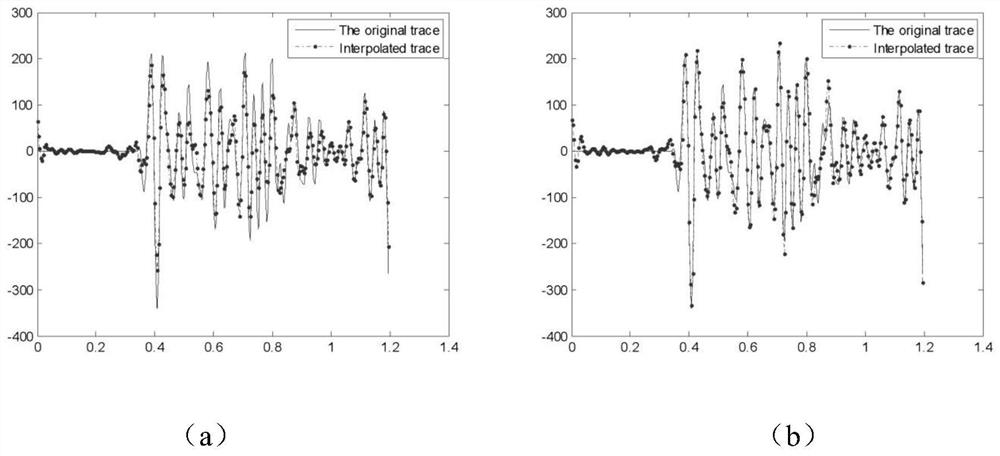
[0050] Embodiment 1 provides a seismic data reconstruction method, the seismic data reconstruction method includes the following steps:

[0051] Step A: Obtain the seismic data to be reconstructed and its acquisition matrix;

[0052] Here, the seismic data to be reconstructed may be the seismic data obtained after the preprocessing of the collected original seismic data, or the data processed by stacking, migration, etc. First, the seismic data to be reconstructed and the acquisition matrix corresponding to the seismic data need to be obtained.

[0053] Step B: perform block processing on seismic data and acquisition matrix;

[0054]Here, the block method adopted may be overlapping blocks or non-overlapping blocks. The seismic data to be reconstructed and the acquisition matrix adopt the same block method.

[0055] Step C: Establishing a sparse inversion model regularized by the hyperbolic tangent function of the seismic data of each small block and its corresponding acquis...

Embodiment 2

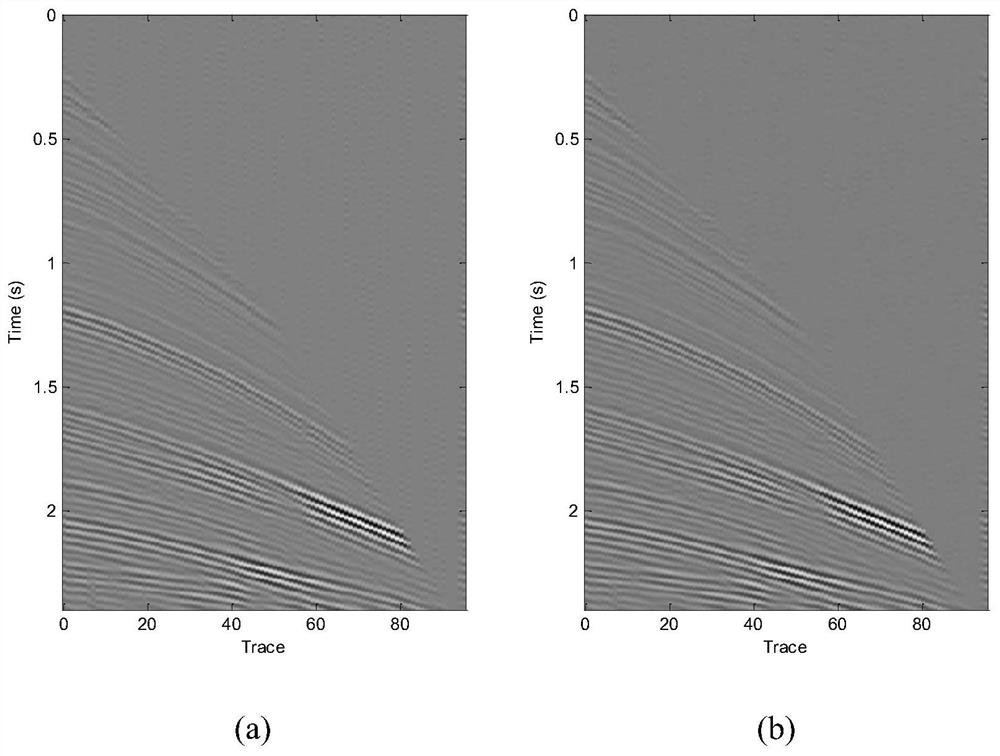
[0082] Embodiment 2 provides a seismic data reconstruction method. On the basis of Embodiment 1, Embodiment 2 makes another equivalent expression for Step D of Embodiment 1:

[0083] Step D uses the modified Newton-projection method to obtain the optimal solution of the sparse inversion model, and the specific steps include:

[0084] Step D21: Set the number of iterations IterN, initial hyperparameter σ 0 and the termination iteration hyperparameter σ IterN , and take the observed seismic data as the initial solution d 0 ;

[0085] Step D22: Calculate the coefficient x of the iterative solution of the current k-th iteration in the transform domain k =Fd k , where k represents the iteration number; F represents the Fourier transform; when k=0, represents the initial iteration, d k Represents the seismic data reconstructed at the kth iteration;

[0086] Compute the modified Newton direction for the current k-th iteration:

[0087]

[0088] In the formula, σ k is the h...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a seismic data reconstruction method, comprising the following steps: acquiring seismic data to be reconstructed and its acquisition matrix; performing block processing on the seismic data and acquisition matrix; establishing each small block of seismic data and its corresponding acquisition The sparse inversion model regularized by the hyperbolic tangent function of the matrix; the optimal solution of the sparse inversion model is obtained through each small block of data, and the reconstructed seismic data is obtained; data reconstruction is performed on all block data, and the All reconstructed data are stitched into a complete reconstruction. The seismic data reconstruction method disclosed by the present invention has fast calculation speed and high precision, and is not only applicable to two-dimensional data, but also applicable to three-dimensional data and five-dimensional data; not only applicable to pre-stack seismic data, but also applicable to post-stack seismic data; not only applicable to It is applicable to land seismic exploration data and also applies to marine seismic exploration data.

Description

technical field [0001] The invention relates to the technical field of seismic data processing, in particular to a method for reconstructing seismic data with high timeliness and high precision. Background technique [0002] Seismic exploration is an important means for humans to obtain underground information, and plays an important role in oil and gas exploration, coal field exploration, environmental and engineering exploration and other fields. In the data acquisition process of seismic exploration, situations such as missing seismic traces, irregular sampling data, and failure to meet the acquisition design scheme and sampling theorem often occur. The reasons for this include bad tracks, the influence of surface obstacles, or the elimination of trace records with strong noise during preprocessing, etc., which will lead to data loss, and data loss will lead to sampling aliases, causing artifacts to appear in seismic traces, thus affecting In the process of seismic data ...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Patents(China)
IPC IPC(8): G01V1/36G01V1/28
CPCG01V1/282G01V1/36G01V2210/48
Inventor 黄小刚曹静杰张金淼杜向东李维新朱振宇翁斌刘志斌张益明丁继才薛东川王小六
Owner CHINA NAT OFFSHORE OIL CORP
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com