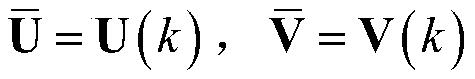Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

A Low Complexity Robust Subspace Estimation Method

A space estimation, low-complexity technique, applied in the field of robust subspace estimation, capable of solving low-complexity problems

Active Publication Date: 2019-06-18
UNIV OF ELECTRONICS SCI & TECH OF CHINA
View PDF4 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0004] The purpose of the present invention is to overcome the deficiencies in the prior art, solve the subspace from the viewpoint of low-rank matrix decomposition, propose a kind of low-complexity robust subspace estimation method, specifically an efficient iterative reweighting algorithm, the method Does not require any step size search, each iteration is an optimal closed-form solution to a subproblem

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • A Low Complexity Robust Subspace Estimation Method
  • A Low Complexity Robust Subspace Estimation Method
  • A Low Complexity Robust Subspace Estimation Method

Examples

Experimental program
Comparison scheme
Effect test

example 1

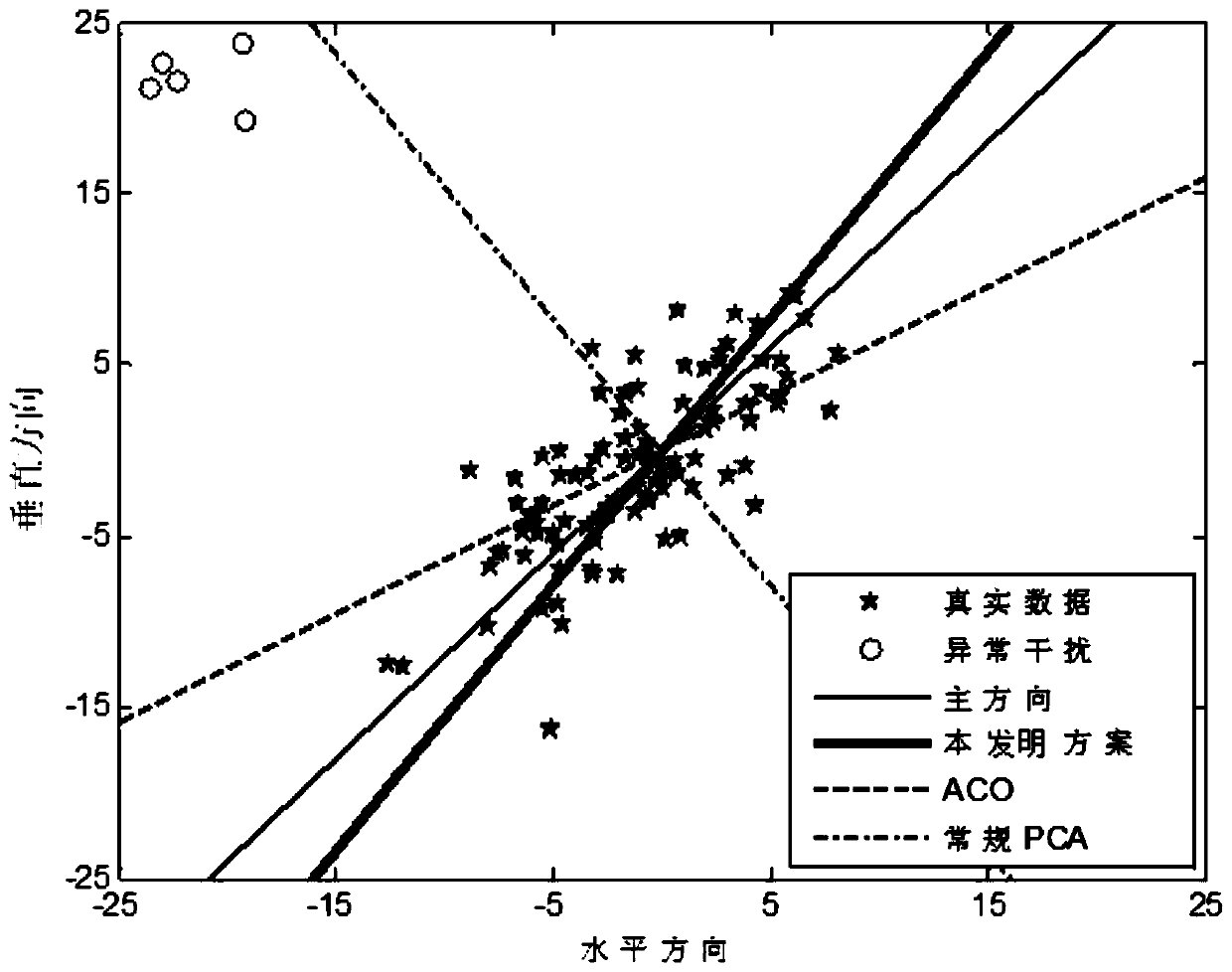
[0056] Example 1 The following uses robust (real number) data dimensionality reduction as an example to elaborate on the specific application of the robust subspace method proposed in the present invention. First, we generate 150 two-dimensional real data samples (Such as figure 1 The five-pointed star in), and obey the following Gaussian distribution:

[0057]

[0058] among them, Represents normal distribution, 0 2 (2-dimensional column vector) is the mean value, Indicates its variance. At the same time, 5 abnormal data points are generated, o 1 ,...,O 5 ,Such as figure 1 The circle point in the upper left corner. At the same time, 5 random abnormal data points obey the following Gaussian distribution:

[0059]

[0060] In this example, define Y in Algorithm 1 as At the same time, let ε=1, γ=0.5, and p=1. The initialization of U(0) and V(0) are the results obtained by conventional PCA (Singular Value Decomposition) respectively, and suppose the maximum number of iteration...

example 2

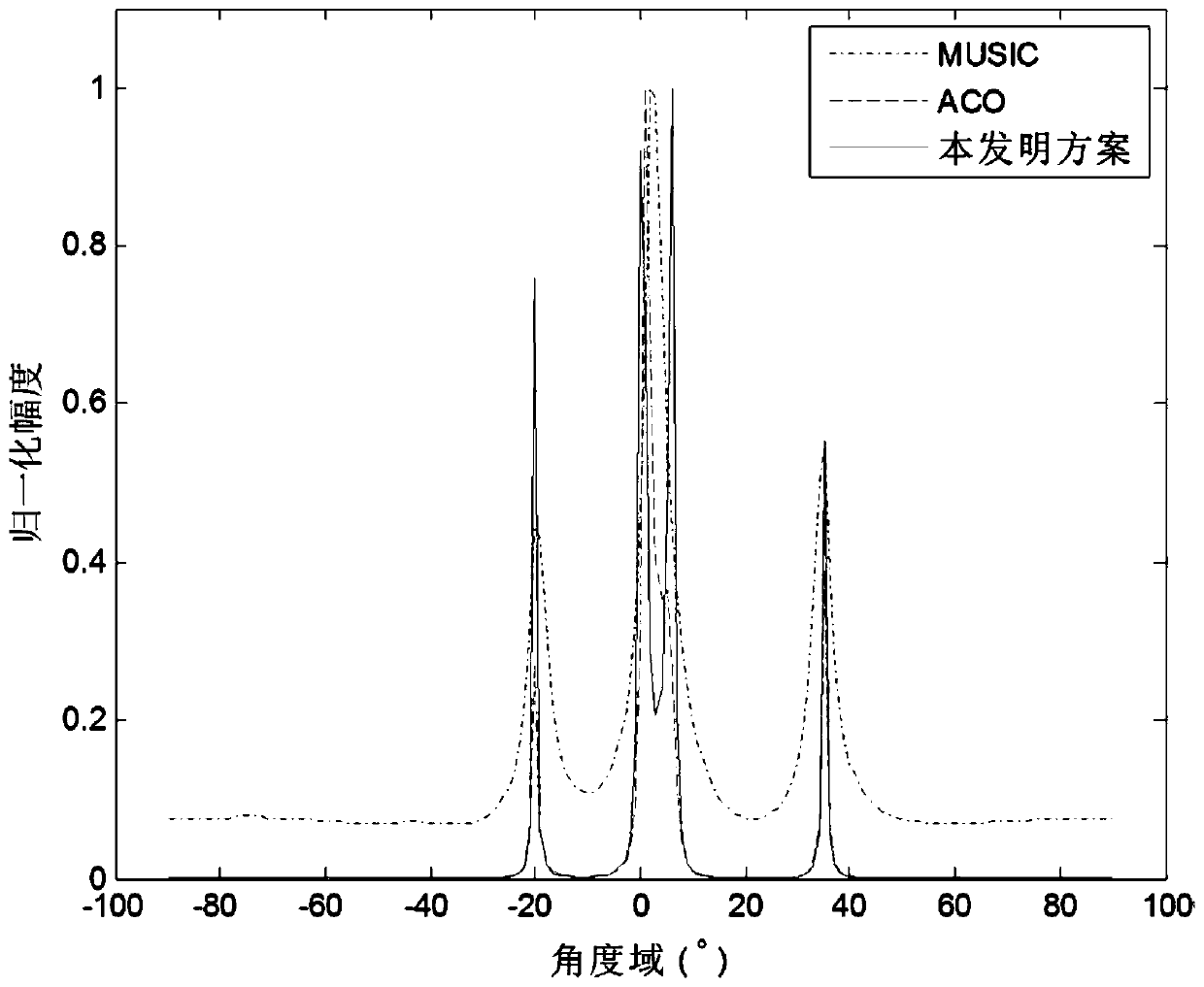
[0061] Example 2 The following describes the application of the solution of the present invention in the positioning of the source of the sensor array. Suppose K direction of arrival angles are θ=[θ 1 ,...,θ K ]'S narrowband source is located at M(M> K) far field of uniform linear array (ULA) structure, then the receiving signal model of N snapshot arrays is

[0062] x(t)=A(θ)s(t)+n(t), t=1,...,N

[0063] In the formula, s(t) is the zero mean signal vector, Is the array flow pattern vector, a(θ k ) Is an M-dimensional column vector and its elements are m=1,...,M,θ k Ε(-π / 2,π / 2), λ and d respectively represent the signal wavelength and the distance between array elements. In this case, set the number of antennas M=8, the number of sources K=4, the number of snapshots N=100, and the source position θ=[-20°,0°,6°,35°] T , And d=λ / 2; the superimposed noise is Gaussian mixed impulse noise, and the signal-to-noise ratio is -5dB. In order to make the solution of the present invention s...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention belongs to the field of signal processing and communication, and relates to a low-complexity robust subspace estimation method. In order to reduce the computational complexity, the method of the present invention mainly decomposes the unknown variable into a series of block variables and selects the block update rule, and then finds an appropriate upper bound function corresponding to the original objective function to solve the current problem. The method is based on the gradient descent of inexact block coordinates. For each sub-problem, by finding a reliable approximate solution and only through the four arithmetic operations of vectors, the computational overhead of the highly complex one-dimensional line search optimization problem is avoided. At the same time, by introducing a new robust function with adjustable parameters, it not only avoids the calculation problems caused by the non-smoothness of the cost function, but also improves the robustness to outliers or impulse noise.

Description

Technical field [0001] The invention belongs to the field of signal processing and communication, and relates to a low-complexity robust subspace estimation method. Background technique [0002] The subspace method has important applications in signal processing, communication and computer vision. Its main function is to represent high-dimensional data by selecting low-dimensional uncorrelated variable components to achieve the effect of noise reduction and dimensionality reduction. Traditional subspace methods are mostly based on the Euclidean norm (l 2 ) Low-rank matrix decomposition in space, such as PCA (principal component analysis) method based on truncated singular value or eigen decomposition; "Multiple emitter location and signal parameter estimation". As we all know, this tradition is based on l 2 The norm method cannot be applied to impulse noise, because l 2 The norm is optimal only under Gaussian background noise. In fact, in many practical applications, in addition...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Patents(China)
IPC IPC(8): G01S5/00G06K9/62
CPCG01S5/00G06F18/2135
Inventor 何振清史治平闫秦怀
Owner UNIV OF ELECTRONICS SCI & TECH OF CHINA
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com