Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

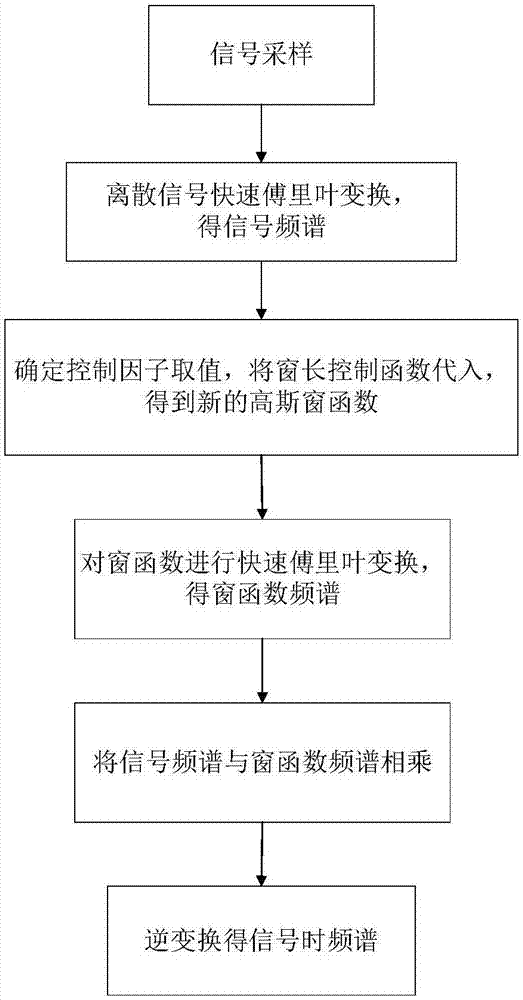
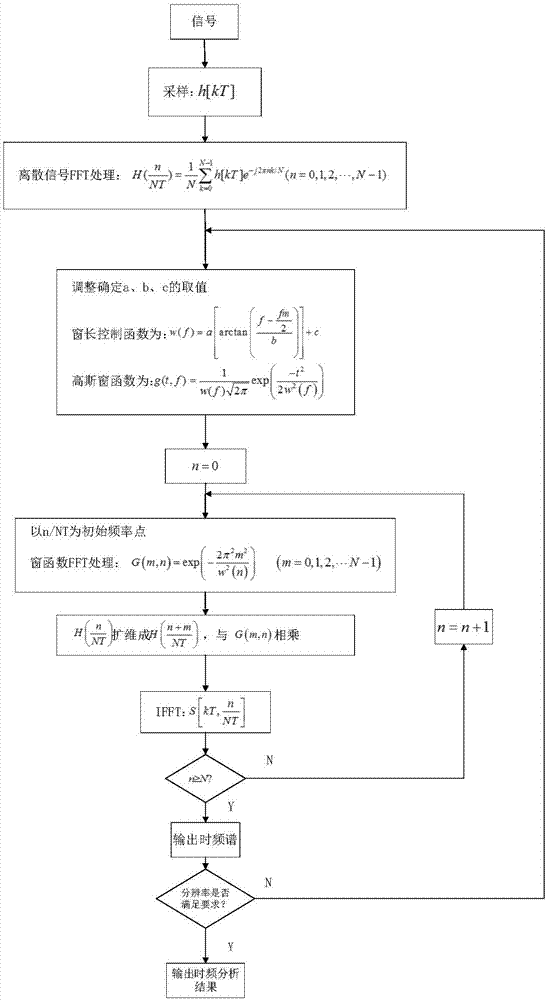
Limited-window length time frequency analysis method based on improved S-transformation

A time-frequency analysis and window length technology, applied in spectrum analysis/Fourier analysis and other directions to achieve high-resolution time-frequency analysis and strong flexibility

Active Publication Date: 2017-11-28
NANJING UNIV OF SCI & TECH
View PDF5 Cites 9 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0007] It can be seen from the above that the existing time-frequency analysis methods still have deficiencies and need to be further improved to achieve high-precision time-frequency analysis

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Limited-window length time frequency analysis method based on improved S-transformation
  • Limited-window length time frequency analysis method based on improved S-transformation
  • Limited-window length time frequency analysis method based on improved S-transformation

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

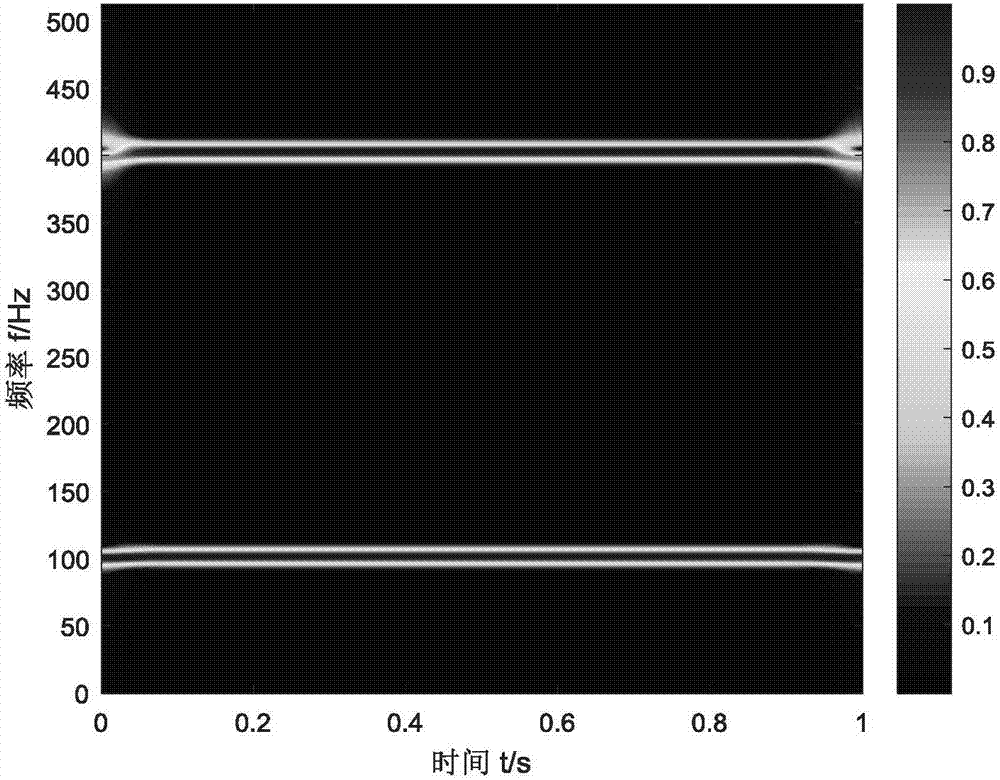
[0044] The simulation signal is the superposition of two sinusoidal signals, the signal frequencies are 100Hz and 400Hz respectively, and the analytical formula is:

[0045] h(t)=sin(200πt)+sin(800πt) t∈[0,1]

[0046] Signal sampling frequency f s =1024Hz, image 3 It is the time-frequency spectrum obtained by the finite-window time-frequency method using arctangent function combined with S-transform. The signal has only two fixed frequency components. For the signal whose frequency does not change with time, only the frequency resolution needs to be considered. Therefore, by adjusting the control factor, take a=1, b=50, c=30 to control the frequency window width at a relatively low In a small range, the time spectrum is guaranteed to have better frequency resolution performance. Depend on image 3 It can be seen that this method can achieve very good frequency resolution.

Embodiment 2

[0048] The simulation signal is a linear frequency modulation signal with a frequency modulation slope of k=400, and the analytical formula is:

[0049]

[0050] Signal sampling frequency f s = 1024Hz, Figure 4 In order to use the arctangent function combined with the S-transform finite window length time-frequency method to analyze the chirp signal. For the chirp signal, the frequency changes greatly, so adjust the control factor, take a=5, b=50, c=50 can be The analysis results shown in the figure are obtained, which solves the problems of signal divergence and poor energy aggregation at high frequencies of the original S-transform, and has good time-frequency performance.

Embodiment 3

[0052] The simulation signal is a non-linear frequency modulation signal whose frequency changes sinusoidally, and the analytical formula is:

[0053] h(t)=e j2π[6cos(10πt)+260t] t∈[0,1]

[0054] Signal sampling frequency f s = 1024Hz, Figure 5 It is the time-frequency diagram obtained after the non-linear frequency modulation signal h(t) is processed by the method of the present invention, because the signal frequency changes sinusoidally with time, so the requirement for time resolution is relatively high, and the minimum value control factor c should be relatively large , adjust the control factor, from the time-frequency results when a=6, b=50, and c=100, you can clearly see the track of frequency change with time, which has good time-frequency analysis performance.

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a limited-window length time frequency analysis method based on improved S-transformation. A mapping based on an arc-tangent function is established between the frequency and the window length, so the window length is changed in an adaptive manner along with the frequency in a certain range and by substituting an improved window function into the S-transformation, high-resolution time frequency analysis is achieved. The method comprises following steps of carrying out signal sampling to obtain discrete sequences; carrying out Fourier transform to acquire signal frequency spectrums; according to characteristics of the signal frequency spectrums and resolution requirements, determining a control factor; substituting an obtained control function into a window function; and allowing the window function to be subjected to the rapid Fourier transform, then be multiplied with the signal spectrums subjected to dimension expansion and then be subjected to Fourier transform so as to obtain a time frequency spectrum. According to the invention, it is achieved that the window length is changed along with the change of the signal frequencies and the change range of the window length is limited; and it is also ensured that each part of the time frequency spectrum has the quite high resolution.

Description

technical field [0001] The invention belongs to a radar signal processing technology, in particular to an improved S-transform limited window length high-precision time-frequency analysis method. Background technique [0002] Non-stationary signals are the most common signals in radar signal processing, and time-frequency analysis is an important tool for analyzing such signals. In order to accurately analyze the local characteristics of the signal, the time-frequency analysis maps the one-dimensional time-domain signal to the two-dimensional time-frequency plane, so as to obtain the time-frequency distribution of the signal. At present, the commonly used time-frequency analysis methods mainly include short-time Fourier transform (STFT), Hilbert-Huang transform (HHT), S-transform (ST), wavelet transform (WT) and so on. [0003] Dennis Gabor proposed the short-time Fourier transform in 1946. The basic idea is to realize the segmental Fourier transform of the signal by adding...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G01R23/16
Inventor 芮义斌严丽萍谢仁宏李鹏郭山红杜禹吕云涛
Owner NANJING UNIV OF SCI & TECH
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com