Near-field signal source positioning method without gridding based on sparse reconstruction

Abstract

The invention discloses a near-field signal source positioning method without gridding based on sparse reconstruction. The method specifically comprises the following steps: acquiring a covariance matrix based on a signal model received by a symmetric uniform linear array, extracting reverse diagonal elements of the covariance matrix, constructing a correlation matrix similar to a Toeplitz matrix,and constructing a target function based on a covariance fitting criterion; estimating a Toeplitz correlation matrix by using positive semidefinite programming, estimating the direction of arrival ofa near-field signal source by using a Prony method based on the Toeplitz correlation matrix, then using a conventional subspace-based method to effectively estimate the range, and using a MUSIC method to estimate the range constructing a correlation matrix similar to a Toeplitz matrix by reverse 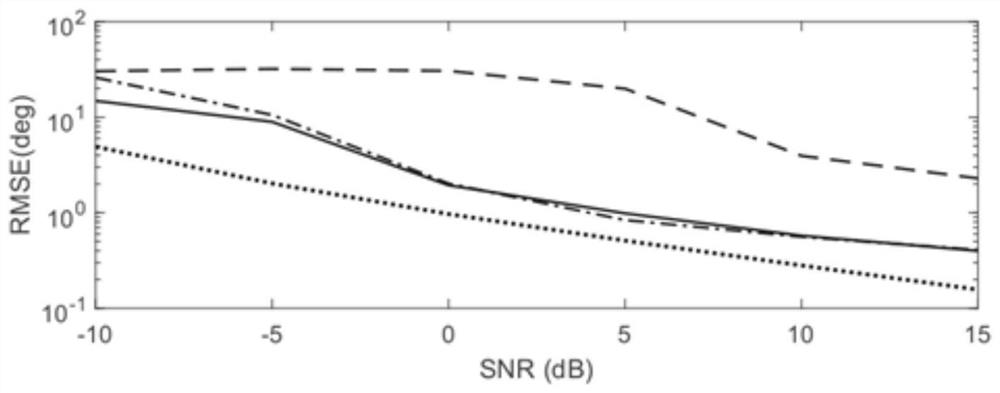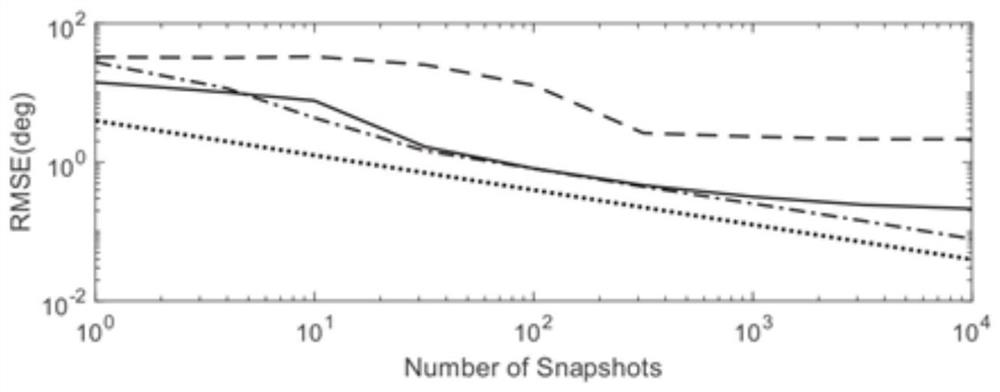diagonal elements of the signal covariance matrix; establishing a convex optimization problem for reconstruction of the correlation matrix, and using the Prony method to estimate the direction of arrival of the near-field signal source based on the recovered correlation matrix, so that the problem ofhigh computational complexity caused by iterative operation is avoided, and the problem of grid mismatch of a traditional gridding method is avoided at the same time.

Description

technical field [0001] The invention belongs to the technical field of array signal processing, and in particular relates to a near-field signal source location method based on sparse reconstruction without gridding. Background technique [0002] The complexity of the near-field problem usually brings a large amount of calculation. How to reduce the calculation amount while ensuring the estimation accuracy has always been the direction of continuous research in the field of near-field source location. The two-dimensional MUSIC algorithm is to extend the traditional one-dimensional MUSIC method to two-dimensional parameter estimation. This method needs to search for two parameters, the direction of arrival and the distance, and the calculation burden is heavy. The maximum likelihood estimation method has good statistical performance, but it usually needs to optimize a highly non-linear cost function, and requires continuous iterations, resulting in a huge amount of calculatio...

AI Technical Summary

Problems solved by technology

The two-dimensional MUSIC algorithm is to extend the traditional one-dimensional MUSIC method to two-dimensional parameter estimation. This method needs to search for two parameters, the direction of arrival and the distance, and the calculation burden is large.

The maximum likelihood estimation method has good statistical performance, but it usually needs to optimize a highly nonlinear cost function, and requires...

Images