Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

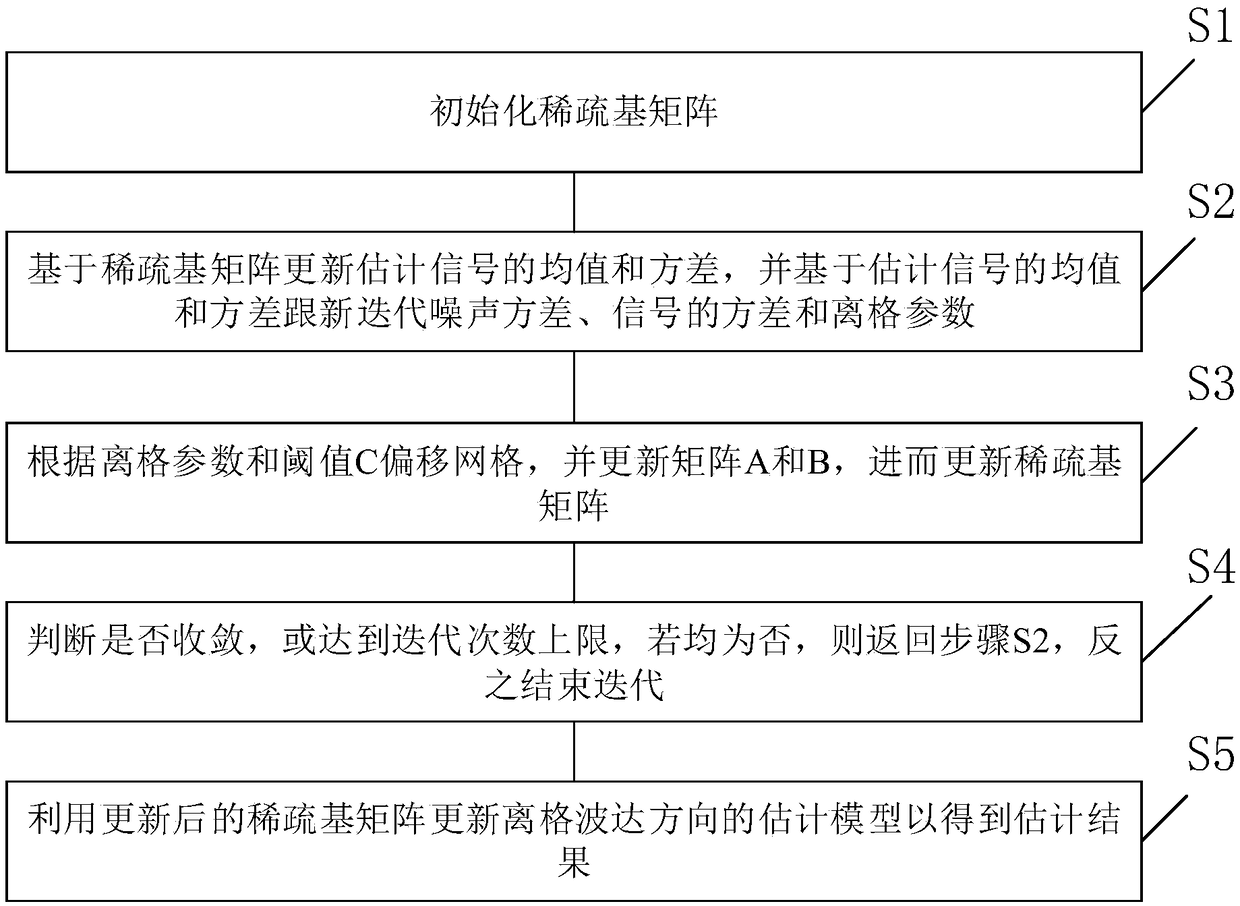
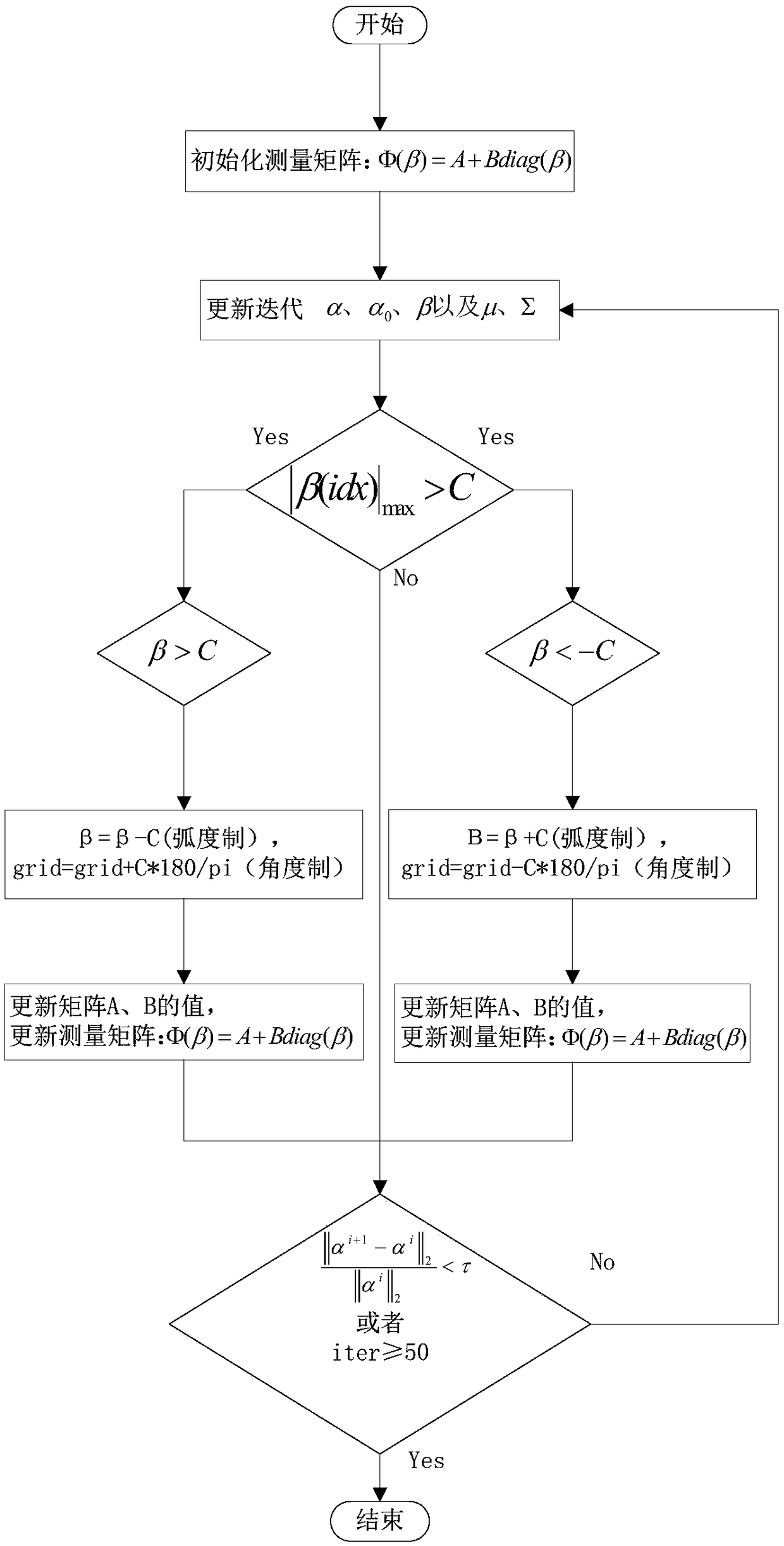
Direction-of-arrival estimation method based on off-grid sparse Bayesian

A direction-of-arrival estimation and sparse Bayesian technology, applied in the field of estimation, to achieve the effect of reducing the amount of calculation, reducing the calculation time, and improving the accuracy

Active Publication Date: 2019-01-01
SHANGHAI NORMAL UNIVERSITY
View PDF5 Cites 12 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

However, in the existing research, there is little discussion on the problem of off-grid DOA estimation in dense signals with high correlation. It is very necessary to use sparse Bayesian to solve the method of off-grid DOA estimation.

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Direction-of-arrival estimation method based on off-grid sparse Bayesian
  • Direction-of-arrival estimation method based on off-grid sparse Bayesian
  • Direction-of-arrival estimation method based on off-grid sparse Bayesian

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

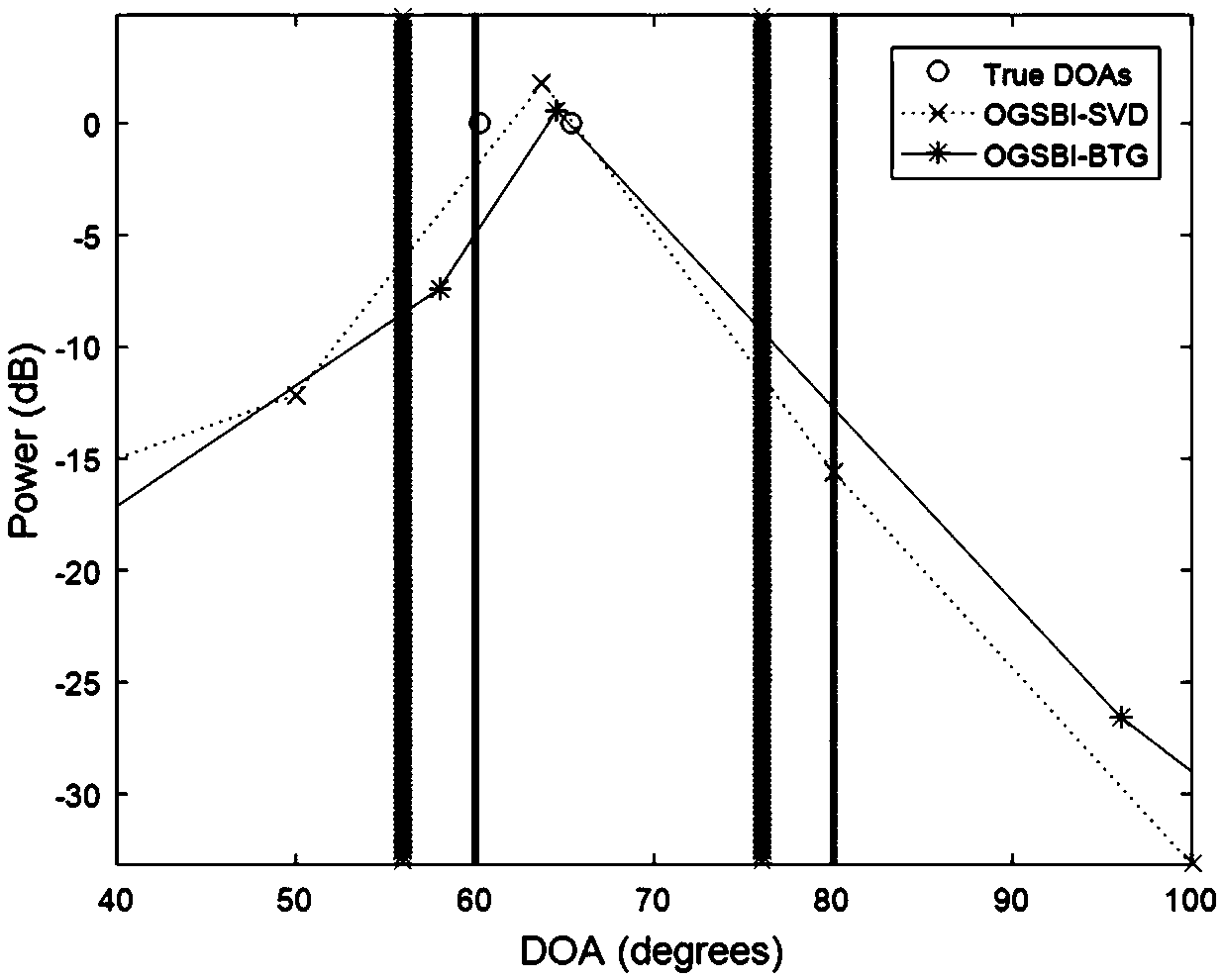
[0128] Embodiment 1: From the perspective of DOA estimation, compare the OGSBI-BTG algorithm of the present invention with the existing OGSBI-SVD algorithm (the thin vertical line is the original grid, and the thick vertical line is the offset grid). The resolution (grid spacing r) is 20, the signal-to-noise ratio SNR is 20, K=2, C=8*pi / 180, and the true DOA of the two signals are 60.2 and 65.4, respectively. available from image 3 It can be seen from the figure that the real DOA of these two signals is between the grid {60~80}, and between the grid {56~76} after migration. In the existing OGSBI-SVD algorithm, one of the DOA values It can basically be estimated that another DOA value cannot be estimated and is outside the grid; in the OGSBI-BTG algorithm of the present invention, one of the DOA estimated values ​​is more accurate than the value estimated by the existing OGSBI-SVD algorithm, And another DOA value can be estimated basically, and these two DOA values ​​are all ...

Embodiment 2

[0129] Embodiment 2: Using the OGSBI-BTG algorithm of the present invention to compare the existing OGSBI-SVD algorithm from the perspective of RMSE. The resolution (grid spacing r) is 20, the signal-to-noise ratio SNR is 0:3:30, K=2, C=8*pi / 180, and the two signal sources are 60.2 and 65.4 respectively. From Figure 4 It can be seen that the RMSE of the OGSBI-BTG algorithm proposed by the present invention is much smaller than that of the existing OGSBI-SVD algorithm when estimating dense DOA in the case of large grid division.

Embodiment 3

[0130]Embodiment 3: From the perspectives of estimated value, β value, and grid division, compare the OGSBI-BTG algorithm of the present invention with the existing OGSBI-SVD algorithm. The resolution (grid spacing r) is 20, the signal-to-noise ratio SNR is 20, K=2, C=8*pi / 180, and the two signal sources are 60.2 and 65.4 respectively.

[0131] Table 1 Comparison table of estimated value, value and grid division between OGSBI-BTG algorithm and existing OGSBI-SVD algorithm

[0132]

[0133]

[0134] It can be seen intuitively from Table 1 that the value of β has been adjusted. Combined with the offset of the overall grid, first set β new The value of is converted into an angle system and the grid value of the corresponding position is the estimated value. The OGSBI-BTG algorithm of the present invention is closer to the real value in estimated value than the existing OGSBI-SVD algorithm.

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention relates to a direction-of-arrival (DOA) estimation method based on off-grid sparse Bayesian, which includes the following steps: S1, initializing a sparse base matrix: Phi(beta)=A+Bdiag(beta); S2, updating the mean and variance of estimation signals based on the sparse base matrix, and updating and iterating the noise variance, signal variance and off-grid parameters based on the mean and variance of estimation signals; S3, judging whether the maximum value of the absolute values of the iterated and updated off-grid parameters is greater than a set threshold C, executing S4 if the maximum value is greater than the set threshold C, otherwise, executing S5; S4, migrating the grid according to the off-grid parameters and the threshold C, updating matrixes A and B, and updating the sparse base matrix; S5, judging whether convergence is achieved or the number of iterations reaches the upper limit, returning to S2 if convergence is not achieved and the number of iterations doesnot reach the upper limit, otherwise, ending iteration; and S6, using the updated sparse base matrix to update an estimation model of off-grid DOA to get an estimation result. Compared with the priorart, the method of the invention has the advantage of high accuracy.

Description

technical field [0001] The invention relates to an estimation method, in particular to a method for estimating the direction of arrival based on the off-grid sparse Bayesian. Background technique [0002] DOA estimation is a key technology for practical engineering applications such as target positioning, detection, and identification. It is widely used in military and national economic applications such as radar, communication, radio astronomy, geophysics, speech recognition, sonar, and medical imaging. Traditional DOA estimation (such as: MUSIC algorithm, l 1 -SVD method, etc.) are based on subspace algorithms, which often need to be estimated in an environment where the signal-to-noise ratio is high, the number of sampling snapshots is large, and the correlation between sources is not strong, and it is also necessary to know the source This limits the application occasions of DOA estimation. Moreover, when the signals are densely distributed in the airspace, the accurac...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G01S3/12
CPCG01S3/12
Inventor 魏爽顾旭陈俊飞彭张节张静李莉
Owner SHANGHAI NORMAL UNIVERSITY
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com