Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Ultrasonic suspension transmission distance compensating method based on excitation phase position

A technology of transmission distance and compensation method, which is applied in the direction of conveyors, conveyor objects, transportation and packaging, etc., which can solve the problems of complex adjustment process, difficult to ensure the accuracy of support length adjustment, and difficult to be equivalent to cross-section

Active Publication Date: 2020-07-24
HARBIN INST OF TECH
View PDF10 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

In order to realize traveling wave transmission at any distance, it is necessary to compensate the vibration elastic body support distance of the ultrasonic traveling wave suspension transmission device. Common compensation methods include mechanical compensation and excitation phase compensation. Mechanical compensation requires adjustment of the experimental device, and the adjustment process is complicated. , since the vibration elastic body and the transducer are connected by bolts, it is difficult to be equivalent to a section, and the adjustment accuracy of the actual support length is difficult to guarantee

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Ultrasonic suspension transmission distance compensating method based on excitation phase position
  • Ultrasonic suspension transmission distance compensating method based on excitation phase position
  • Ultrasonic suspension transmission distance compensating method based on excitation phase position

Examples

Experimental program
Comparison scheme
Effect test

specific Embodiment approach 1

[0031] Combine below Figure 1-6 To illustrate this embodiment, a method for compensating the transmission distance of ultrasonic levitation based on the excitation phase includes the following steps:

[0032] Step 1: Build an ultrasonic traveling wave suspension transmission device, apply two excitation signals with the same amplitude and frequency to the ultrasonic transducer I3-1 and the ultrasonic transducer II3-2 respectively, and there is a phase difference I between the two excitation signals;

[0033]Step 2: Analyze the vibration displacement of the vibrating elastic body 5, set the distance between the two support points of the vibrating elastic body 5 and the corresponding spatial phase difference is φ, set the signals of ultrasonic transducer Ⅰ3-1 and ultrasonic transducer Ⅱ3-2 The phase difference I in time is θ, and the relationship between the excitation signal phase difference IIθ and the spatial phase difference φ under the condition that the maximum vibration ...

specific Embodiment approach 2

[0037] Combine below Figure 1-6 Describe this embodiment, this embodiment will further explain Embodiment 1, the method for solving the relationship between the excitation signal phase difference IIθ and the spatial phase difference φ under the condition that the maximum vibration displacement and the minimum vibration displacement at any position on the vibrating elastic body 5 are equal is: At any time t, the vibration displacement equation f(x, t) of the particle located at x on the vibrating elastic body 5 along the z direction, from the vibration displacement equation f(x, t), the amplitude expression A(t ), looking for the extremum value of the amplitude on the vibrating elastic body 5, let Bring the solution conditions into the amplitude expression A(t) to find the maximum vibration displacement A max (θ, φ) and minimum vibration displacement A min (θ,φ), solve for A max (θ,φ)=A min (θ, φ), obtain the maximum vibration displacement at any position on the vibrating...

specific Embodiment approach 3

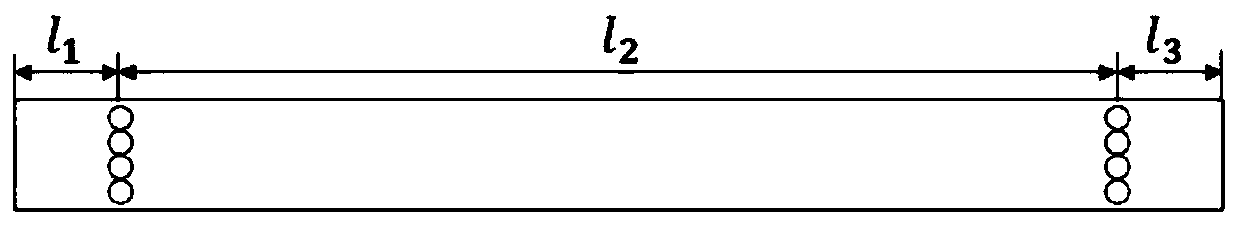
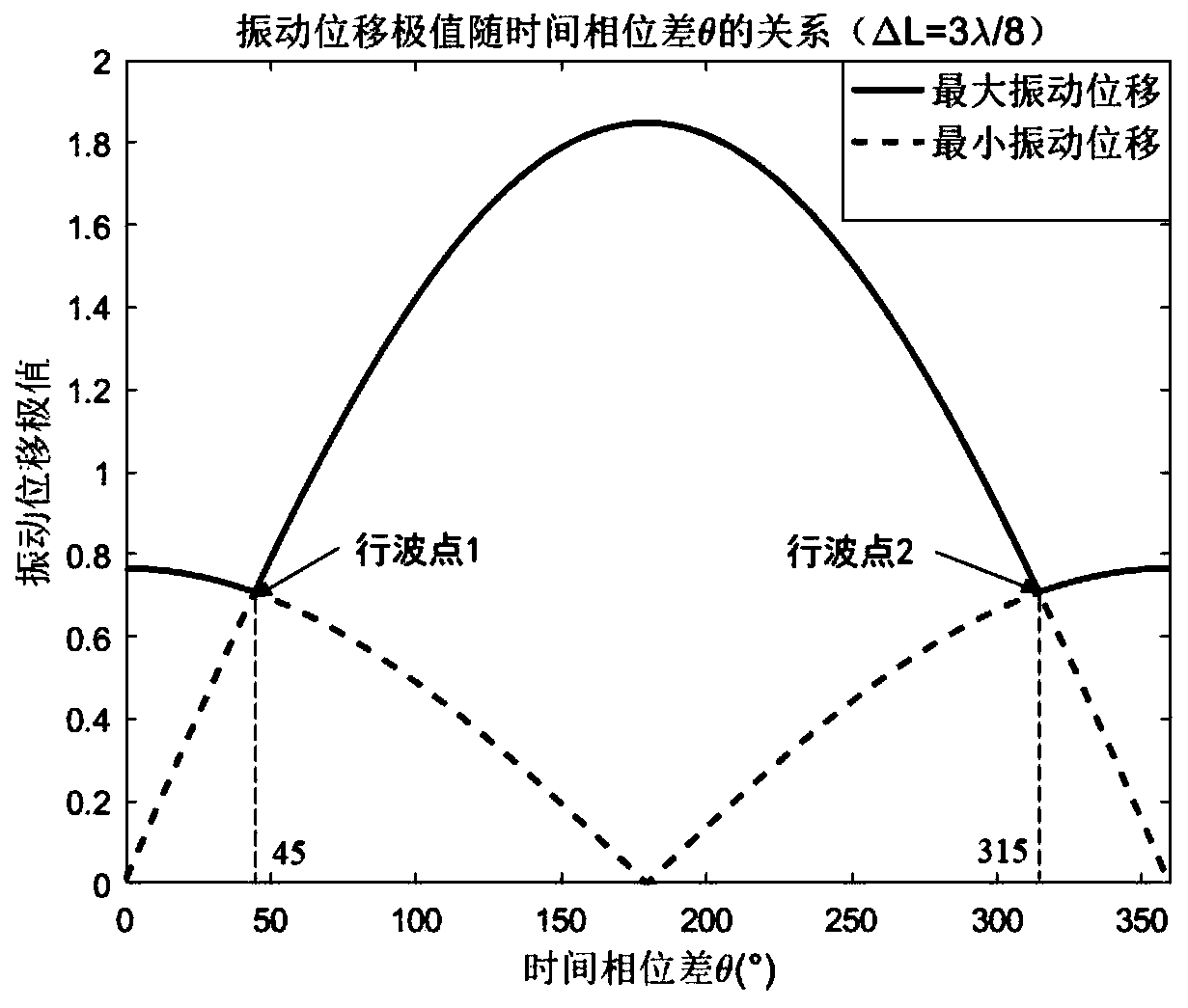
[0078] Combine below Figure 1-6 Describe this embodiment. This embodiment will further explain Embodiment 2. From the relational expression obtained in Step 2, find out the relationship between the time phase difference IIθ and the space phase difference φ in the traveling wave mode, and the vibrating elastic body 5 supports the distance l 2 =mλ+ΔL, Spatial phase φ = kl 2 =2mπ+Δφ, when ΔL figure 2 Shown l 2 is the supporting distance of the vibrating elastic body 5, l 1 and l 3is the shortest distance from the two supporting positions along the length of the vibrating elastic body 5 to the edge of the vibrating elastic body 5, no matter how the supporting distance L of the vibrating elastic body 5 changes, the spatial phase Δφ is compensated by the excitation phase θ, so that a pure traveling wave can be realized transmission. Drawing the change curve of the maximum vibration displacement of the vibrating elastic body 5 with the phase difference Ⅱθ can guide the experim...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention relates to the field of ultrasonic suspension transmission, in particular to an ultrasonic suspension transmission distance compensating method based on the excitation phase position. The design frequency of an ultrasonic traveling wave suspension transmission device can be set as the excitation frequency of an ultrasonic transducer I and an ultrasonic transducer II; the phase position difference I of excitation signals of the ultrasonic transducer I and the ultrasonic transducer II of the ultrasonic traveling wave suspension transmission device is defined as theta, the phase position difference I theta is constantly changed, a laser vibration measurer is utilized for conducting the vibration speed scanning test, and the corresponding phase position difference II theta when the vibration speed amplitudes of various points of a vibration elastic body are the same is found; and the time phase position difference II theta is used for determining the space phase position difference phi, after the space phase position difference phi is determined, the corresponding time phase position difference II theta when the maximum vibration displacement and the minimum vibration displacement are the same is found, the phase position difference I of the excitation signals is adjusted into the phase position difference I corresponding to the pure traveling wave point, a test device can generate a traveling wave sound field, and ultrasonic travelling wave suspension transmission is achieved.

Description

technical field [0001] The invention relates to the field of ultrasonic levitation transmission, in particular to a method for compensating the ultrasonic levitation transmission distance based on the excitation phase. Background technique [0002] Ultrasonic levitation is a nonlinear phenomenon of the sound field, and the object is suspended at the position of the sound pressure potential well by means of the sound radiation force in the high-intensity sound field. Ultrasonic levitation transmission is a non-contact transmission technology developed on the basis of ultrasonic levitation. Among them, the standing wave transmission actively adjusts the excitation parameters, changes the sound field distribution, and makes the position of the sound pressure potential well move in the direction of the sound field, so that the object at the suspended node moves, but the moving speed of the suspended object is relatively slow; however, the line The wave transmission is through t...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): B65G54/00B65G49/07B65G49/06
CPCB65G49/06B65G49/07B65G54/00
Inventor 赵杰穆冠宇孟繁斌董亚北董惠娟
Owner HARBIN INST OF TECH
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com