Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

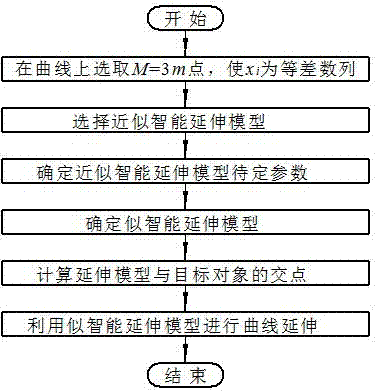
Method for non-circular curve trend extrapolation similarity intelligent extension

A trend extrapolation and curve technology, applied in the field of 3D modeling, can solve the problems of high labor intensity, low precision, low efficiency of drawing and modeling for designers, etc.

Inactive Publication Date: 2013-03-13
ANHUI UNIVERSITY OF TECHNOLOGY AND SCIENCE
View PDF2 Cites 1 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

At present, designers can only use spline curves to manually draw the extended part, the accuracy is very low, the efficiency of drawing and modeling is not high, and the labor intensity of designers is high

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Method for non-circular curve trend extrapolation similarity intelligent extension
  • Method for non-circular curve trend extrapolation similarity intelligent extension
  • Method for non-circular curve trend extrapolation similarity intelligent extension

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

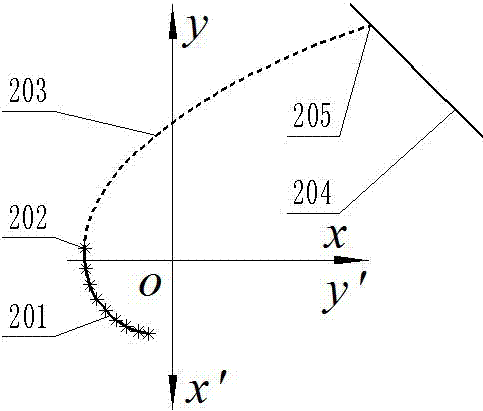
[0036] See attached figure 2 , the expression of curve 201 to be extended in this embodiment is , [-33.000, -7.027]; the target object 204 expression is ; The trend extrapolation approximate intelligent extension includes the following steps:

[0037] (1) Calculate the characteristic axis of the curve 201 and y Axis angle =-90°, establish a Cartesian affine coordinate system with the coordinate origin , select 9 points on the curve 201: (21.624, -7.027), (18.333, -17.442), (15.042, -22.116), (11.751, -25.383), (8.460, -27.842), (5.169, -29.732), (1.878, -31.17), (-1.413, -32.250), (-4.704, -33.000), the 9 points are in the coordinate system The middle abscissa is an arithmetic sequence;

[0038] (2) Judging the application conditions of the non-circular curve extension model: the above-mentioned selection of 9 point sequences second difference of The maximum difference is 7.840×10 -4 , the third order difference The maximum difference is 1.170×10 -2 , logar...

Embodiment 2

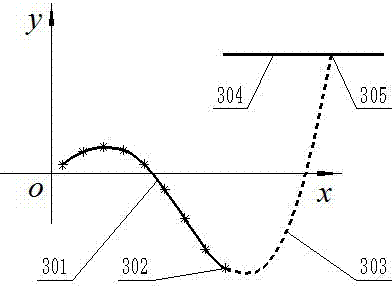
[0044] See attached image 3 , the expression of the non-circular curve 301 to be extended in this embodiment is , [1.000, 4.800]; target object 304 expression is ; The trend extrapolation approximate intelligent extension includes the following steps:

[0045] (1) Select 9 points on the non-circular curve 301 to be extended: (1.000, 0.842), (1.475, 1.468), (1.950, 1.812), (2.425, 1.593), (2.900, 0.694), (3.375, - 0.781), (3.850, -2.505), (4.325, -4.005), (4.800, -4.782), the abscissa of the 9 points is an arithmetic sequence;

[0046] (2) Judging the application conditions of the non-circular curve extension model: the above-mentioned selection of 9 point sequences second difference of The maximum difference is 0.001, the third order difference The maximum difference is 1.760×10 -5 , logarithmic first order difference The maximum difference is 8.214, logarithmic first order difference ratio of The maximum difference is 5.518, comparing the calculation resul...

Embodiment 3

[0052] See attached Figure 4 , the expression of the non-circular curve 401 to be extended in this embodiment is , [-0.198, 0.281]; target object 404 expression is ; The trend extrapolation approximate intelligent extension includes the following steps:

[0053] (1) Select 9 points on the non-circular curve 401 to be extended: (-0.198, 0.140), (-0.138, 0.149), (-0.078, 0.164), (-0.018, 0.184), (0.042, 0.209), (0.102, 0.238), (0.162, 0.272), (0.221, 0.309), (0.281, 0.351), the abscissa of the 9 points is an arithmetic sequence;

[0054] (2) Judging the application conditions of the non-circular curve extension model: the above-mentioned selection of 9 point sequences second difference of The maximum difference is 1.003×10 -5 , the third order difference The maximum difference is 0.002, logarithmic first order difference The maximum difference is 7.096×10 -8 , logarithmic first order difference ratio of The maximum difference is 0.003, comparing the calculat...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention belongs to the field of three-dimensional (3D) modeling for computer graphics, in particular relates to polynomial surface graphics, and relates to a method for non-circular curve trend extrapolation similarity intelligent extension. For connection and transition modeling with low precision requirements, non-circular curves with arbitrary shapes are frequently extended to a designated target object. According to the property that characteristic points are selected from a non-circular curve to be extended, the non-circular curve which has a shape being the most similar to the non-circular curve to be extended is selected from four typical non-circular curves to be subjected to trend extrapolation intelligent extension, high-precision extension can be carried out within a neighborhood area adjacent to an extension starting point, and the practical requirements of most graphics and modeling with low precision requirements can be satisfied. The method comprises the following steps that: points M which equals to 3m are selected from the non-circular curve to be extended and form an arithmetic progression; a similar intelligent extension model is selected; the undetermined parameters of the similar intelligent extension model are determined; a non-circular curve extension model is determined; the intersection points of the extension model and the target object are calculated; and the non-circular curve extension model is utilized to extend.

Description

technical field [0001] The invention belongs to the field of 3D modeling for computer drawing, in particular to polynomial surface drawing, and relates to a non-circular curve trend extrapolation approximate intelligent extension method. Background technique [0002] Modern CAD software such as AutoCAD, CAXA, Pro / E, UG, CATIA, etc. all have the extend function, which can extend the line or arc to intersect the specified object, and keep the original characteristics (line or arc) unchanged; but these software extend cannot extend non-circular curves of arbitrary shape. [0003] Chinese patent CN 101482979A discloses a smooth and optimized CAD method for continuous curvature splicing of NURBS space curves, which fills the gap between two NURBS curves without changing the original part of the curve, and ensures that the extension of the curve Optimum smoothness; Chinese patent CN 101299278A discloses a CAD method based on extended product shape space curve splicing, which not ...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): G06T17/00
Inventor 刘有余杜俊俊随和
Owner ANHUI UNIVERSITY OF TECHNOLOGY AND SCIENCE
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com